
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
(1-b) Given the initial conditions ?(0) = 4, and ? (0) = 1, determine the particular solution of
?(?).
(1-c) Use Laplace transform to solve the given differential equation with the same initial conditions
given in (1-b).
(1-f) Assume now that the external force ?(?) equals 10 ?, repeat all parts (1-a,b,c,d,e).
just solve c and f u can see all question on pic
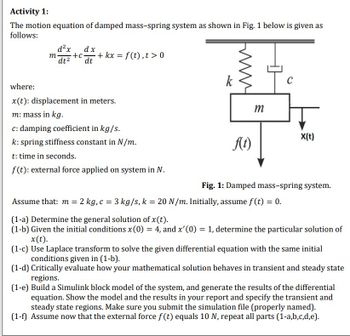
Transcribed Image Text:Activity 1:
The motion equation of damped mass-spring system as shown in Fig. 1 below is given as
follows:
m
d²x
dx
-+c+kx = f(t), t> 0
dt² dt
where:
x(t): displacement in meters.
m: mass in kg.
c: damping coefficient in kg/s.
k: spring stiffness constant in N/m.
t: time in seconds.
f(t): external force applied on system in N.
k
f(t)
m
C
Assume that: m = 2 kg, c = 3 kg/s, k = 20 N/m. Initially, assume f(t) = 0.
X(t)
Fig. 1: Damped mass-spring system.
(1-a) Determine the general solution of x (t).
(1-b) Given the initial conditions x (0) = 4, and x'(0) = 1, determine the particular solution of
x(t).
(1-c) Use Laplace transform to solve the given differential equation with the same initial
conditions given in (1-b).
(1-d) Critically evaluate how your mathematical solution behaves in transient and steady state
regions.
(1-e) Build a Simulink block model of the system, and generate the results of the differential
equation. Show the model and the results in your report and specify the transient and
steady state regions. Make sure you submit the simulation file (properly named).
(1-f) Assume now that the external force f(t) equals 10 N, repeat all parts (1-a,b,c,d,e).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 5 steps with 5 images

Knowledge Booster
Similar questions
- Obtain the differential equation by eliminating all arbitrary constants. 1.) y² = Ax² + B secxarrow_forwardTransform the differential equation into an equivalent system of first-order differential equations: x(3) = (x')? + cos xarrow_forwardSolve the differential equation using either the method of undetermined coefficients or Laplace transforms. y'''+y"+y'+y=e-t+4tarrow_forward
- 3arrow_forwardConsider the differential equation: dy/dx = y(3 - y) 1) Use the slope field plotter to plot different solutions corresponding to A: (0,-2); B: (0,1); C: (0,3); D: (0,4). You can drag each point to the correct place if they're somewhere else on the plane, or click on the point and redefine. Capture a screenshot showing all the solution curves and insert below. f'(x)=y(3-y) Kin/5 -5 Density Length dy de -8 Step size 0.1 Ymas y (3-9) Input. ✔Solution A Solution B Solution C Solution D DCQ= AC: 2) To what value does y(x) approach, as x → ∞, for the solutions corresponding to B,C, and D? To what value does y(x) approach, as x →∞, for the solution corresponding to A? Why is it different from the others? Be specific and answer in terms of what you know about the slope field and its effect on the solution. - 3) Use wolframalpha.com or something similar to find the particular solution corresponding to each of the points A,B,C,D. How is the solution through Point C different from the other…arrow_forwardConsider the differential equation following is the slope of a line-segment 3 -1 0 07 - 2y = 3x. If sketching a slope field, which one of the at the point (x, y) = (1, 2)?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

