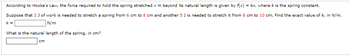
According to Hooke's Law, the force required to hold the spring stretched x m beyond its natural length is given by f(x) = kx, where k is the spring constant. Suppose that 3 J of work is needed to stretch a spring from 6 cm to 8 cm and another 5 J is needed to stretch it from 8 cm to 10 cm. Find the exact value of k, in N/m. k= N/m What is the natural length of the spring, in cm? cm

A spring with a 2-kg mass and a damping constant 2 can be held stretched 2.5 meters beyond its natural length by a force of 7.5 newtons. Suppose the spring is stretched 5 meters beyond its natural length and then released with zero velocity, In the notation of the text, what is the value c² 4mk? 2 m²kg²/sec² Find the position of the mass, in meters, after t seconds. Your answer should be a function of the variable t with the general form cos(ßt) + c₂ert sin(8t) eat C1 α= ß = Y = 8 = - C1 = C₂ =

A mass weighing 16 pounds stretches a spring 8 feet. The mass is initially released from rest from a point 4 feet below the equilibrium position, and the subsequent motion takes place in a medium that offers a 1 damping force that is numerically equal to - the instantaneous velocity. Find the equation of motion x(t) if the mass is driven by an external force equal to f(t) = 20 cos(3t). (Use g = 32 ft/s2 for the acceleration 2 due to gravity.) x(t) = ft

According to Newton's law of universal gravitation, the force F between two bodies of constant mass m and M is GmM given by the formula F = d? where G is the gravitational constant and d is the distance between the bodies. a. Suppose that are constants. Find the rate of change of force F with respect to distance d. F'(d) = Preview syntax error Enter an algebraic expression [more.] b. Find the rate of change of force F with gravitational constant G = 6.67 x 10-1 Nm²/kg², on two bodies 25 meters apart, each with a mass of 1000 kilograms. Answer in scientific notation, rounding to 2 decimal places. * N/m Preview