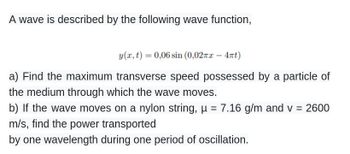
A wave is described by the following wave function, y(x, t) = 0,06 sin (0,027x - 4t) a) Find the maximum transverse speed possessed by a particle of the medium through which the wave moves. b) If the wave moves on a nylon string, μ = 7.16 g/m and v = 2600 m/s, find the power transported by one wavelength during one period of oscillation.

A wave in a string obeys the equation y(x,t) = (5.0cm) sin [(13.8 rad/m)x + (2070 rad/s)t + 2.19rad]. Find the velocity of the wave propagation

For the following statements, identify which are True and which are False. If False, please explain

A string is attached – vertically – from one end to a vibrator and from the other end to a block of mass m = 1.8 kg. The power delivered to produce a wave on this string is P = 12 W. Assume that the block is replaced by a lighter one with a mass m' = 0.2 kg. Determine the new power, P', required to generate a wave with the same amplitude and frequency. 2 W О зw 4 W 5 W O 6 W

The wave vectok (k) of a travelling wave propagating along +xy plane is given below. If the magnitude of k is 5rad/m, angular frequency of the wave is 60rad/s, amplitude is 6 unit and value of the wave at origin is zero (u(x:0,y:0; t:0)=0) write a mathematical expression for this wave. ky K 30⁰ 0 |k|=5rad/m kx

Two children stretch a jump rope between them and send wave pulses back and forth on it. The rope is 4.9 m long, its mass is 0.58 kg, and the force exerted on it by the children is 53 N. (a) What is the linear mass density of the rope (in kg/m)?  kg/m (b) What is the speed of the waves on the rope (in m/s)?  m/s

A transverse wave on a string is described by the wave function y = 0.14 sin [() + 5nt] What is the speed of propagation of this wave? Answer:

A wave travels at speed of 60 cm/s on a string. Its wave is described with the function: y = A sin(kx – wt + p). Here x and y are in cm. A snapshot of the wave is shown below. a) Find the phase difference between x1 = 4 cm and x2 = 10 cm at the time when the snapshot was taken. b) Find the wave function in the form of, y = A sin(kx – wt + p), if the snapshot was taken at t = 0.01 s y(cm) 4 3 1 x(cm) 20 -1 6. 8 10 12 14 16 18 -3 -4 -5

A simple particle in a transverse traveling wave undergoes simple harmonic motion given by y(t)=(4.20cm)cos(2.10t). The wave travels to the left with a speed of 1.30cm/s Part a) Write an equation for the traveling wave, (x,t) assuming y(0,0)=0 Part b) Find the transverse velocity as a function of time for a particle located at 3.10cm Part c) Find the transverse velocity as a function of time for a particle located at 5.10cm

A wave is modeled with the wave function y(x,t) = 0.75m sinf 85cm'x+ 7.75s't + n/121 Calculate the following: a) Time period b) Frequency O Wavelength d) Wave speed e) The direction of propagation of the wave modeled by the given wave function?