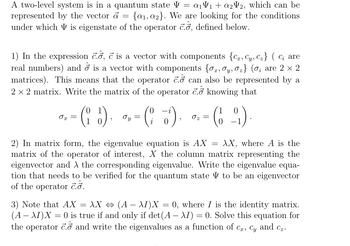
A two-level system is in a quantum state = α₁₁ + a22, which can be represented by the vector a = {a1, a2}. We are looking for the conditions under which is eigenstate of the operator c., defined below. 1) In the expression c.ỗ, c is a vector with components {Cr, Cy, Cz} ( C; are real numbers) and ỗ is a vector with components {σx, σy,σz} (σ¿ are 2 × 2 matrices). This means that the operator c. can also be represented by a 2 × 2 matrix. Write the matrix of the operator c. knowing that 0 0x = = (₁ }) = ( 5 ) . Oy σz= 0 (6-99) 1 0 1 0 2) In matrix form, the eigenvalue equation is AX AX, where A is the matrix of the operator of interest, X the column matrix representing the eigenvector and the corresponding eigenvalue. Write the eigenvalue equa- tion that needs to be verified for the quantum state to be an eigenvector of the operator c.. = 3) Note that AX = XX ⇒ (A - I)X 0, where I is the identity matrix. (AAI)X=0 is true if and only if det(A - I) = 0. Solve this equation for the operator c. and write the eigenvalues as a function of Cx, Cy and Cz.

[p* L₂ da 0 HO H + →

For the system described in Exercise E7C.8(a), evaluate the expectation value of the angular momentum represented by the operator(ħ/i)d/dϕ for the case ml = +1, and then for the general case of integer ml.

P7B.1 Imagine a particle confined to move on the circumference of a circle ('a particle on a ring'), such that its position can be described by an angle & in the range 0 to 2π. Find the normalizing factor for the wavefunctions: (a) e" and (b) eim, where m, is an integer.

P7F.9 In this problem you will establish the commutation relations, given in eqn 7E. 14, between the operators for the x-, y-, and z-components of angular momentum, which are defined in eqn 7F.13. In order to manipulate the operators correctly it is helpful to imagine that they are acting on some arbitrary function f: it does not matter what fis, and at the end of the proof it is simply removed. Consider [,,1,] = ,-11. Consider the effect of the first term on some arbitrary function fand evaluate A D -x dx se The next step is to multiply out the parentheses, and in doing so care needs to be taken over the order of operations. (b) Repeat the procedure for the other term in the commutator, 1,1, f. (c) Combine the results from (a) and (b) so as to evaluate l f-11f;you should find that many of the terms cancel. Confirm that the final expression you have is indeed iħl_f, where l̟ is given in eqn 7F.13. (d) The definitions in eqn 7E.13 are related to one another by

Consider the three spherical harmonics (a) Y0,0, (b) Y2,–1, and (c) Y3,+3. (a) For each spherical harmonic, substitute the explicit form of the function taken from Table 7F.1 into the left-hand side of eqn 7F.8 (the Schrödinger equation for a particle on a sphere) and confirm that the function is a solution of the equation; give the corresponding eigenvalue (the energy) and show that it agrees with eqn 7F.10. (b) Likewise, show that each spherical harmonic is an eigenfunction of lˆz = (ℏ/i)(d/dϕ) and give the eigenvalue in each case.

Consider a particle of mass m confined to a one-dimensional box of length L and in a state with normalized wavefunction ψn. (a) Without evaluating any integrals, explain why ⟨x⟩ = L/2. (b) Without evaluating any integrals, explain why ⟨px⟩ = 0. (c) Derive an expression for ⟨x2⟩ (the necessary integrals will be found in the Resource section). (d) For a particle in a box the energy is given by En = n2h2/8mL2 and, because the potential energy is zero, all of this energy is kinetic. Use this observation and, without evaluating any integrals, explain why <p2x> = n2h2/4L2.

For a particle in a rectangular box with sides of length L1 = L and L2 = 2L, find a state that is degenerate with the state n1 = n2 = 2.

Evaluate Δx = (⟨x2⟩ − ⟨x⟩2)1/2 and Δpx = (⟨px2⟩ − ⟨px⟩2)1/2 for the ground state of (a) a particle in a box of length L and (b) a harmonic oscillator. Discuss these quantities with reference to the uncertainty principle.

Imagine a particle free to move in the x direction. Which of the following wavefunctions would be acceptable for such a particle? In each case, give your reasons for accepting or rejecting each function. (1) Þ(x) = x²; (iv) y(x) = x 5. (ii) ¥(x) = ; (v) (x) = e-* ; (iii) µ(x) = e-x²; (vi) p(x) = sinx