Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Related questions
Concept explainers
Question
1
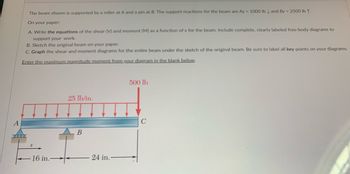
Transcribed Image Text:### Beam Analysis Problem for Educational Purposes
#### Problem Statement:
The beam shown is supported by a roller at point A and a pin at point B. The support reactions for the beam are \( A_y = 1000 \text{ lb} \downarrow \) and \( B_y = 2500 \text{ lb} \uparrow \).
#### Tasks:
- **A.** Write the equations for the shear force (\( V \)) and bending moment (\( M \)) as functions of \( x \) for the beam. Include complete, clearly labeled free-body diagrams to support your work.
- **B.** Sketch the original beam on your paper.
- **C.** Graph the shear force and bending moment diagrams for the entire beam under the sketch of the original beam. Be sure to label all key points on your diagrams.
#### Beam Configuration:
- The entire length of the beam from A to C is subjected to a uniformly distributed load of \( 25 \text{ lb/in} \).
- There is also a concentrated load of \( 500 \text{ lb} \) acting downward at point C.
- The distance between points A and B is \( 16 \text{ in} \).
- The distance between points B and C is \( 24 \text{ in} \).
#### Diagram Description:
1. **Support at Point A:**
- Represented as a roller support.
- Located at the left end of the beam.
2. **Support at Point B:**
- Represented as a pin support.
- Located \( 16 \text{ in} \) to the right of point A.
3. **Distributed Load:**
- Spans the entire length of the beam from A to C.
- Distributed load of \( 25 \text{ lb/in} \).
4. **Concentrated Load:**
- Applied at point C.
- Magnitude: \( 500 \text{ lb} \) downward.
#### Instructions for Students:
1. **Shear and Moment Equations:**
- Write the shear force (\( V \)) and bending moment (\( M \)) equations as functions of \( x \).
- Use free-body diagrams to explain the steps.
2. **Beam Sketch:**
- Draw the original beam with all applied loads and supports labeled.
3. **Shear and Moment Diagrams:**
![### Educational Content: Moments of Inertia for Various Shapes
#### Fundamental Equations
- **Maximum Friction Force:**
\[
F_{\text{max}} = \mu_s N
\]
where \(\mu_s\) is the coefficient of static friction and \(N\) is the normal force.
- **Kinetic Friction Force:**
\[
F_k = \mu_k N
\]
where \(\mu_k\) is the coefficient of kinetic friction.
- **Angle of Friction:**
\[
\tan \Phi = \mu
\]
#### Parallel Axis Theorem
\[
I = \bar{I} + Ad^2
\]
where \(\bar{I}\) is the moment of inertia about the centroidal axis, \(A\) is the area of the shape, and \(d\) is the distance between the centroidal axis and the axis about which the moment of inertia is being calculated.
#### Centroid Formulas
- **\( \bar{X} \):**
\[
\bar{X} = \frac{\sum Ax}{\sum A}
\]
- **\( \bar{Y} \):**
\[
\bar{Y} = \frac{\sum Ay}{\sum A}
\]
### Moments of Inertia for Common Shapes
#### Rectangular Area
- Diagram: A rectangle with base \(b\) and height \(h\). The centroid \(C\) is at the center.
- **Centroid Coordinates:**
\[
\bar{x} = \frac{b}{2}, \quad \bar{y} = \frac{h}{2}
\]
- **Area Moments of Inertia:**
\[
I_x = \frac{bh^3}{3}, \quad \bar{I}_x = \frac{bh^3}{12}, \quad I_z = \frac{bh}{12}(b^2 + h^2)
\]
#### Triangular Area
- Diagram: A right triangle with base \(b\) and height \(h\). The centroid \(C\) is at \(\frac{1}{3}\) along the base and height.
- **Centroid Coordinates:**
\[
\bar{x} = \frac{a + b}{](https://content.bartleby.com/qna-images/question/2f3435e6-2af6-4060-8093-d10f07d52056/4db91a3d-ca89-4a17-a85a-b272aa1b7481/mln871o_processed.jpeg)
Transcribed Image Text:### Educational Content: Moments of Inertia for Various Shapes
#### Fundamental Equations
- **Maximum Friction Force:**
\[
F_{\text{max}} = \mu_s N
\]
where \(\mu_s\) is the coefficient of static friction and \(N\) is the normal force.
- **Kinetic Friction Force:**
\[
F_k = \mu_k N
\]
where \(\mu_k\) is the coefficient of kinetic friction.
- **Angle of Friction:**
\[
\tan \Phi = \mu
\]
#### Parallel Axis Theorem
\[
I = \bar{I} + Ad^2
\]
where \(\bar{I}\) is the moment of inertia about the centroidal axis, \(A\) is the area of the shape, and \(d\) is the distance between the centroidal axis and the axis about which the moment of inertia is being calculated.
#### Centroid Formulas
- **\( \bar{X} \):**
\[
\bar{X} = \frac{\sum Ax}{\sum A}
\]
- **\( \bar{Y} \):**
\[
\bar{Y} = \frac{\sum Ay}{\sum A}
\]
### Moments of Inertia for Common Shapes
#### Rectangular Area
- Diagram: A rectangle with base \(b\) and height \(h\). The centroid \(C\) is at the center.
- **Centroid Coordinates:**
\[
\bar{x} = \frac{b}{2}, \quad \bar{y} = \frac{h}{2}
\]
- **Area Moments of Inertia:**
\[
I_x = \frac{bh^3}{3}, \quad \bar{I}_x = \frac{bh^3}{12}, \quad I_z = \frac{bh}{12}(b^2 + h^2)
\]
#### Triangular Area
- Diagram: A right triangle with base \(b\) and height \(h\). The centroid \(C\) is at \(\frac{1}{3}\) along the base and height.
- **Centroid Coordinates:**
\[
\bar{x} = \frac{a + b}{
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 5 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Question 6 a) The bore of each cylinder in a six-cylinder car engine is 45 mm and the stroke for each piston is 125 mm. calculate the cubic capacity of the engine in cubic centimetres (cm³); and calculate the clearance volume per cylinder for a compression ratio, r, of 8.75. b) The bore of each cylinder in a four-cylinder four-stroke internal combustion engine is 38 mm and the stroke of each piston is 165 mm. During testing, the engine runs at 180 revolutions per minute (rpm) with a pressure-volume indicator diagram showing a mean net area of 3.4 cm² and a diagram length of 1.2 cm. The pressure scale on the indictor diagram is set to 150 kN/m² per cm. Calculate the mean effective pressure (m.e.p) and the indicated power developed by this four-cylinder four-stroke engine. c) Figure Q6c shows a pulley system with a suspended load w of 2000 N. Calculate the following: (i) (ii) (iii) (iv) The effort force (FEffort) necessary to lift and hold a load of 2000 N. The ideal mechanical advantage…arrow_forward10 Figure 5arrow_forwardanswer this; The numerical value of ? can be calculated as mmarrow_forward
Recommended textbooks for you
- Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education 
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY