Question
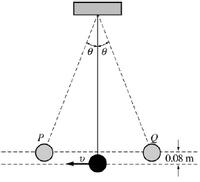
A simple pendulum consists of a bob of mass of 0.85 kg attached to a string of length 1.5 m. The pendulum is raised to point Q, which is 0.08 m above its lowest position, and released so that it oscillates with a small amplitude θ between the points P and Q as shown below.
- Draw free-body diagrams showing and labeling the forces acting on the bob in each situation.
- When it is at point P.
- When it is at the lowest point.
- Calculate the speed of the bob at the lowest point.
- Calculate the total energy of the oscillating system.
- Determine the height at which the potential and kinetic energies are equal.
- Describe one modification that can be made to double the period of the oscillation. Explain.
Transcribed Image Text:eTe
0.08 m
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 7 steps with 1 images
