Introductory Circuit Analysis (13th Edition)
13th Edition
ISBN: 9780133923605
Author: Robert L. Boylestad
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Related questions
Question
thumb_up100%
![**Question:**
A sensor needs a bias voltage of +3.000 volts. The DAC producing the voltage is 12 bits with a 3.3 volt reference. What is the DAC input code?
- ○ 1241
- ○ 3724
- ○ 3103
- ○ 3541
**Explanation:**
To find the correct DAC input code, use the formula for a Digital-to-Analog Converter (DAC):
\[ \text{Output Voltage} = \left(\frac{\text{Digital Code}}{2^n - 1}\right) \times \text{Reference Voltage} \]
Where:
- \( n \) is the number of bits (12 bits in this case).
- Digital Code is what we are trying to find.
- Reference Voltage is 3.3 volts.
Rearranging for the Digital Code gives:
\[ \text{Digital Code} = \left(\frac{\text{Output Voltage} \times (2^n - 1)}{\text{Reference Voltage}}\right) \]
Plug in the values:
\[ \text{Digital Code} = \left(\frac{3.000 \times (2^{12} - 1)}{3.3}\right) \]
Calculate:
\[ 2^{12} - 1 = 4095 \]
\[ \text{Digital Code} = \left(\frac{3.000 \times 4095}{3.3}\right) \]
\[ \text{Digital Code} \approx 3724 \]
Therefore, the correct answer is:
- ○ 3724](https://content.bartleby.com/qna-images/question/78790db4-d72e-4785-a32a-a8a933d5b327/ab887157-c975-4f89-8720-ca4dad15af3f/w1s42g_processed.png)
Transcribed Image Text:**Question:**
A sensor needs a bias voltage of +3.000 volts. The DAC producing the voltage is 12 bits with a 3.3 volt reference. What is the DAC input code?
- ○ 1241
- ○ 3724
- ○ 3103
- ○ 3541
**Explanation:**
To find the correct DAC input code, use the formula for a Digital-to-Analog Converter (DAC):
\[ \text{Output Voltage} = \left(\frac{\text{Digital Code}}{2^n - 1}\right) \times \text{Reference Voltage} \]
Where:
- \( n \) is the number of bits (12 bits in this case).
- Digital Code is what we are trying to find.
- Reference Voltage is 3.3 volts.
Rearranging for the Digital Code gives:
\[ \text{Digital Code} = \left(\frac{\text{Output Voltage} \times (2^n - 1)}{\text{Reference Voltage}}\right) \]
Plug in the values:
\[ \text{Digital Code} = \left(\frac{3.000 \times (2^{12} - 1)}{3.3}\right) \]
Calculate:
\[ 2^{12} - 1 = 4095 \]
\[ \text{Digital Code} = \left(\frac{3.000 \times 4095}{3.3}\right) \]
\[ \text{Digital Code} \approx 3724 \]
Therefore, the correct answer is:
- ○ 3724
Expert Solution
arrow_forward
Step 1
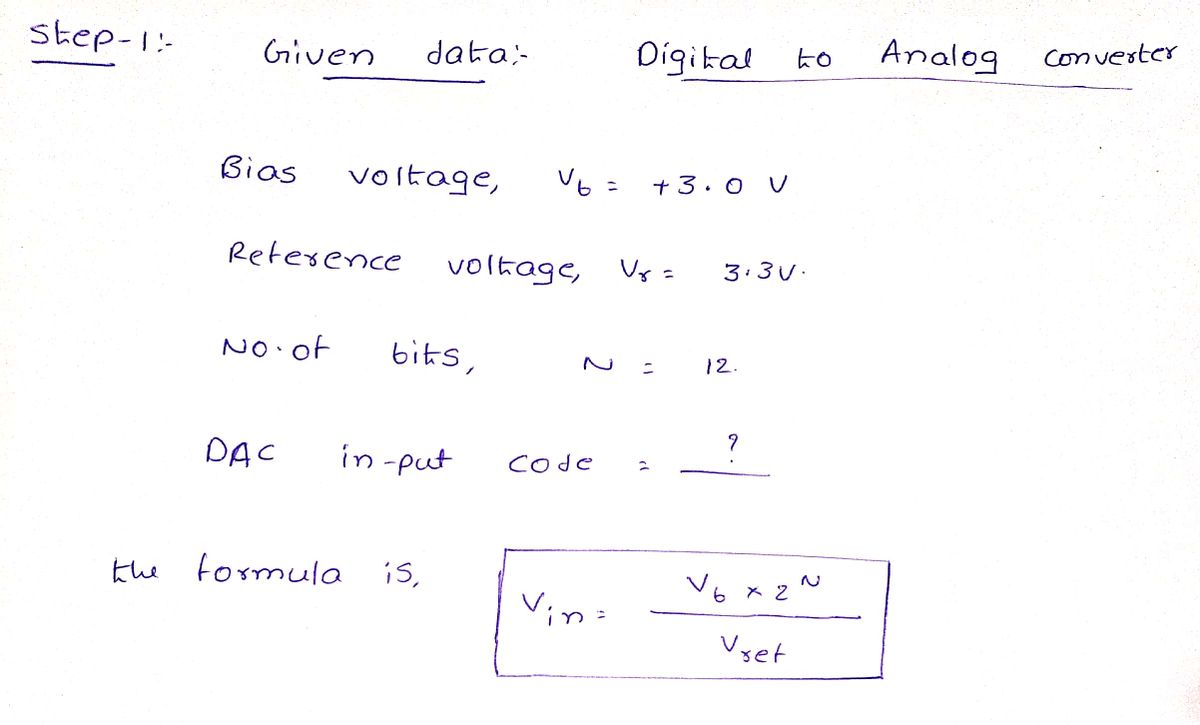
Step by stepSolved in 2 steps with 2 images
Knowledge Booster
Similar questions
- Please in typing format please ASAP for Thanks ?arrow_forwardIf the frequency of the input signal applied to a full wave rectifier is 120 Hz, then what will be the frequency of the output signal?arrow_forwardQuestion 13 The output frequency of a full-wave rectifier is twice the input frequency. True O Falsearrow_forward
- 2) approximately draw Vout for the circuits below, separately. Point out which one is a rectifier which one is a clipping circuit. Use Vp = 0.7V. Input signal 2tVin 1.5 1 0.5 -0.5 -1 -1.5 -2 Circuit (a) Circuit (b) Vin Vout Vin Vaut R 2 Vonut 1.5 1.5 1 1 0.5 0.5 1 3 4 7 1 2 3 6 7 -0.5 -0.5 -1 -1 -1.5 -1.5 -2, -2arrow_forwardFor a half wave or full wave rectifier the Peak Inverse Voltage of the rectifier is always greater than the input voltage. Select one: True O Falsearrow_forwardVI Ru Ri Vz I, Io R2 = 3837.79 O. R3 = 3989.3 Q. %3D R4 = 2898.06 Q. V1 = 4.8 V. V2 = 8.1 V. 11 = 0.0099 A Determine the following: a.) The output current lo %3Darrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Introductory Circuit Analysis (13th Edition)Electrical EngineeringISBN:9780133923605Author:Robert L. BoylestadPublisher:PEARSON
Delmar's Standard Textbook Of ElectricityElectrical EngineeringISBN:9781337900348Author:Stephen L. HermanPublisher:Cengage Learning
Programmable Logic ControllersElectrical EngineeringISBN:9780073373843Author:Frank D. PetruzellaPublisher:McGraw-Hill Education 
Fundamentals of Electric CircuitsElectrical EngineeringISBN:9780078028229Author:Charles K Alexander, Matthew SadikuPublisher:McGraw-Hill Education
Electric Circuits. (11th Edition)Electrical EngineeringISBN:9780134746968Author:James W. Nilsson, Susan RiedelPublisher:PEARSON
Engineering ElectromagneticsElectrical EngineeringISBN:9780078028151Author:Hayt, William H. (william Hart), Jr, BUCK, John A.Publisher:Mcgraw-hill Education,
Introductory Circuit Analysis (13th Edition)
Electrical Engineering
ISBN:9780133923605
Author:Robert L. Boylestad
Publisher:PEARSON
Delmar's Standard Textbook Of Electricity
Electrical Engineering
ISBN:9781337900348
Author:Stephen L. Herman
Publisher:Cengage Learning
Programmable Logic Controllers
Electrical Engineering
ISBN:9780073373843
Author:Frank D. Petruzella
Publisher:McGraw-Hill Education
Fundamentals of Electric Circuits
Electrical Engineering
ISBN:9780078028229
Author:Charles K Alexander, Matthew Sadiku
Publisher:McGraw-Hill Education
Electric Circuits. (11th Edition)
Electrical Engineering
ISBN:9780134746968
Author:James W. Nilsson, Susan Riedel
Publisher:PEARSON
Engineering Electromagnetics
Electrical Engineering
ISBN:9780078028151
Author:Hayt, William H. (william Hart), Jr, BUCK, John A.
Publisher:Mcgraw-hill Education,