Question
In the circuit shown in Fig. , switch S1 has been
closed for a long enough time so that the current reads a steady 3.50 A.
Suddenly, switch S2 is closed and S1 is opened at the same instant.
(a) What is the maximum charge that the capacitor will receive?
(b) What is the current in the inductor at this time?
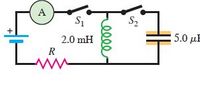
Transcribed Image Text:A
2.0 mH
5.0 µE
R
elee
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 1 images

Knowledge Booster
Similar questions
- In the RL circuit shown below, it takes 1.5 seconds after the switch is closed for the current to reach half of its steady-state value. If the inductor was replaced with an inductor with twice the inductance and the experiment was run again from the beginning, how long would it take for the current to reach half of its steady-state value?arrow_forwardA loop of wire has a self-inductance of 5.5 mH. You pass a current of 9.0 Amps though the loop, and then drop the current to 0 at a contastant rate over 3.5 seconds. What is the EMF generated?arrow_forwardA 10 -mH inductor and a 25 MF (micro F) capacitor are connected in parallel. The combination is in series with a resistor and a 15 Ohm resistor and 10V, 160Hz emf. (a) What is the current through the resistor? (b) What is the phase angle?arrow_forward
- A 12.0 V battery is connected into a series circuit containing a 20.0 resistor and a 1.50 H inductor. (a) In what time interval (in s) will the current reach 50.0% of its final value? S (b) In what time interval (in s) will the current reach 90.0% of its final value? S What If? After a very long time, using a switch like that shown in the figure below, the battery is removed and the inductor is connected directly across the resistor. R Są ob ก ε ele (c) In what time interval (in s) will the current decrease to 50.0% of its initial value? S (d) In what time interval (in s) will the current decrease to 10.0% of its initial value? Sarrow_forwardA circuit consists of a 20 nF capacitor in series with a 20 µH inductor. If a charge of 30 nC is put on the capacitor, there is an oscillation. (a) What is the maximum current that moves through the circuit? (b) Find the maximum energy in the inductor and the in the capacitor? (c) What is the angular frequency of oscillation? What is the period? (d) Assume the trace resistance in the circuit is 0.102. Describe quantitatively and qualitatively the oscillation in the circuit.arrow_forwardYou are given an LC oscillator, with an L = 3.3 mH and a C = 0.09 mF. Initially, the inductor has a current flowing through it of 24 mA and the charge on the capacitor is zero at this same time. Find the maximum charge that we can expect on the capacitor at some later time. Assume that there are no internal resistance losses for these oscillations.arrow_forward
- In the circuit shown in the Figure with 3.0 mH for the inductance and 6.0 uF for the capacitance, S1 has been closed for a long enough time so that the current reads a steady 3.50 A. Suddenly, S2 is closed and S1 is opened at the same instant. What is the maximum charge that the capacitor will receive? What is the current in the inductor at this time? At an instant when the charge on the capacitor is 0.1 mC, what is the current in the inductor? At an instant when the charge on the capacitor is 0.1 mC, what is the rate at which the current in the inductor is changing?arrow_forward(L11) At t = 0 s, a 3.53E-3 H inductor carries a 5.03 A current flowing from a to b, as shown in the figure. The current then decreases at a constant rate until it becomes zero at t = 4.10 s. The voltage is measured to be Va at point a and V₁ at point b. What is the voltage difference Va-Vb between t = 0s and t = 4.10 s (in V; answer the sign and magnitude)? a فیقققق L elle I barrow_forwardA(n) 37.0 µF capacitor is charged to 4.7 µC and then an inductor of 9 mH is connected across the terminals of the charged capacitor. Find the maximum current that will flow in this circuit assuming there are no losses due to internal resistance.arrow_forward
arrow_back_ios
arrow_forward_ios