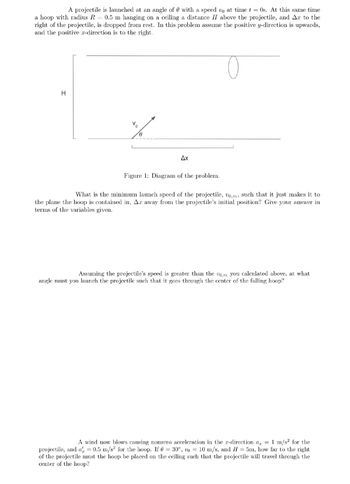
A projectile is launched at an angle of θ with a speed v0 at time t = 0s. At this same time
a hoop with radius R = 0.5 m hanging on a ceiling a distance H above the projectile, and ∆x to the
right of the projectile, is dropped from rest. In this problem assume the positive y-direction is upwards,
and the positive x-direction is to the right. (Diagram attached in image)
A) What is the minimum launch speed of the projectile, v0,m, such that it just makes it to
the plane the hoop is contained in, ∆x away from the projectile’s initial position? Give your answer in
terms of the variables given.
B) Assuming the projectile’s speed is greater than the v0,m you calculated above, at what
angle must you launch the projectile such that it goes through the center of the falling hoop?
C) A wind now blows causing nonzero acceleration in the x-direction ax = 1 m/s^2 for the
projectile, and a′x = 0.5 m/s^2 for the hoop. If θ = 30◦, v0 = 10 m/s, and H = 5m, how far to the right
of the projectile must the hoop be placed on the ceiling such that the projectile will travel through the
center of the hoop?
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 4 images

- The same projectile experiment was conducted as you did in class. A racquet ball rolls down a track, leaves the track at an initial height of 0.961m above floor, with an exit speed Vexit 1.40m/s at an angle exit 7.46°. The landing spot was repeatedly measured for ten times, and the racquet ball's horizontal range data is provided below. Data run = Landing spot (m) 1 2 0.585 0.590 3 4 0.595 0.590 5 6 0.585 0.580 7 0.580 8 = 9 10 0.585 0.580 0.575arrow_forwardlooking for A and Barrow_forwardA projectile is fired with initial speed 150 m/s and an angle of elevation 45° from a position 10 m above ground level. Where does the projectile hit the ground and with what speed? Solution If we place the origin at ground level, then the initial position of the projectile is (0, 10) and so we need to adjust the parametric equations of the trajectory by adding 10 to the expression for y. With v0 = 150 m/s, ? = 45°, and g = 9.8 m/s2, we have x = 150 cos ? 4 t = y = 10 + 150 sin ? 4 t − 1 2 (9.8)t2 = . Impact occurs when y = 0, that is 4.9t2 − 75 2 t − 10 = 0. Solving this quadratic equation (and using only the positive value of t), we get the following. (Round your answer to two decimal places.) t = 75 2 + 11,250 + 196 9.8 ≈ Then x ≈ 75 2 (21.74) ≈ (rounded to the nearest whole number), so the projectile hits the ground about…arrow_forward
- This problem will involve deriving a formula or two for a projectile launched from one height and angle and landing at a different height on Earth. Begin with a projectile launched at angle 0 above horizontal from a height y₁ with initial velocity Vo. The projectile lands at a point with height y₂. These are the given quantities: vo, 0, y₁, y2 and g. Construct formulae for each of the following, as. a function of given quantities the horizontal distance traveled. the maximum height reached. the time taken. the angle of impact. (find the final velocity components first).arrow_forwardmake it clear and answerr visible thanksarrow_forwardYoure in an airplane that flies horizontally with speed 940km/h (260m/s) when the engine falls off. Neglecting air resisitence, assume it takes 27 s for the engine to hit the ground. Part A: Find the height of the airplane. Part B:Find the horizontal distance that the aircraft engine falls Part C: If the airplane somehow continues to fly as if nothing had happened, what is the distance between the engine and the airplane at the moment the engine hits the ground?arrow_forward