Question
- a physics student start sailing away in a boat at 5.0 m/s as shown in the diagram. When they are 50.0 m from the shore, Mr Berthiaume spots them, and launches a copy of the exam towards the boat so they will have no choice but to complete it. Using every quantum of strength in his body, he throws the exam with a speed of 40.0 m/s, while summoning physics to remove air resistance for the moment. The exam lands on the boat after the boat has moved another 50.0 m.
(a) At what angle above the horizontal must Mr Berthiaume throw the exam so it lands on the boat?
(b) How high is the cliff?
(c) With what velocity does the exam hit the boat?
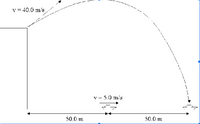
Transcribed Image Text:v = 40.0 m/s
v - 5.0 m's
50.0 m
50.0 m
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 5 steps

Knowledge Booster
Similar questions
- The muon is an unstable subatomic particle witha mean lifetime of 2.2 μs, and about 10,000 muons reach everysquare meter of the earth's surface a minute. A muon has a linear momentum G, and after some short time it decays into two other elementary particles with masses m1 and m2. The energy Q released during the decay is converted entirely into the kinetic energy of the decay products (i.e., into kinetic energy of m1 and m2). Find linear momenta of decay products. Draw a vector diagram showing a range of momenta decay products can take.arrow_forwardAn experimentalist in a laboratory finds that a particle has a helical path. The position of this particle in the laboratory frme is given by r(t)= R cos(wt)i + R sin(wt)j + vztk R,vz, and w are constants. A moving frame has velocity (Vm)L= vzk relative to the laboratory frame. In vector form: A)What is the path of the partical in the moving frame? B)what is the velocity of the particle as a function of time relative to the moving frame? C)What is the acceleration of the particle in each frame? D)How should the accelerartion in each frame be realted?Does your answer to part c make sense?arrow_forwardThe Starship Enterprise returns from warp drive to ordinary space with a forward speed of 51 km/skm/s . To the crew's great surprise, a Klingon ship is 140 kmkm directly ahead, traveling in the same direction at a mere 30 km/skm/s . Without evasive action, the Enterprise will overtake and collide with the Klingons in just about 6.7 ss . The Enterprise's computers react instantly to brake the ship. What magnitude acceleration does the Enterprise need to just barely avoid a collision with the Klingon ship? Assume the acceleration is constant.arrow_forward
- To study Brownian motion, Bob, a student at the University of Ottawa, used his camera to capture the positions (i.e. displacements) of 50 microscopic dust particles floating on the surface of the water of his aquarium. By setting his camera to record 10 frames per second and noting down each particle's 2D displacement for each recorded frame, he managed to obtain the following mean-displacement vs time graph: 1600 1400 1200 2 1000 V 800 600 400 200 20 40 60 80 100 120 140 Time (s) a. Use the above mean-displacement graph to roughly approximate the diffusion coefficient D of the dust particles with units of um? /s. b. From the diffusion coefficient estimate in a), calculate the root mean- square displacement expected between each consecutive frame? c. Are the diffusion coefficient D and mean-square displacement calculated reasonable for a microscopic particle, or did Bob make a mistake in his calculations? Mean-square disp. (cm²)arrow_forwardParticle A has half the mass and eight times the kinetic energy of particle B. Part A What is the speed ratio VA/VB? VA/VB = Submit ——| ΑΣΦ VE Request Answer ?arrow_forwardR3B2. Alicia is a student on a passenger train moving at a constant velocity relative to the ground. She synchronizes her watch with the station clock as she passes through the town of Bannon station, and then compares her watch with the station clock as she passes through the Center town station farther down the line. The ground is an inertial frame, and the Bannon and Center clocks are synchronized in that frame. (1) Using a model or diagram, is the time she measures between the events of passing through these towns a proper time? (2) Is it a coordinate time in some inertial reference frame? (3) Is it the spacetime interval between the events?arrow_forward
- Imagine that you are going for a ride in a hot air balloon at the Canowindra Balloon Challenge. While the balloon is rising at a speed of 2.0 m/s, you throw a small ball down at a speed of 5.0 m/s relative to your body. A person who measures the ball's velocity at the instant of release will find that the ball's velocity relative to the ground at that instant is O a. 3.0 m/s, up. O b. 2.0 m/s, up. O c. 3.0 m/s, down. O d. 12.8 m/s, down. O e. 5.0 m/s, down.arrow_forwardfo 10. A square frame has sides that measure 2.00 m when it is at rest. What is the area of the frame when it moves parallel to one of its diagonal a speed of 0.72c as indicated in the figure? with m² ssf60 ssf60 ssf60 ssf60 s² sf60 ssf60 ssf60 ssf60 esf60 ssfor 50 ssf ss:60 ssi0 ssf603 js (9 08 09588 ssf60 ssf60 ssf60 ssi 60 ssf60 ssf60 ssf60 ssf60 ssf60 ssf60arrow_forward
arrow_back_ios
arrow_forward_ios