Question
thumb_up100%
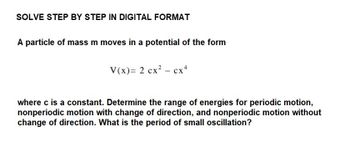
Transcribed Image Text:SOLVE STEP BY STEP IN DIGITAL FORMAT
A particle of mass m moves in a potential of the form
V(x)= 2 cx² cx4
where c is a constant. Determine the range of energies for periodic motion,
nonperiodic motion with change of direction, and nonperiodic motion without
change of direction. What is the period of small oscillation?
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
- Question 6: The dispersion relation of a system is given by w(k) = 2w, sin, where wo is a constant and n is an integer. 1. Calculate the group velocity vg. 2. Calculate the phase velocity Uph.arrow_forwardProblem 2 Consider the block of mass m, connected to a spring of spring constant k and placed on a inclined plane of angle a. Let la be the length of the spring at equili brium, and r be the elongation. The block oscillates and at the same time is rotating around origin 0, in the plane of the inclined, by a variable angular velocity . 1. Calculate the degrees of freedom of the block 2. What is the kinetic energy of the block 3. What is the potential energy of the block 4. Write the Lagrangian function (don't derive the Euler Lagrange equa- tions) k reference plane m o'arrow_forwardConsider a block of mass m attached to a spring with force constant k, as shown in the figure(Figure 1). The spring can be either stretched or compressed. The block slides on a frictionless horizontal surface, as shown. When the spring is relaxed, the block is located at x=0. If the block is pulled to the right a distance A and then released, A will be the amplitude of the resulting oscillations. Assume that the mechanical energy of the block-spring system remains unchanged in the subsequent motion of the block. Part A After the block is released from x=A, it will? Part B If the period is doubled, the frequency is? Part C An oscillating object takes 0.10 s to complete one cycle; that is, its period is 0.10 s. What is its frequency f? Part D If the frequency is 40 Hz, what is the period T ? Part E Which points on the x axis are located a distance A from the equilibrium position? Part F Suppose that the period is T. Which of the following points on the t axis are separated by the time…arrow_forward
- Please help me to solve thisarrow_forwardConsider a particle of mass m acted upon by a central potential (r is distance and a>0 is a constant): U (r) p2 a) What are the constants of the motion? b) Is a circular orbit possible in this potential? If so, state the conditions for it. c) Under what conditions will the particle fall to the center of the potential (r = 0)?arrow_forwardCalculate the energy, corrected to first order, of a harmonic oscillator with potential:arrow_forward
arrow_back_ios
arrow_forward_ios