A linear programming computer package is needed. Epsilon Airlines services predominately the eastern and southeastern United States. A vast majority of Epsilon's customers make reservations through Epsilon's website, but a small percentage of customers make reservations via phone. Epsilon employs call-center personnel to handle these reservations along with any problems with the website reservation system and for the rebooking of flights for customers if their plans change or their travel is disrupted. Staffing the call center appropriately is a challenge for Epsilon's management team. Having too many employees on hand is a waste of money, but having too few results in very poor customer service and the potential loss of customers. Epsilon analysts have estimated the minimum number of call-center employees needed by day of week for the upcoming vacation season (June, July, and the first two weeks of August). These estimates are given in the following table. s.t. Monday Tuesday Wednesday Thursday Friday Day Saturday Monday Tuesday Wednesday Thursday Friday Saturday Sunday Minimum Number of Employees Needed 60 The call-center employees work five consecutive days and then have two consecutive days off. An employee may start work any day of the week. Each call-center employee receives the same salary. Assume that the schedule cycles and ignore start-up and stopping of the schedule. Develop a model that will minimize the total number of call center employees needed to meet the minimum requirements. (Let X, the number of call-center employees who start work on day i where/1 Monday, 2 Tuesday, etc). = Min 45 40 45 80 75 35 Sunday Ху Хуа Ху Хар Ху Хар Худо Find the optimal solution. (X₁ X₂ X₂ X₁ X₂ Xq Xy) | Give the number of call-center employees that exceed the minimum required. (M. Tu. W, Th, F, Sa. Su)
A linear programming computer package is needed. Epsilon Airlines services predominately the eastern and southeastern United States. A vast majority of Epsilon's customers make reservations through Epsilon's website, but a small percentage of customers make reservations via phone. Epsilon employs call-center personnel to handle these reservations along with any problems with the website reservation system and for the rebooking of flights for customers if their plans change or their travel is disrupted. Staffing the call center appropriately is a challenge for Epsilon's management team. Having too many employees on hand is a waste of money, but having too few results in very poor customer service and the potential loss of customers. Epsilon analysts have estimated the minimum number of call-center employees needed by day of week for the upcoming vacation season (June, July, and the first two weeks of August). These estimates are given in the following table. s.t. Monday Tuesday Wednesday Thursday Friday Day Saturday Monday Tuesday Wednesday Thursday Friday Saturday Sunday Minimum Number of Employees Needed 60 The call-center employees work five consecutive days and then have two consecutive days off. An employee may start work any day of the week. Each call-center employee receives the same salary. Assume that the schedule cycles and ignore start-up and stopping of the schedule. Develop a model that will minimize the total number of call center employees needed to meet the minimum requirements. (Let X, the number of call-center employees who start work on day i where/1 Monday, 2 Tuesday, etc). = Min 45 40 45 80 75 35 Sunday Ху Хуа Ху Хар Ху Хар Худо Find the optimal solution. (X₁ X₂ X₂ X₁ X₂ Xq Xy) | Give the number of call-center employees that exceed the minimum required. (M. Tu. W, Th, F, Sa. Su)
Practical Management Science
6th Edition
ISBN:9781337406659
Author:WINSTON, Wayne L.
Publisher:WINSTON, Wayne L.
Chapter2: Introduction To Spreadsheet Modeling
Section: Chapter Questions
Problem 20P: Julie James is opening a lemonade stand. She believes the fixed cost per week of running the stand...
Related questions
Question
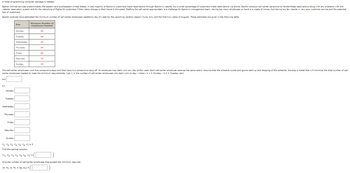
Transcribed Image Text:### Epsilon Airlines Call-Center Staffing Model
**Introduction:**
Epsilon Airlines services predominantly the eastern and southeastern United States. Most of Epsilon's customers make reservations online, but some use phone reservations. Call-center personnel handle these reservations and support for booking changes. Efficient staffing is crucial to avoid excessive costs or poor service.
**Staffing Requirements:**
Epsilon analysts estimate the minimum number of employees needed for each day during the vacation season (June, July, and the first two weeks of August) as follows:
| Day | Minimum Number of Employees Needed |
|-----------|------------------------------------|
| Monday | 60 |
| Tuesday | 45 |
| Wednesday | 40 |
| Thursday | 45 |
| Friday | 80 |
| Saturday | 75 |
| Sunday | 35 |
**Work Schedule:**
- Employees work five consecutive days followed by two days off.
- Employees can start on any day of the week.
- All employees receive equal pay.
- Schedules are cyclic and independent of start/stop transitions.
**Objective:**
Develop a model to minimize the total number of call-center employees while meeting daily staffing needs. Use the following notation:
- Let \( x_i \) be the number of employees starting work on day \( i \) (where \( i = 1 \) for Monday, \( i = 2 \) for Tuesday, etc.).
**Optimization Model:**
- Objective: Minimize the total employees \( (x_1 + x_2 + x_3 + x_4 + x_5 + x_6 + x_7) \)
- Subject to constraints:
- Monday: \( \begin{array}{c|c}
& \\
& \\
& \\
\end{array} \)
- Tuesday: \( \begin{array}{c|c}
& \\
& \\
& \\
\end{array} \)
- Wednesday: \( \begin{array}{c|c}
& \\
& \\
& \\
\end{array} \)
- Thursday: \( \begin{array}{c|c}
& \\
& \\
& \\
\end{array} \)
- Friday: \( \begin{array}{c|c}
& \\
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 6 images
Recommended textbooks for you
Practical Management Science
Operations Management
ISBN:
9781337406659
Author:
WINSTON, Wayne L.
Publisher:
Cengage,
Operations Management
Operations Management
ISBN:
9781259667473
Author:
William J Stevenson
Publisher:
McGraw-Hill Education
Operations and Supply Chain Management (Mcgraw-hi…
Operations Management
ISBN:
9781259666100
Author:
F. Robert Jacobs, Richard B Chase
Publisher:
McGraw-Hill Education
Practical Management Science
Operations Management
ISBN:
9781337406659
Author:
WINSTON, Wayne L.
Publisher:
Cengage,
Operations Management
Operations Management
ISBN:
9781259667473
Author:
William J Stevenson
Publisher:
McGraw-Hill Education
Operations and Supply Chain Management (Mcgraw-hi…
Operations Management
ISBN:
9781259666100
Author:
F. Robert Jacobs, Richard B Chase
Publisher:
McGraw-Hill Education
Purchasing and Supply Chain Management
Operations Management
ISBN:
9781285869681
Author:
Robert M. Monczka, Robert B. Handfield, Larry C. Giunipero, James L. Patterson
Publisher:
Cengage Learning
Production and Operations Analysis, Seventh Editi…
Operations Management
ISBN:
9781478623069
Author:
Steven Nahmias, Tava Lennon Olsen
Publisher:
Waveland Press, Inc.