Question
I only need help with part e)
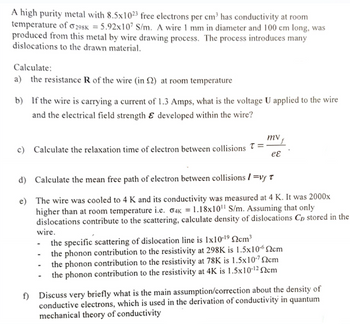
Transcribed Image Text:A high purity metal with 8.5x1023 free electrons per cm³ has conductivity at room
temperature of 298K = 5.92x107 S/m. A wire 1 mm in diameter and 100 cm long, was
produced from this metal by wire drawing process. The process introduces many
dislocations to the drawn material.
Calculate:
a) the resistance R of the wire (in 2) at room temperature
b) If the wire is carrying a current of 1.3 Amps, what is the voltage U applied to the wire
and the electrical field strength & developed within the wire?
c) Calculate the relaxation time of electron between collisions =
mv
d) Calculate the mean free path of electron between collisions=vy T
eɛ
e) The wire was cooled to 4 K and its conductivity was measured at 4 K. It was 2000x
higher than at room temperature i.e. σ4K = 1.18x10" S/m. Assuming that only
dislocations contribute to the scattering, calculate density of dislocations Cp stored in the
wire.
the specific scattering of dislocation line is 1x10-19 cm³
- the phonon contribution to the resistivity at 298K is 1.5x10-62cm
-the phonon contribution to the resistivity at 78K is 1.5x10-72cm
the phonon contribution to the resistivity at 4K is 1.5x10-12 Qcm
f) Discuss very briefly what is the main assumption/correction about the density of
conductive electrons, which is used in the derivation of conductivity in quantum
mechanical theory of conductivity
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps
