(a) Fibonacci posed the following problem: Suppose that rabbits live forever and that every month each pair produces a new pair which becomes productive at age 2 months. If we start with one nowoorn pan v many pairs of rabbits will we have in the nth month? Show that the answer is f, where (f.)}} is the Fibonacci sequence defined recursively by the following conditions. n = fn -1+n- 2 n2 3 From Let r, be the number of rabbit pairs in the nth month. Since we're starting with one newborn pair, r, = 1, and since each pair of rabbits only produces a new pair when the pair is 2 mor hs old, r, - the third month forward, thke original pair of ra bits will begin to produce a new of rabbits each month. f2 = 1 mont will be too young to reproduce, but all rabbits present in the (nth - 2) month can. The number of producingirs each month willed In general, in the nth month, the rabbits born n the (nth - to the pairs already present the previous month Which of the following comple s the proof? O Thus, r.- 1 =n+n-2 Thus, r, = rn -1+n -2 Thus, r, = ro -n - 2 Thus, r,-n- 1-n- 2 %3D Thus, r,-ro+n-2 So {r,) = (). 1 -? 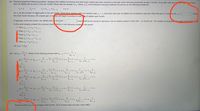'n +1 Which of the following shows that a,1 =1+ fo (b) Let a,= an-2 %3D 1 fn +1 - an -1 an = -1+n-2 -1+ n-2 -1+ In-1 = 1 + fn-1/fn-2 » an-1 %3D fn -1 fn - 1 1 a, - 2 fo =1+ fn+1 - an -1 an= fo fn+ fn - 1 - 1 + fn - 1 = 1+ an - 2 » an %3D 1-1 fn- 1 1 fn-1+ fn-2 -1+ n-1-1+ - 1+ fn- 2fn -1 = 1+ fn+1 - an -1 -2 an= n-2 an - 2 1 fn - 1 = 1 + n-1 = 1+ fn- 2 = 1 + fn-1- fn+1 - an -1 an fo fn- 2fn-1 + an - fn-1+n-2 1 an - 2 fn+1 - an -1- O a.= fn+2 - n+1+fn - 1+'n = 1 + fn+1 = 1 + ffn+1 » an -1 fn + 1 an- 2 f+1 Assuming that (a,) is onvergent, find its limit. (If an answer does not exist, enter DNE.)

Q: dt VR-2t + 26

Q: Use the sum-to-product identity to find an expression that is equivelentto cust 15)- cos(5ØL Frovide…

Q: S. S. dy dx

Q: Number 19

Q: J〇 is

Q: de de

Q: Translate

Q: Both please