(a) Determine the exact time, tr, at which the rock hits the river by solving the equation h(t) = 0 for t. (b) Sketch a graph of y = h (t) for 0≤t≤t seconds. (c) Using your graph from (b), deduce the maximum height attained by the rock above the river.
(a) Determine the exact time, tr, at which the rock hits the river by solving the equation h(t) = 0 for t. (b) Sketch a graph of y = h (t) for 0≤t≤t seconds. (c) Using your graph from (b), deduce the maximum height attained by the rock above the river.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
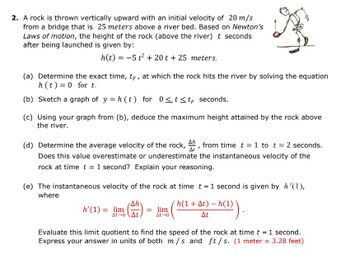
Transcribed Image Text:2. A rock is thrown vertically upward with an initial velocity of 20 m/s
from a bridge that is 25 meters above a river bed. Based on Newton's
Laws of motion, the height of the rock (above the river) t seconds
after being launched is given by:
h(t) = -5t² + 20 t + 25 meters.
(a) Determine the exact time, tr, at which the rock hits the river by solving the equation
h (t) = 0 for t.
(b) Sketch a graph of y = h (t) for 0≤t≤t seconds.
(c) Using your graph from (b), deduce the maximum height attained by the rock above
the river.
(d) Determine the average velocity of the rock, Ah, from time t = 1 to t = 2 seconds.
Does this value overestimate or underestimate the instantaneous velocity of the
rock at time t = 1 second? Explain your reasoning.
(e) The instantaneous velocity of the rock at time t = 1 second is given by h'(1),
where
Ah
h' (1) = lim
= lim
At-0 At. At-0
(h(₁.
(¹).
h(1 + At) - h(1)
At
Evaluate this limit quotient to find the speed of the rock at time t = 1 second.
Express your answer in units of both m/s and ft/s. (1 meter≈ 3.28 feet)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 3 steps with 2 images
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
