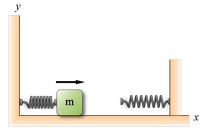
A crate of mass m = 0.24 kg is set against a spring with a spring constant of k1 = 605 N/m which has been compressed by a distance of 0.1 m. Some distance in front of it, along a frictionless surface, is another spring with a spring constant of k2 = 487 N/m. The crate is not connected to the first spring and may slide freely. 1) How far, d2 in meters, will the second spring compress when the crate runs into it?  2) How fast, v in meters per second, will the crate be moving when it strikes the second spring?  3) Now assume that the surface is rough (that is, not frictionless). You perform the experiment and observe that the second spring only compresses a distance d2/2. How much energy, in joules, was lost to friction?

Imagine a skydiver with a mass of m jumping out of an airplane at an altitude of h. As the skydiver descends, they experience two forces: air resistance and gravity. The force of air resistance varies with time and is described by the following function, where t is the time in seconds since t e skydiver has jumped: vec 2 (F)(t) = (0.5t)ha (x) + (0.11 +0.3t)hat(y) a) Create a air free body diagrar for the skydiver at t = 2 seconds. b) - What is the net for e acting on the skydiver at t = 2 seconds? c) What i the magnitude of the acceleration of the skydiver at t = 2 seconds? Imagine a skydiver with a mass of m jumping out of an airplane at an altitude of h. As the skydiver descends, they experience two forces: air resistance and gravity. The force of air resistance varies with time and is described by the following function, where t is the time in seconds since the skydiver has jumped: Fair (t) = (0.5t)2 + (0.12 + 0.3t)ŷ a) Create a free-body diagram for the skydiver at t = 2 seconds.…

The small mass m = 3.5 kg and big mass M = 5.8 kg are initially at rest with the small mass at the top of the inclined surface. The small mass will start sliding down the large mass M without friction. The large mass itself is also free to move on a frictionless horizontal surface. Given that h = 4.6 meter, what will the magnitude of the velocity of the mass M when it reaches the horizontal surface? Take g =9.81 m/s? Provide your answer with 2 decimal places. h M

An ideal spring can compress 2.33 cm by a force of 555 N, a block of mass m = 3.18 kg is released from rest on an inclined plane, the onclination of the plane is 32 degrees,  the block rest momentarly when the spring is compress 5.48 cm. Find the distance d that the block travels from the begining of the motion until it reaches the spring. (g = 10 m/s2 ).

>V=0 d (m A block of mass m is on an inclined ramp. The ramp makes an angle with respect to the horizontal, as shown. The ramp has friction, with coefficient of kinetic friction and static friction μs. This experiment takes place on earth. The block has an initial speed of v up the ramp. It travels a distance d along the ramp before it stops. There are no numbers in this problem! Answer all questions in terms of the given variables (g, m, µk, µs, 8, v, d) only. Do not use any other variables. a) Draw a free body diagram clearly showing all the forces acting on the block while it is moving up the ramp. b) Calculate the work done by the Normal force as the block travels the distance d. Is it positive, negative, or zero? c) Calculate the work done by the Weight force as the block travels the distance d. Is it positive, negative, or zero? d) Calculate the work done by the Friction force as the block travels the distance d. Is it positive, negative, or zero? e) If the block comes to rest,…

A massless spring with spring constant 26 N/m hangs vertically. A body of mass 1.0 kg is attached to its free end and then released. Assume that the spring was unstretched before the body was released. Find how far below the initial position the body descends. I don't understand why I'm getting this first part wrong specifically because the formula is just x= mg/k because F=kx.

A particle moving in the xy plane follows a path described as a function of time by i(t) = (3.4 m/s)tî - (2.8 m/s?)tj. A constant force given by F = (5.0î - 2.0j) N acts on the particle (note that other forces must act on the particle as well if it follows the path given above). (a) Determine the displacement of the particle during the interval t = 1.0 s to t = 4.0 s. AT = îm - m (b) Calculate the work done by F during this interval. W =

4

A small boat is being pushed up a ramp into the back of a flatbed truck. The boat is sitting at the top of the ramp, which is located 1.4 m above the ground. The boaters think the boat is safe there, and begin to walkaway. However, they have neglected the thin layer of ice that has formed on the ramp, which is angled at 30◦ up from the ground. Neglecting the friction between the boat and the ramp, how long do the boaters havebefore the boat reaches the bottom of the ramp?

A crate with mass m = 20 kg is being pushed to the right by a force of magnitude F = 80 N The crate is placed on a rough horizontal coefficient of kinetic friction surface with a HK= 0.2 Take g = 10 m/s² The acceleration of the crate is: 3.8 m/s^2 O 2 m/s^2 O 6 m/s^2 4 m/s^2