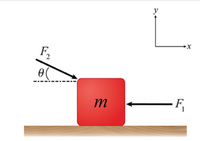
A block with a mass of m = 32 kg rests on a frictionless surface and is subject to two forces acting on it. The first force is directed in the negative x-direction with a magnitude of F1. The second has a magnitude of F2 and acts on the body at an angle θ = 23° measured from horizontal, as shown. Write an expression for the magnitude of the normal force, FN, acting on the block, in terms of F2 and the other variables of the problem. Assume that the surface it rests on is rigid.

Determine the force Q-> when the block moves with constant velocity. Express your answer in vector form.

In the figure, a crate of mass m = 99 kg is pushed at a constant speed up a frictionless ramp (0 = 30°) by a horizontal force . The positive direction of an x axis is up the ramp, and the positive direction of a y axis is perpendicular to the ramp. (a) What is the magnitude of F? (b) What is the magnitude of the normal force on the crate? (a) Number i (b) Number Units Units II ATTIT This answer has no units °(degrees) m kg

A crate is sitting on a horizontal surface where the coefficient of static friction is 0.60. A worker pushes horizontally on the crate, which has a mass of 45 kg. The crate does not move, and there are no other forces acting on it. Which of the following statements is true?       (a) The force of friction on the crate is mgμs.       (b) The force of friction on the crate is mgμk.       (c) The force of friction on the crate is greater than the force that the worker exerts on the crate.           (d) The force exerted on the worker by the crate is equal in magnitude to the force of friction exerted by the surface on the crate.

A block with a mass of m = 22 kg rests on a frictionless surface and is subject to two forces acting on it. The first force is directed in the negative x-direction with a magnitude of F1 = 12 N. The second has a magnitude of F2 = 18.25 N and acts on the body at an angle θ = 19° measured from horizontal, as shown. Write an expression for the magnitude of the normal force, FN, acting on the block, in terms of F2, g, and the other variables of the problem. Assume that the surface it rests on is rigid.  Write an expression for the component of net force, Fnet,x, in the x-direction, in terms of the variables given in the problem statement.  Part (d)  Find the block's acceleration in the x-direction, ax, in meters per second squared.

Tom enlists the help of his friend John to move his car. They apply forces to the car. F1 = 430 N and F2 = 347 N and friction is negligible. Mass of car = 3500 kg O1= -25 degrees and O2 = 12 degrees (assume the car faces the positive x-axis before the forces are applied. A) Find the force (in N) exerted on the car     Magnitude =     Direction (counterclockwise from the +x-Axis) = ______________ degrees B) What is the acceleration (in m/s squared) of the car?     Magnitude =   __________ m/s squared     direction (counterclockwise from the +x-Axis) ______________ degrees

Two objects (m1=11.0 kg and m2=3.00 kg) are separated by 40.0 cm. A third object (m3=1.00 kg) is placed at a location along the line connecting them such that the net force acting on it is zero. By considering the force vectors, this location must be between the two original objects. We will define x as the distance between m1 and m3 and y as the distance between m2 and m3.   1) Find the distance between 11.0-kg object and 1.00-kg object along the line AB where a small, 1.00-kg object could rest such that the net gravitational force on it due to the two objects shown is exactly zero. (Express your answer to two significant figures.)

In a two-dimensional tug-of-war, Alex, Betty, and Charles pull horizontally on an automobile tire at the angles shown in the picture. The tire remains stationary in spite of the three pulls. Alex pulls with force F A of magnitude 214 N, and Charles pulls with force F c of magnitude 183 N. Note that the direction of F c is not given. What is the magnitude of Betty's force F B if Charles pulls in (a) the direction drawn in the picture or (b) the other possible direction for equilibrium? Alex Charles 145° Betty (a) Number i Units (b) Number i Units >

In the figure here, a box of Cheerios (mass mc = 1.70 kg) and a box of Wheaties (mass mw = 2.60 kg) are accelerated across a horizontal surface by a horizontal force applied to the Cheerios box. The magnitude of the frictional force on the Cheerios box is 2.10 N, and the magnitude of the frictional force on the Wheaties box is 4.60 N. If the magnitude of F is 12.7 N, what is the magnitude of the force on the Wheaties box from the Cheerios box? Number i Unit

(Problem 3:   A horizontal force, F1 = 55 N, and a force, F2 = 16.3 N acting at an angle of θ to the horizontal, are applied to a block of mass m = 2.6 kg. The coefficient of kinetic friction between the block and the surface is μk = 0.2. The block is moving to the right.  Question: Solve numerically for the magnitude of the normal force, FN in Newtons, that acts on the block if θ = 30°.  and, Solve numerically for the magnitude of acceleration of the block, a in m/s2, if θ = 30°.