A 2000-kg automobile starts from rest at point A on a 6° incline and coasts through a distance of 120 m to point B. The brakes are then applied, causing the automobile to come to a stop at point C, 20 m from B. 20 m AB m B C 6° Knowing that slipping is impending during the braking period and neglecting air resistance and rolling resistance, determine the coefficient of static friction between the tires and the road.
Newton's second law of Motion:
According to this law, the magnitude of the net force on an object of constant mass is given as,
where is the mass and is the magnitude of the net acceleration of the object.
Friction force:
The friction force is the force that is responsible for opposing any relative motion between two surfaces in contact. The friction force is given by the formula,
where is the normal force and is the coefficient of friction.
The acceleration of a rolling object on an inclined plane:
The acceleration of a rolling object along the inclined plane when the rolling friction and other resistive forces are neglected is given by the formula,
where is the acceleration due to gravity and is the inclination angle.
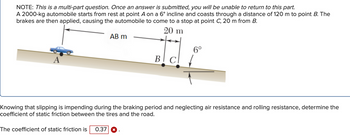
NOTE:
Trending now
This is a popular solution!
Step by step
Solved in 6 steps with 1 images
