
Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Question
1. Find the measure of
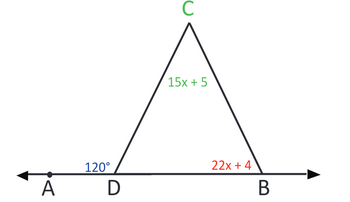
Transcribed Image Text:The image shows a geometric diagram involving a triangle and a straight line. Here's a detailed explanation:
**Diagram Description:**
- There is a straight line, represented by points A, D, and B. Point D is between points A and B on the line.
- From point D, a triangle is formed with vertices D, B, and C.
**Line Segment:**
- The segment extending from point A through D to B forms a straight angle.
- The angle between segments AD and DB is marked as \(120^\circ\).
**Triangle:**
- The triangle is labeled as \(\triangle DBC\), with vertex C above point D.
- The angle at vertex C formed by lines DC and BC is labeled with the expression \(15x + 5\).
- The angle at vertex B of the triangle is labeled with the expression \(22x + 4\).
This geometric setup illustrates the relationship between angles in a triangle and a straight line, ideal for exploring angle sum properties and linear equations involving unknowns like \(x\).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Similar questions
- If m∠5 = x°, which angles also have a measure of x°? Select all that apply. A.∠1 B.∠4 C.∠9 D.∠12arrow_forward2. The measure of angle A in an equilateral triangle is 5x + 5. Solve for x. A. x = 11 B. x = 35 A C. x 34 %3D D. x 60 %3Darrow_forwardIn A LPM, which angle is the smallest? 11 10 P. AM 12 Your answer: ZL ZP ZMarrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning