A First Course in Probability (10th Edition)
A First Course in Probability (10th Edition)
10th Edition
ISBN: 9780134753119
Author: Sheldon Ross
Publisher: PEARSON
Bartleby Related Questions Icon

Related questions

Q: Find the standard deviation for the following group of data items. 14, 16, 16, 21 The standard…
A: The first step in calculating the standard deviation is to find the mean (average) of the data set.…
Q: Needed asap and show all answers and round!
A: General Formula:Probability=number of favorable outcomes / number of possible outcomes 1. The number…
Q: Prove that if events A and B are independent, then...(a)  A and Bc are independent. (Hint: Split up…
A:
Q: Which of the following indicate why you should transform a set of raw scores into a set of Z scores?…
A: A Z score is a statistical measurement that describes a value's relationship to the mean of a group…
Q: Find a probability density function f(x) for the distribution whose CDF is below. Make sure to be…
A: Let CDF = F(x) which is a Cumulative Distribution Function  Let PDF = f(x) = Probability Density…
Q: You are a high school student council member tasked with analyzing how much time students in your…
A: ### Given DataThe data representing the number of hours 16 students spent on extracurricular…
Q: Help me solve 14
A: Step 1:Step 2:
Q: 13. A committee of 12 is to be randomly selected from 15 science teachers and x math teachers. If…
A: Step 1:number of teachers to be selected =12number of available science teacher =15number of…
Q: Let A and B be events with P(A)=0.4 and P(B)= 0.6 and P (A and B) =0.2 . (a) Are A and B…
A: Two events A and B are independent if the probability of both events occurring is the product of the…
Q: 4. Plasma aldosterone has been related to blood-pressure levels in adults in some studies. In…
A: To analyze the plasma aldosterone levels between Caucasian and African American children, we can use…
Q: 2. Waluigi and Bowser play a game where they take turns flipping a fair coin. The first one to flip…
A: If Waluigi goes first, then Bowser is getting turns to flip the coin at even positions (second,…
Q: 8.21 A soft-drink machine is regulated so that the amount of drink dispensed averages 240…
A: The answer provided is correct and complete. It accurately follows the given information and…
Q: Part C only
A: The given values are:Mean (μ) = 60 grams per mililiterStandard deviation (σ) = 9.2 grams per…
Q: Based on a poll, among adults who regret getting tattoos, 22% say that they were too young when they…
A: Let's solve the problem step-by-step using probability concepts. This problem involves a binomial…
Q: What is the probability of choosing a penny from a jar of coins that contains 12 pennies, 19…
A: The objective of this question is to find the probability of choosing a penny from a jar of coins…
Q: Given that n(AB) = 8, n(An Bn C) = 7, n(An C) = 10, n(BNC)=7, n(An C') = 3, n(Bn C') = 6, n(C) = 19,…
A: Step 1: Step 2: Step 3: Step 4:
Q: Consider two boxes: box I contains 3 blue and 2 red marbles; box II contains 3 blue and 5 red…
A: Let's denote the following:B1: Event that the marble drawn is blue from box IB2: Event that the…
Q: Suppose that X and Y are independent random variables with probability densities and and g(x): = x >…
A:
Q: Consider the following CDF: a) Sketch the graph of F(x) x<0 F(x)=(x+1) 0≤x≤1 x ≥ 1 b) Find the…
A: ### (a) Sketch the graph of  F(x) : To sketch the graph of \( F(x) \):1. For  x<0**,  F(x)=0.…
Q: None
A: Step 1: Step 2: Step 3: Step 4:
Q: When coin 1 is flipped, it lands on heads with probability 3; when coin 2 is flipped it lands on…
A: Step 1:Part (a) :If coin 1 is flipped 12 times, find the probability that it lands on heads at least…
Q: Consider an irreducible Markov chain X on the state space S = {a, b, c, d, e, f} with transition…
A:
Q: Suppose X and Y have joint PDFfX,Y (x, y) = cxy if 0 &lt; x &lt; y &lt; 1                    0…
A: a) Find the value of ccc that makes this a valid joint PDF.To be a valid joint probability density…
Q: Let U ∼ Unif(0, 1) and X ∼ Expo(1) be independent r.v.s. Find the PDF ofU + X
A: Step 1: To find the PDF of W = U + X where U∼Unif(0,1) and X∼Expo(1) are independent random…
Q: not use ai please
A: Recall that the z-score formula is given byZ=σX−μ​whereX is the scoreμ is the population mean, andσ…
Q: A variable X is normally distributed with a varianceof 125. To estimate the mean with a margin of…
A:
Q: Tyler decides which type of pizza to order. The choices for crust are thin crust or regular crust.…
A: The objective of this question is to determine the total number of possible outcomes in the sample…
Q: In all of the following questions, let be a sample space with a probability measure P, and let A, BC…
A: Step 1: Step 2:
Q: Let X have PMF P(X = k) = −p^k/(k log(1 − p)) for k = 1, 2, 3, ...,where p is a parameter such that…
A: Let X be a random variable. The expected value of X or the mean of X, denoted by E(X) or μX​ is…
Q: not use ai please ensjejejejjdjfjfjfjftjrjdjsje
A: Here is the python code attatched to calculate you can also run in google colab:import scipy.stats…
Q: PLEASE LET EXPERT ANSWER, NO AI!!!!!!! PLEASE LET EXPERT ANSWER, NO AI!!!!!!! PLEASE LET EXPERT…
A: (a) The mean is calculated as xˉ=n1​∑xi​, where n is the sample size and xi is the data point in the…
Q: A questionnaire was given to students. The first question asked was "How stressed have you been in…
A: The data provided is a frequency distribution table of stress ratings from a student survey. The…
Q: 8.29 The distribution of heights of a certain breed of terrier has a mean of 72 centimeters and a…
A: Given:Mean height of terriers (μT​): 72 cmStandard deviation of terriers' heights (σT​): 10 cmSample…
Q: อธิบาย
A: English Translation
Q: The output voltage of a power supply is normally distributed with mean 5 V and standard deviation…
A: Step 1:Given data : Mean μ=5Standard deviation σ=0.02Let X be the random variable that represents…
Q: For each sequence X1, X2, ... of i.i.d. random variables, determine limn→∞ Xn.(a)  Xi ∼Unif(0,…
A: Approach to solving the question:Let's analyze the behavior of each sequence of i.i.d. random…
Q: A survey of 500 book buyers was conducted and the data was obtained; at Casa del Libro 150, Sótano…
A: Step 1:1. Total number of book buyers surveyed: 5002. Number of buyers at Casa del Libro: 1503.…
Q: List which rows or columns dominate other rows or columns. Then, remove any dominated strategies in…
A: Step 1: Note: Kindly give me helpful rating          (Thanks you)
Q: Please break it down so that as I compare my work, I am able to identify my mistakes.
A:
Q: 4-2. 1 A calculator with a random number generator produces the following sequence of random…
A: a)To find the sample mean, add all values and then divide it by the number of values.…
Q: can you pleasaes show the working out for this question
A: detailed explanation step-by-step:The key idea here is to use the "birthday problem" to solve this…
Q: can someone help me with this probability question
A: First, we need to calculate the total number of parts in the inventory. This is the sum of the parts…
Q: I want a question that talks about networks according to the topic of probability data analysis with…
A: Probability of all events occurring together: P(all servers fail)=P(A)×P(B)×P(C) Probability of at…
Q: A coin comes up heads with probability p and tails with probability q=1−p. For the moment, assume…
A: Step 1:To determine the expected number of coin flips needed to produce the pattern "HT" for the…
Q: Let U ∼ Unif(0, 1). As a function of U, construct a r.v. X with desired CDFF(x) = 1 − e^−x^3 for x…
A:
Q: Let X be a continuous random variable with probability density function f(x) otherwise 5x x > 1 =…
A: Step 1:Step 2:Step 3:Step 4:
Q: 3.2. The probability mass function of the random variable X is given as follows. X -3 6 9 p(x) =…
A: 1) a) E(X) is given as, E(X)=∑x∗P(X=x)=−3∗1/6+6∗1/2+9∗1/3=5.5 E(X^2) is given as,…
Q: Suppose that the lifespan of a certain model of laptop is normally distributed with mean4 years and…
A: Given:mean µ = 4 years convert in months = 4 x 12 = 48 monthsstandard deviation σ = 4 months The…
Q: Suppose 59% of the students in a university are baseball players. If a sample of 632 students is…
A: The given values are:Population proportion (p) = 59% = 0.59Sample size (n) = 632Desired sample…
Q: It was found at the CHOME factory that the time requiredto manufacture a certain part is a normally…
A:
Question
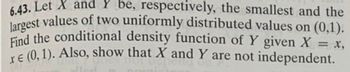
6.43. Let X and Y be, respectively, the smallest and the largest values of two uniformly distributed values on (0,1). Find the conditional density function of Y given X € (0,1). Also, show that X and Y are not independent. = x,
expand button
Transcribed Image Text:6.43. Let X and Y be, respectively, the smallest and the largest values of two uniformly distributed values on (0,1). Find the conditional density function of Y given X € (0,1). Also, show that X and Y are not independent. = x,
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
bartleby

Step by stepSolved in 2 steps

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
A First Course in Probability (10th Edition)
Probability
ISBN:9780134753119
Author:Sheldon Ross
Publisher:PEARSON
Text book image
A First Course in Probability
Probability
ISBN:9780321794772
Author:Sheldon Ross
Publisher:PEARSON
SEE MORE TEXTBOOKS