Inquiry into Physics
8th Edition
ISBN: 9781337515863
Author: Ostdiek
Publisher: Cengage
expand_more
expand_more
format_list_bulleted
Related questions
Question
PP6
![**Transcription for Educational Website**
**Problem Statement:**
6. Show that \( q(t) = q_0 \exp(-t/RC) \) is a solution of the ordinary differential equation (ODE):
\[ Ri(t) + \frac{q(t)}{C} = 0 \]
**Explanation:**
This problem involves showing that the given function \( q(t) = q_0 \exp(-t/RC) \) satisfies the provided ordinary differential equation. The ODE is a first-order linear differential equation commonly seen in the context of electrical circuits, specifically RC (resistor-capacitor) circuits. We are asked to verify if the exponential function, which represents the charge \( q \) over time \( t \), is a solution by substituting it into the equation.
**Notes:**
- \( q(t) \) denotes the charge as a function of time.
- \( q_0 \) is the initial charge.
- \( \exp \) refers to the exponential function.
- \( R \) is the resistance.
- \( C \) is the capacitance.
- \( i(t) \) implies the current as a function of time.
**Application:**
To verify, differentiate \( q(t) \) with respect to time \( t \), relate it to the current function \( i(t) \), and substitute into the ODE to check if it holds true. This problem highlights how exponential decay models the discharge of a capacitor in an RC circuit.](https://content.bartleby.com/qna-images/question/c3edbe93-d39c-43f4-8aa9-2c034a88e626/318b5abb-dcfa-47dd-b5bf-ff9ce2153517/q09ke4l_processed.jpeg)
Transcribed Image Text:**Transcription for Educational Website**
**Problem Statement:**
6. Show that \( q(t) = q_0 \exp(-t/RC) \) is a solution of the ordinary differential equation (ODE):
\[ Ri(t) + \frac{q(t)}{C} = 0 \]
**Explanation:**
This problem involves showing that the given function \( q(t) = q_0 \exp(-t/RC) \) satisfies the provided ordinary differential equation. The ODE is a first-order linear differential equation commonly seen in the context of electrical circuits, specifically RC (resistor-capacitor) circuits. We are asked to verify if the exponential function, which represents the charge \( q \) over time \( t \), is a solution by substituting it into the equation.
**Notes:**
- \( q(t) \) denotes the charge as a function of time.
- \( q_0 \) is the initial charge.
- \( \exp \) refers to the exponential function.
- \( R \) is the resistance.
- \( C \) is the capacitance.
- \( i(t) \) implies the current as a function of time.
**Application:**
To verify, differentiate \( q(t) \) with respect to time \( t \), relate it to the current function \( i(t) \), and substitute into the ODE to check if it holds true. This problem highlights how exponential decay models the discharge of a capacitor in an RC circuit.
Expert Solution
arrow_forward
Step 1
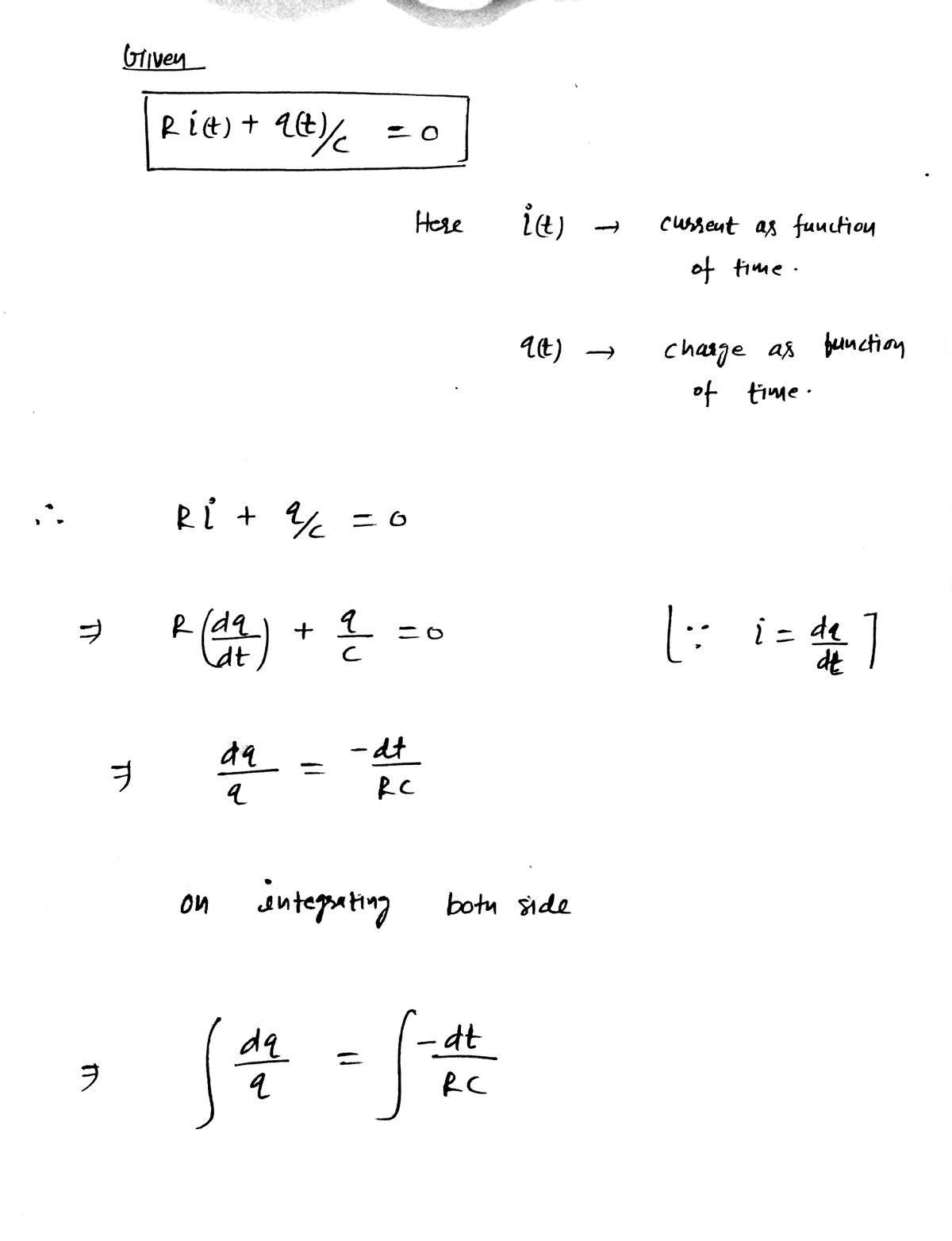
Step by stepSolved in 2 steps with 2 images
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Integrated Concepts (a) What is the average power output of a heart defibrillator that dissipates 400 J of energy in 10.0 ms? (b) Considering the high-power output, why doesn’t the defibrillator produce serious burns?arrow_forwardCalculate the speed of (a) an electron and (b) a proton with a kinetic energy of 1.00 electron volt (eV). (c) Calculate the average translational kinetic energy in cV of a 3.00 102 K ideal gas particle. (Recall from Topic 10 that 12mv2 = 32kBT.)arrow_forwardProfessor Edward Ney was the founder of infrared astronomy at the University of Minnesota. In his later years, he wore an artificial pacemaker. Always an experimentalist, Ney often held a strong laboratory magnet near his chest to see what effect it had on his pacemaker. Perhaps he was using the magnet to throw switches that control different modes of operation. An admiring student (without an artificial pacemaker) thought it would be fun to imitate this great man by holding a strong magnet to his own chest. The natural pacemaker of the heart (known as the sinoatrial node) carries a current of about 0.5 mA. Estimate the magnetic force exerted on a natural pacemaker by a strong magnet held to the chest. How do you think the student might have felt during the experiment? Explain your geometric assumptions. Hints: See Table 30.1 (page 941) to estimate the magnetic field, and assume the field is roughly uniform. Use Figure P30.58 to estimate the size of the sinoatrial node; your heart is about the size of your fist. FIGURE P30.58arrow_forward
- An evacuated tube uses an accelerating voltage of 40 kV to accelerate electrons to hit a copper plate and produce x rays. Non-relativistically, what would be the maximum speed of these electrons?arrow_forwardLunar astronauts placed a reflector on the Moon's Surface, off which a laser beam is periodically reflected. The distance to the Moon is calculated from the round-trip time. (a) To what accuracy in meters can the distance to the Moon be determined, if this time can be measured to 0.100 ns? (b) What percent accuracy is this, given the average distance to the Moon is 3.84108m ?arrow_forwardYou are working for SETI, the Search for Extraterrestrial Intelligence. One day, you receive a radio communication from an alien intelligence. Although you cannot understand their language, they have included some photos from an I Love Lucy episode. The photos allow you to determine that it is the episode in which Lucy makes a television commercial on Vitameatavegamin. This episode first aired on CBS on May 5, 1952. Before running to your supervisor to tell him the news, you quickly determine how far away in light-years the alien civilization is.arrow_forward
- Radio station WWVB, operated by the National Institute of Standards and Technology (NIST) from Fort Collins, Colorado, at a low frequency of 60 kHz, broadcasts a time synchronization signal whose range covers the entire continental US. The timing of the synchronization signal is controlled by a set of atomic clocks to an accuracy of 101012 s, and repeats every 1 minute. The signal is used for devices, such as radio-controlled watches, that automatically synchronize with it at preset local times. WWVB's long wavelength signal tends to propagate close to the ground. (a) Calculate the wavelength of the radio waves from WWVB. (b) Estimate the error that the travel time of the signal causes in synchronizing a radio controlled watch in Norfolk, Virginia, which is 1570 mi (2527 km) from Fort Collins, Colorado.arrow_forwardThe planetary model of the atom pictures electrons orbiting the atomic nucleus much as planets orbit the Sun. In this model you can view hydrogen, the simplest atom, as having a single electron in a circular orbit 1.061010 m in diameter. (a) If the average speed of the electron in this orbit is known to be 2.20106 m/s, calculate the number of revolutions per second it makes about the nucleus. (b) What is the electron's average velocity?arrow_forwardYou are a coach for the Physics Olympics team participating in a competition overseas. You are given the following sample problem to present to your team of students, which you need to solve very quickly: A person is standing on the midline of a soccer field. At one end of the field, as shown in Figure P24.28, is a letter D, consisting of a semicircular metallic ring of radius R and a long straight metal rod of length 2R, the diameter of the ring. The plane of the ring is perpendicular to the ground and perpendicular to the midline of the field shown by the broken line in Figure P24.28. Because of an approaching lightning storm, the semicircular ring and the rod become charged. The ring and the rod each attain a charge Q. What is the electric potential at point P, which is at a position x along the midline of the field, measured from the center of the rod, due to the letter D? Think quickly and use all resources available to you, which include your physics textbook: yon are in competition! Figure P24.28arrow_forward
- Unreasonable Results A proton has a mass of 1.671027 kg. A physicist measures the proton's total energy to be 50.0 MeV. (a) What is the proton's kinetic energy? (b) What is unreasonable about this result? (c) Which assumptions are unreasonable or inconsistent?arrow_forwardSuppose a star 1000 times brighter than our Sun (that is, emitting 1000 times the power) suddenly goes supernova. Using data from Table 7.3: (a) By what factor does its power output increase? (b) How many times brighter than our entire Milky Way galaxy is the supernova? (c) Based on your answers, discuss whether it should be possible to observe supernovas in distant galaxies. Note that there are on the order of 1011 observable galaxies, the average brightness of which is somewhat less than our own galaxy.arrow_forwardDo Maxwells equations allow for the existence of magnetic monopoles? Explain.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781938168000
Author:Paul Peter Urone, Roger Hinrichs
Publisher:OpenStax College
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning