Answered: 6. Show that a cycle of odd length is… | bartleby

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

13th Edition

ISBN:9781133382119

Author:Swokowski

Publisher:Swokowski

Chapter10: Sequences, Series, And Probability

Section10.2: Arithmetic Sequences

Problem 51E

See similar textbooks

Related questions

Question

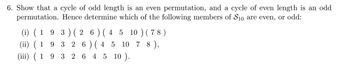
6. Show that a cycle of odd length is an even permutation, and a cycle of even length is an odd
permutation. Hence determine which of the following members of S10 are even, or odd:
(i) ( 193 ) ( 26 ) ( 4 5 10 ) (78)
(ii) ( 19326 ) ( 4
5 10 78),
(iii) ( 1932645 10).

Expert Solution

Step by step

Solved in 2 steps

SEE SOLUTION Check out a sample Q&A here

Blurred answer

Similar questions

In the expansion of (5x+3y)n , each term has the form (nk)ankbk ,where k successively takes on the value 0,1,2....,n. If (nk)=(72) what is the corresponding term?
Find the fourth term of (3a22b)11 without fully expanding the binomial.
6. Show that a cycle of odd length is an even permutation, and a cycle of even length is an odd permutation. Hence determine which of the following members of S10 are even, or odd: (i) (19 3) (2 6) (4 5 10 ) (78) 1 9 3 2 6) (4 5 10 7 8), (iii) 1 9 3 2 6 4 5 10).
Show that a cycle of odd length is an even permutation, and a cycle of even length is an odd permutation. Hence determine which of the following members of S10 are even, or odd: (i) (1 9 3) (2 6) (4 5 10) (78) (ii) (1 9 3 2 6 ) (4 5 10 7 8), (iii) ( 1 9 3 2 6 4 5 10 ).
Find all 6 permutations of (12)(345).
The permutations p = (3 5 4), q = (1 5 2 3) and r = (1 5 2) are elements of S5. (a) Find each of the following as a permutation in cycle form: wold wode ei doang poq, poqop-¹, q². (b) State the order and parity of each of po q and q². (c) Determine, in cycle form, two permutations in S5 that conjugate p to r.
If o is a k-cycle then the order of o is k. On the otherhand, if 7 is a product of disjoint cycles o1, 02, 03 .m such that the order of o; is k; then the order of 7 is given by lem(k1, k2, kg, ..., km). Find the order each of the following. (a) (1 32 4) e S4. (b) (1 4 3)(2 5 6 7) E S7. (c) (1 43 6)(2 9)(5 7 8) E Sg. (d) (1 7)(2 6)(3 5 4) o (1 2 3)(4 5 6 7) e S,
Suppose a permutation s swaps the ﬁrst two elements of {1, 2, ..., n}. A permutation r rotates the elements: r(1)=2, r(2)=3, ... r(n-1)=n, r(n)=1. We can thus write in cycle notation: s=(1 2), r=(1 2 3 4 ... n). What does the permutation rm sr -m do?
4. (i) Write the following permutations in S9 as a product of disjoint cycles 6 1 2 3 4 5 6 7 8 9 7 29 8 3 4 1 6 5 1 2 3 4 5 6 7 8 5 9 1 f = , 9 = 7 8 9 2 3 4 (ii) Calculate fg and write it both in two row notation and as a product of disjoint cycles. (iii) Calculate f-¹ and write it both in two row notation and as a product of disjoint cycles. (iv) Find the order of f, and compute f20 as a product of disjoint cycles. (v) Determine which of f, g and fg are even or odd.
Suppose α is a single cycle of length m and β is a single cycle of length n. What can be said about the order of the element αβ if α and β are disjoint cycles? If the cycles α and β share a single element in common? If the cycles α and β share two elements in common? (There are two different answers to this last question!)
(A) = and (B) D = . Find 2A - BO* none of the above
Please do Exercise 14.4.21 part B and D. Please show step by step

SEE MORE QUESTIONS

Recommended textbooks for you

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

Linear Algebra: A Modern Introduction

Linear Algebra: A Modern Introduction

Algebra

ISBN:

9781285463247

Author:

David Poole

Publisher:

Cengage Learning

Elements Of Modern Algebra

Elements Of Modern Algebra

Algebra

ISBN:

9781285463230

Author:

Gilbert, Linda, Jimmie

Publisher:

Cengage Learning,

Algebra & Trigonometry with Analytic Geometry

Algebra & Trigonometry with Analytic Geometry

Algebra

ISBN:

9781133382119

Author:

Swokowski

Publisher:

Cengage

Linear Algebra: A Modern Introduction

Linear Algebra: A Modern Introduction

Algebra

ISBN:

9781285463247

Author:

David Poole

Publisher:

Cengage Learning

Elements Of Modern Algebra

Elements Of Modern Algebra

Algebra

ISBN:

9781285463230

Author:

Gilbert, Linda, Jimmie

Publisher:

Cengage Learning,

College Algebra

College Algebra

Algebra

ISBN:

9781938168383

Author:

Jay Abramson

Publisher:

OpenStax

SEE MORE TEXTBOOKS