
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
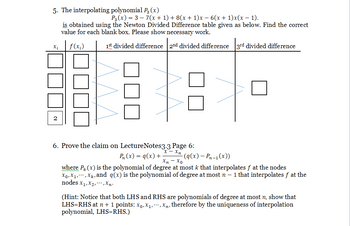
Transcribed Image Text:5. The interpolating polynomial P3 (x)
Xi
P3(x) = 37(x + 1) + 8(x + 1)x − 6(x + 1)x(x - 1).
is obtained using the Newton Divided Difference table given as below. Find the correct
value for each blank box. Please show necessary work.
f(x₂)
1st divided difference 2nd divided difference 3rd divided difference
☐☐☐
6. Prove the claim on Lecture Notes3.3 Page 6:
x -
P₂ (x) = q (x) +
Xn - Xo
where P(x) is the polynomial of degree at most k that interpolates f at the nodes
Xo, X₁, X, and q(x) is the polynomial of degree at most n - 1 that interpolates f at the
nodes x₁,x₂,.,Xn-
-(q (x) - Pn-1(x))
(Hint: Notice that both LHS and RHS are polynomials of degree at most n, show that
LHS=RHS at n + 1 points: Xo, X₁, X, therefore by the uniqueness of interpolation
polynomial, LHS=RHS.)
Expert Solution
arrow_forward
Step 1
Given : ,
To Find : Value for Box
Note : According to the guideline we are suppose to answer only one question at a time . kindly repost the remaining question and mention it .
Step by stepSolved in 2 steps

Knowledge Booster
Similar questions
- Find the root between [-5,-4] where f(x) = (x+2)' – 3x+5 with error c=0.1 by using False Position Method. Write your answer in 4 decimal plates. like: 2.1212 Answer:arrow_forwardDescribe the effect of multiplying VI by -1 as in f (x) = -/I.arrow_forwardFor what value of m is the value x=-1 a zero of the function f(x)=2x^3+mx^2−2x−1. Please show work!arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

