Question
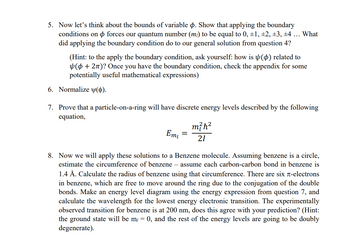
Transcribed Image Text:5. Now let's think about the bounds of variable . Show that applying the boundary
conditions on forces our quantum number (m) to be equal to 0, ±1, ±2, ±3, ±4 ... What
did applying the boundary condition do to our general solution from question 4?
(Hint: to the apply the boundary condition, ask yourself: how is (p) related to
(+2π)? Once you have the boundary condition, check the appendix for some
potentially useful mathematical expressions)
6. Normalize (d).
7. Prove that a particle-on-a-ring will have discrete energy levels described by the following
equation,
Emi
=
m²ħ²
21
8. Now we will apply these solutions to a Benzene molecule. Assuming benzene is a circle,
estimate the circumference of benzene - assume each carbon-carbon bond in benzene is
1.4 Å. Calculate the radius of benzene using that circumference. There are six л-electrons
in benzene, which are free to move around the ring due to the conjugation of the double
bonds. Make an energy level diagram using the energy expression from question 7, and
calculate the wavelength for the lowest energy electronic transition. The experimentally
observed transition for benzene is at 200 nm, does this agree with your prediction? (Hint:
the ground state will be my = 0, and the rest of the energy levels are going to be doubly
degenerate).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images

Knowledge Booster
Similar questions
- Question A3 Consider the energy eigenstates of a particle in a quantum harmonic oscillator with frequency w. a) Write down expressions for the energies of the three lowest states. b) c) Sketch the potential for this system, along with the position of the three lowest energy levels. Add to your sketch the form of the wavefunction and the probability density in the three lowest energy states. [10 marks]arrow_forwardPlease give me answers in 5min I will give you like surearrow_forward2. Consider the potential shown: -kx, x 0 2 where k is a positive constant. Call the ground state energy eigenfunction of this well ₁(x), with energy E₁. The fourth excited state would be called 45(x), with energy E5. In your answers to the questions below, please indicate in words interesting features, e.g. sign of concavity, where zeros are, where there is decay or oscillation, forbidden regions, etc. (a) Sketch the ground state, 4₁(x), (b) Sketch the excited state, 45(x) V(x) I I V(x) I x=0 E5 E₁arrow_forward
- Needs Complete typed solution with 100 % accuracy. Don't use chat gpt or ai i definitely upvote you.arrow_forward2. Quantum Mechanics Please write the solutions completely (from general formula to derivation of formula) for study purposes. Thank you. Book: Quantum Mechanics Concepts and Applications - Nouredine Zettiliarrow_forward9arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios