Question
I need a proper solution for this problem. Please give a well explained answer
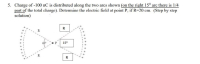
Transcribed Image Text:### Educational Resource
**Title: Determining the Electric Field at a Point Due to a Distributed Charge**
#### Problem Statement:
A charge of -100 nC is distributed along the two arcs as shown in the figure below. Note that on the right 15° arc, there is 1/4 of the total charge. Determine the electric field at point P, given that R = 20 cm. (Step-by-step solution)
#### Diagram Explanation:
The diagram consists of the following elements:
- Two arcs of circles centered at point P.
- The left arc subtending an angle of 60°.
- The right arc subtending an angle of 15°.
- Both arcs are at a distance R = 20 cm from point P.
- The total charge is -100 nC, with a quarter of this charge (-25 nC) on the 15° arc and the remainder (-75 nC) on the 60° arc.
#### Step-by-Step Solution:
1. **Understand Charge Distribution:**
- Total charge \(Q = -100 \text{ nC}\).
- Charge on 15° arc \( = \frac{1}{4} Q = -25 \text{ nC}\).
- Charge on 60° arc \( = \left(1 - \frac{1}{4}\right) Q = -75 \text{ nC}\).
2. **Determine Electric Field Contribution from Each Arc:**
- Electric field due to a charge \(dq\) at a distance \(R\) is \(dE = \frac{k_e \cdot dq}{R^2}\), where \(k_e = 8.99 \times 10^9 \text{ Nm}^2/\text{C}^2\).
3. **Calculate Linear Charge Densities:**
- Linear charge density for 15° arc, \(\lambda_1 = \frac{-25 \text{ nC}}{\text{arc length}}\).
- Linear charge density for 60° arc, \(\lambda_2 = \frac{-75 \text{ nC}}{\text{arc length}}\).
4. **Arc Length Calculation:**
- Arc length \(L = R \theta\), with \(\theta\) in radians.
- For 15°: \(\theta_1 = 15° \times \frac{\pi}{180
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- You will deal with a spherical shell -- a ball, where all the mass is on the surface. Inside is vacuum. (This is difficult to draw other than a circle.) Calculate the surface density (areal density) in g/cm2. You need these numbers: R = 27 cm M = 21,000 gramsarrow_forwardSchwarzschild radius RS of a black hole is the maximum distance from the black hole’s center at which light cannot escape its gravitational field. The quantity RS (with dimensions of length) is dependent on the mass of the black hole M, the speed of light c, and the gravitational constant G. Based on the dimensions of these four parameters, predict an equation for the Schwarzschild radius. Hint: G has dimensions of [L3/MT2]arrow_forward
arrow_back_ios
arrow_forward_ios