Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
thumb_up100%
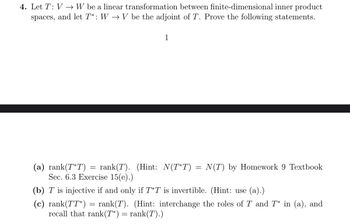
Transcribed Image Text:4. Let T: VW be a linear transformation between finite-dimensional inner product
spaces, and let T*: W→ V be the adjoint of T. Prove the following statements.
1
=
(a) rank(T*T) = rank(T). (Hint: N(T*T) =
Sec. 6.3 Exercise 15(e).)
(b) T is injective if and only if T*T is invertible. (Hint: use (a).)
(c) rank(TT*) rank(T). (Hint: interchange the roles of T and T* in (a), and
recall that rank(T*) = rank(T).)
=
N(T) by Homework 9 Textbook
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images
Knowledge Booster
Similar questions
- Please show work with steps and solution!arrow_forward3 be W be vector a positive integer, V and field F, T: VV be linear transformation, and Spaces over f: V²->w be a multilinear alternating function. Let g: V" w be the function defined by Let n a V g (V., ..., Vn ) = + (T(V₁), T(V₂), ...,T (Un)) (V.... . Vn) EV" Prove the following statements. (a g is multilinear and alternating. (b) If V=F", W=F₁, and T=LA for some A € Mnxn (F), then g= (detA/.farrow_forwardPlease help me. Fast solution Thankyou.arrow_forward
- +b] Let T : R? → R be a linear transformation. Which of the following is equal to T a +b O A. T (B) +" (!) В. T +T +T +T Oc. T 피 (B) OD. | 2Tarrow_forwardLet T: R³ R2 be a linear mapping. → 10 *([:]) = [ ] ¹ ({]) - [ ] -~-~(:)) - [²7] ([]) - T = and T find T (ED). Given that T sin (a) Ər f α Ω Earrow_forwardLet U,V ,W be finite-dimensional F-vector spaces and let T:U → V, S:V→W be linear transformations. Prove that nullity(S • T) - nullity(T ) = dim(im(T ) N ker(S)) following steps (a)-(c) below. a) LetU:=ker(S-T)andT:=T|u.Prove:im(T').=T(U')=im(T)N ker(S ). b) Prove:ker(T')=ker(T). c) Deduce:nullity(S•T)-nullity(T)=dim(im(T)nker(S)).arrow_forward
- 4. Let VCR" be a subspace. Let F: V→V and G: V → V be invertible linear transformations. Denote by F¹: V→V and G¹: V → V the inverses of F and G respectively. Is the map H: V → V defined by H(v):= F(G(F¹(G¹(v)))), for v € V, a linear transformation? Justify your answer.arrow_forwardLet f : R? → R be defined by f((x, y)) = 5x + 7y. Is f a linear transformation? a. f({x1,Yı) + (x2, Y2)) : f({x1, Y1)) + f({x2, Y2)) = + Does f((x1,Y1) + (x2, Y2)) = f(x1, Y1)) + f((x2, Y2)) for all (01, Y1), (x2, Y2) E R?arrow_forwardQ3. Let A = -1 1 2 0 3 -2 0-6 4 0 12 (a) Find the null space of A. (b) Let a linear transformation T: R → R be defined by T() = Au. Find n and m. (c) For the linear transformation T defined in part (b) find dim(Ker(T)) and dim(Im(T)).arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,