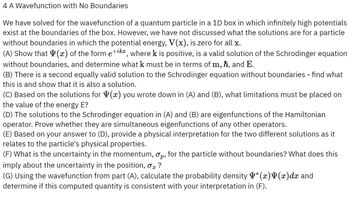
4 A Wavefunction with No Boundaries We have solved for the wavefunction of a quantum particle in a 1D box in which infinitely high potentials exist at the boundaries of the box. However, we have not discussed what the solutions are for a particle without boundaries in which the potential energy, V(x), is zero for all x. (A) Show that (x) of the form e+ikx, where k is positive, is a valid solution of the Schrodinger equation without boundaries, and determine what k must be in terms of m, ħ, and E. (B) There is a second equally valid solution to the Schrodinger equation without boundaries - find what this is and show that it is also a solution. (C) Based on the solutions for ✓ (x) you wrote down in (A) and (B), what limitations must be placed on the value of the energy E? (D) The solutions to the Schrodinger equation in (A) and (B) are eigenfunctions of the Hamiltonian operator. Prove whether they are simultaneous eigenfunctions of any other operators. (E) Based on your answer to (D), provide a physical interpretation for the two different solutions as it relates to the particle's physical properties. (F) What is the uncertainty in the momentum, σp, for the particle without boundaries? What does this imply about the uncertainty in the position, σx ? (G) Using the wavefunction from part (A), calculate the probability density Y* (x) (x)dx and determine if this computed quantity is consistent with your interpretation in (F).

Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.Very very grateful!Please do not rely too much on chatgpt, because its answer may be wrong. Please consider it carefully and give your own answer. You can borrow ideas from gpt, but please do not believe its answer.

and and Very very grateful!