Answered: 4 12 Continuous Compounding 1 365

Glencoe Algebra 1, Student Edition, 9780079039897, 0079039898, 2018

18th Edition

ISBN:9780079039897

Author:Carter

Publisher:Carter

Chapter5: Linear Inequalities

Section5.2: Solving Inequalities By Multiplication And Division

Problem 3AGP

See similar textbooks

Related questions

Question

Complete the table by determining the balance A for P dollars invested at rate r for t years and compounded n times per year P = $2500 r = 6% t = 20 years

Expert Solution

Step 1

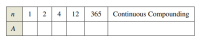
Given: P=$2500, r=6%, t=20 years

The formula for Compound Interest is:

$A = P {(1 + \frac{\frac{r}{n}}{100})}^{t n}$ , where A is the amount, P is the principal, r is the rate of interest, n is the number of times compounding is done in a year and t is the time period.

For n=1:

$\begin{array}{rcl} A & = & P {(1 + \frac{\frac{r}{n}}{100})}^{t n} \\ = & 2500 {(1 + \frac{6}{100})}^{20} \\ = & 2500 {(1.06)}^{20} \\ = & 8, 017.83 \end{array}$

Thus, when n=1 the value of the amount is $8,017.83

Step 2

For n=2:

$\begin{array}{rcl} A & = & P {(1 + \frac{\frac{r}{n}}{100})}^{t n} \\ = & 2500 {(1 + \frac{\frac{6}{2}}{100})}^{20 \times 2} \\ = & 2500 {(1 + \frac{3}{100})}^{40} \\ = & 2500 {(1.03)}^{40} \\ = & 8, 155.09 \end{array}$

Thus, when n=2 the value of the amount is $8,155.09.

For n=4:

$\begin{array}{rcl} A & = & P {(1 + \frac{\frac{r}{n}}{100})}^{t n} \\ = & 2500 {(1 + \frac{\frac{6}{4}}{100})}^{20 \times 4} \\ = & 2500 {(1 + \frac{1.5}{100})}^{80} \\ = & 2500 {(1.015)}^{80} \\ = & 8, 226.65 \end{array}$

Thus, when n=4 the value of the amount is $8,226.65.

Step by step

Solved in 4 steps

Knowledge Booster

$Transcendental Expression$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, probability and related others by exploring similar questions and additional content below.

Recommended textbooks for you

Glencoe Algebra 1, Student Edition, 9780079039897…

Algebra

ISBN:

9780079039897

Author:

Carter

Publisher:

McGraw Hill