
Calculus: Early Transcendentals
8th Edition
ISBN: 9781285741550
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question

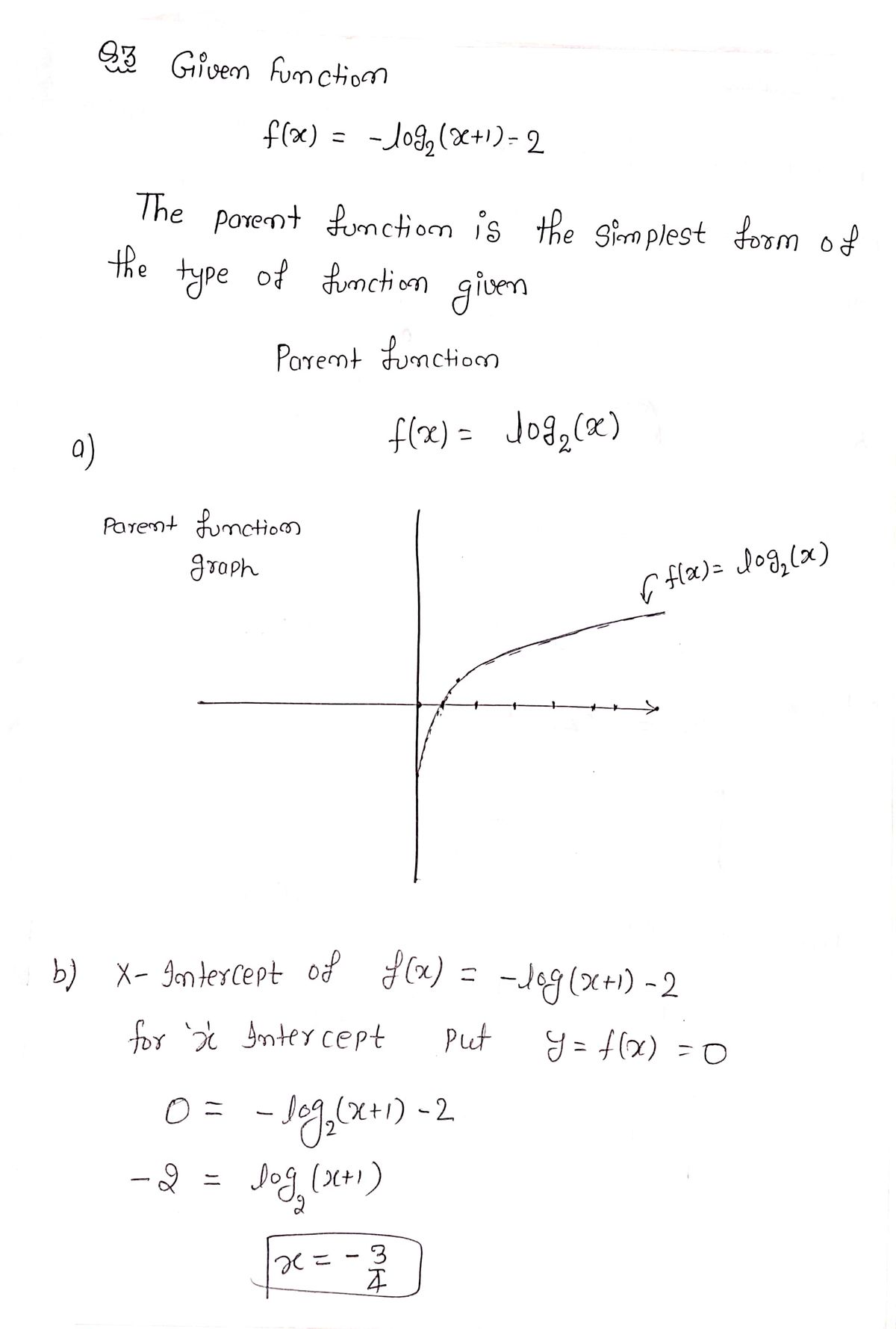
Transcribed Image Text:### Exercise: Logarithmic Function Analysis
Given the function \( f(x) = -\log_2(x + 1) - 2 \), complete the following tasks:
#### a) Determine the parent function and sketch its graph.
Parent Function: The parent function of \( f(x) = -\log_2(x + 1) - 2 \) is \( \log_2(x) \).
- **Graph Explanation:**
- The graph is a standard Cartesian coordinate system with the x-axis labeled from -10 to 10 and the y-axis labeled from -10 to 10.
- The scale is uniform, with grid lines at every unit on both axes.
- The graph should depict a transformation of the logarithmic function, involving reflection over the x-axis, a shift to the left by 1 unit, and a downward shift by 2 units.
#### b) Find the x-intercept of \( f(x) \).
- **X-Intercept:** Solve \( f(x) = 0 \) to find \( x \).
#### c) Find the y-intercept of \( f(x) \).
- **Y-Intercept:** Evaluate \( f(0) \).
#### d) Is there a vertical asymptote? If so, what is it?
- **Vertical Asymptote:** There is a vertical asymptote at \( x = -1 \) due to the transformation \( (x + 1) \).
#### e) Is there a horizontal asymptote? If so, what is it?
- **Horizontal Asymptote:** Analyze the function as \( x \to \infty \).
Note that each task should be completed with detailed calculations and graphing as needed to facilitate understanding.

Transcribed Image Text:**Educational Website Content:**
### Exercise on Function and Graph Analysis
#### Part f: Sketching the Graph of \( f(x) \)
- **Task**: Sketch the graph of \( f(x) \). Make sure to label all intercepts and asymptotes.
- **Graph Details**:
- The graph is plotted on a standard coordinate plane.
- The x-axis and y-axis are labeled from -10 to 10.
- Each axis is divided into increments of 2.
- **Instructions**:
1. Identify and label the x-intercepts and y-intercepts on the graph.
2. Determine any vertical or horizontal asymptotes and mark them clearly.
#### Part g: Analyzing Domain and Range
- **Task**: Find the domain and range of \( f(x) \).
- **Instructions**:
1. Determine the set of all possible x-values (domain) for which the function \( f(x) \) is defined.
2. Identify the set of all possible y-values (range) that the function can take.
### Tips for Solution:
- Review the characteristics of the function \( f(x) \) to determine intercepts and asymptotes.
- Use the graph to visually verify the intercepts and asymptotic behavior.
- Apply the definition of domain and range in the context of the graph and the function's equation (if provided).
Use these guidelines to complete the exercise and deepen your understanding of graphing functions and analyzing their properties.
Expert Solution
arrow_forward
Step 1
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- The function D(h) = 7 e-0.69h can be used find the number of milligrams D of a certain drug that is in a patient's bloodstream h hours after as been administered. How many milligrams will be present after 1 hour? After 8 hours? 12 After 1 hour, there will be milligrams. (Round to two decimal places as needed.) After 8 hours, there will be milligrams. (Round to two decimal places as needed.)arrow_forward25. (a) Determine whether y = 3. 7* is a linear, power, or exponential function (i.e, choose one column,and fill in the missing values in that column. Linear Function slope = Power Function coefficient = Exponential Function initial value = y-intercept = exponent = b = (b) Determine whether y = is a linear, power, or exponential function (i.e, choose one column,and fill in the missing values in that column. Exponential Function initial value Linear Function Power Function coefficient = slope = y-intercept = exponent = (c) Determine wheter y = 3- (r+10)-7x is a linear, power, or exponential function (i.e, choose one column,and fill in the missing values in that column. Linear Function slope = Power Function coefficient = Exponential Function initial value = y-intercept = exponent = b = (d) Determine wheter y = 12" · 997 is a linear, power, or exponential function (i.e, choose one column,and fill in the missing values in that column. Linear Function slope = Power Function coefficient =…arrow_forwardThe average temperature of a particular town in Alberta can be represented by the function 6 T=15.8sin((-3))+5, where T is the average temperature in degrees Celsius and t is the time in months after January 1. The minimum number of months, to the nearest tenth of a month, it takes for the average temperature of the town to rise from 5°C to 15°C is a. 1.3 months b. 3.0 months c. 2.6 months d. 4.3 monthsarrow_forward
- Determine which of the following functions matches the given graph. I- 3 A. y = (z – 3)(z + 7) I - 3 B. y = (z + 3)(z + 7) 3 C. y = I+7 3 - I OD. y= (z – 3)(x + 7)arrow_forward2. Given g(x) =- ft(* - 3)) – 5where f(x) = 2" a. Write the equation of the transformed function b. Graph the transformed function. Be sure to show all steps! c. State the domain ana range of the function -10arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning

Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning