
Managerial Economics: A Problem Solving Approach
5th Edition
ISBN: 9781337106665
Author: Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike Shor
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
Not use ai please
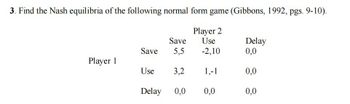
Transcribed Image Text:3. Find the Nash equilibria of the following normal form game (Gibbons, 1992, pgs. 9-10).
Player 2
Save
Use
Delay
Save
5,5
-2,10
0,0
Player 1
Use
3,2
1,-1
0,0
Delay 0,0
0,0
0,0
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps

Knowledge Booster
Similar questions
- Consider the Normal Form Game characterized in the following figure: P1 \ P2 A1 A2 A3 A4 B1 B2 B3 B4 (-1,1) (0,0) (1,-1) (2,0) (-2,-2) (2,7) (-1,-1) (-1,1) (5,7) (3,5) (0,0) (0,8) (0,3) (-1,-1) (10,2) (0,0) Which is the set of rationalizable actions? O (A2, A4)x(B2, B3) O (A1, A2)x(B1, B2, B4) O (A1, A3)x(B1, B4) O (A1, A3, A4}x{B1, B3)arrow_forwardW X Y Z 47, 15| 39, 41 45, 53 12, 56 In equilibrium, what is the probability that player 1 will use the pure strategy X in this game?arrow_forwardFind all ESS, if any, in the following symmetric game. Please check all that apply: Stop OMixed 1/4 Go and 3/4 Stop OMixed 1/2 Go and 1/2 Stop OMixed 2/3 go and 1/3 Stop Go There are no ESS Go Stop Go 2.2 5,3 Stop 3,5 2,2arrow_forward
- B block allow 5,9 3, 11 high A 6,2 4,4 low In this normal form game, there is a Nash equilibrium where A chooses high; block high; allow low; block low; allow and B choosesarrow_forwardConsider the following simultaneous move game: Player 1 U M D Player 2 L 2,10 2,5 8,7 C 5,2 11,8 8,4 R 5,4 2,9 8,3 In the Nash equilibrium of this game, player 1 receives a payoff of ✓ and player 2 receives a payoff ofarrow_forwardHi this is from a textbook. Thank you. Consider the following game in which Sally can play T or B and John chooses between L or R. Each player makes their choice simultaneously. If Sally chooses T and John chooses L, Sally gets a payoff of 5 and John has a payoff of 4. If Sally plays T and John R, Sally’s payoff is 8 and John gets 3. If Sally Chooses B and John L, the payoffs are 3 to Sally and 2 to John. Finally, if Sally chooses B and John R, the payoffs are 7 to Sally and 0 to John. Which statement is true? a) The Nash equilibrium is (B,R); this is a prisoners’ dilemma b) The Nash equilibrium is (T,R); this is a prisoners’ dilemma c) The Nash equilibrium is (T,R); this is not dominant strategy equilibrium d) The Nash equilibrium is (T,L); this is a dominant strategy equilibrium e) None of the abovearrow_forward
- Consider a sequential game where there are two players, Jake and Sydney. Jake really likes Sydney and is hoping to run in to her at a party this weekend. Sydney can't stand Jake. There are two parties going on this weekend and each player's payoffs are a function of whether they see one another at the party. The payoff matrix is as follows: Sydney Party 1 Party 2 Party 1 6, 18 18, 6 Jake Party 2 24,8 0,24 a) Does this game have a pure strategy Nash Equilibrium? b) What is the mixed strategy Nash Equilibrium? c) Now suppose Sydney decides what party she is going to first. Her roommate is friends with Jake and will call him to tell him which party they go to. Write the extensive form of this game (game tree). d) What is the subgame perfect Nash equilibrium from part c?arrow_forwardCan you help me with the question below? What is [are] the Nash Equilibrium [Equilibria] of this game? A) (10;10) and (20;20) B) (30;30) C) (10;20) and (20;10) D) (20;20) E) (30;30)arrow_forwardAa4arrow_forward
- 3. How many extensive forms are there that are equivalent to the following normal form game (pay attention to the payoffs in the matrix)? You may rename strategies, if necessary. Show the extensive forms. II ti t2 t3 t4 ts to si 7,1 2,2 | 1,5 7,1 I 2,2 1,5 S2 3,3 3,3 | 3,3 2,1 2,1 2,1arrow_forwardUp ооооо Down Player 1 Up No EFFICIENT equilibrium exists Down Up Down Player 2 P1 gets $120 P2 gets $5 P1 gets $100 P2 gets $10 P1 gets $95 P2 gets $95 In the game above, what is/are the EFFICIENT sub-game perfect Nash equilibrium? (up,up) (up,down) (down, up) (down, down) P1 gets $15 P2 gets $25arrow_forwardThe following table contains the possible actions and payoffs of players 1 and 2. Player 1 U M D L 2,10 2,5 8,7 Player 2 C 5,2 11,8 8,4 R 5,4 2,9 8,3 This is a simultaneous move game. In a pure strategy Nash equilibrium of this game, Player 1 receives a payoff of ✓and player 2 receives a payoff of If, instead of playing simultaneously, player 2 moves first, then in the Nash equilibrium player 1 receives a payoff of ✓and player 2 receives a payoff ofarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Managerial Economics: A Problem Solving ApproachEconomicsISBN:9781337106665Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike ShorPublisher:Cengage Learning
Managerial Economics: A Problem Solving ApproachEconomicsISBN:9781337106665Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike ShorPublisher:Cengage Learning

Managerial Economics: A Problem Solving Approach
Economics
ISBN:9781337106665
Author:Luke M. Froeb, Brian T. McCann, Michael R. Ward, Mike Shor
Publisher:Cengage Learning