3. A thick spherical shell centered at the origin has inner radius a and outer radius 2a carries, and volume charge density p= kr (a 2a.
3. A thick spherical shell centered at the origin has inner radius a and outer radius 2a carries, and volume charge density p= kr (a 2a.
Related questions
Question
Please answer question 3, and, if possible question 4. These problems deal with the same system, so I hope this isn't too much to ask. Thank you

Transcribed Image Text:3. A thick spherical shell centered at the origin has inner radius a and outer radius 2a carries, and
volume charge density p=kr (a<r< 2a), where k is a constant. In addition there is a negative
point charge -q at the origin. Using Gauss law, calculate the electric field at radii a < r < 2a and
r> 2a.
4. Consider the same system as in problem 3 above, except that now take the charge density to be
constant such that the total charge in the spherical shell is given by +q. In that case, calculate the
electric potential at radius r for 0 <r <a. (Hint: Consider the line integral of the electric field in
three pieces, as = √²ª + √₂²a+Sa).
√∞
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution!
Trending now
This is a popular solution!
Step by step
Solved in 3 steps with 1 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
Are you able to now answer question 4?
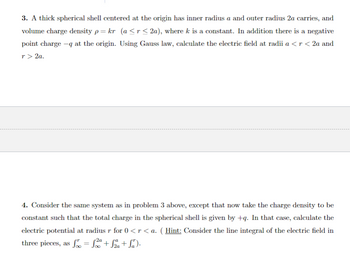
Transcribed Image Text:3. A thick spherical shell centered at the origin has inner radius a and outer radius 2a carries, and
volume charge density p=kr (a<r< 2a), where k is a constant. In addition there is a negative
point charge -q at the origin. Using Gauss law, calculate the electric field at radii a < r < 2a and
r> 2a.
4. Consider the same system as in problem 3 above, except that now take the charge density to be
constant such that the total charge in the spherical shell is given by +q. In that case, calculate the
electric potential at radius r for 0 <r<a. (Hint: Consider the line integral of the electric field in
three pieces, as = √²ª + √₂²a+Sa).
√∞
Solution