
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
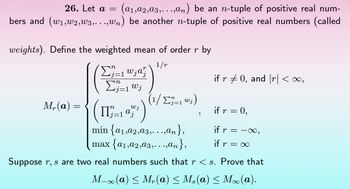
Transcribed Image Text:26. Let a =
(a₁,a2,a3,...,an) be an n-tuple of positive real num-
bers and (w₁,w2,w3,...,wn) be another n-tuple of positive real numbers (called
weights). Define the weighted mean of order r by
1/r
M₁(a) =
Σ=1 w;a;
n
Σj=1 Wj
(I_17)
j=1
(1/Σ₁ ₁²₁)
if r ‡ 0, and \r\ <∞,
if r = 0,
if r = -∞,
if r = ∞
min {a1,92,93,...,an},
max {a1,02,03,...,α₂},
Suppose r, s are two real numbers such that r < s. Prove that
M-∞(a) ≤ Mr(a) ≤ M₁(a) ≤ M∞(a).
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
- 9.K Let X = Q y K = {p € Q : 2 < p? < 3} . Show that K is closed in Q, is bounded, and is not compact in Q.arrow_forwardThe Real Numbers *7. Let S be a nonempty bounded subset of R and let ke R. Define kS = {ks :se S}. Prove the following: (a) If k20, then sup (kS) = k · sup S and inf (kS) =k · inf S. (b) Ifk 0, then there exists ne N such that n-1< y < n. ☆ (b) Prove that the n in part (a) is unique. 10. (a) Prove: If x and y are real numbers with x < y, then there are infinitely many rational numbers in the interval [x, y]. (b) Repeat part (a) for irrational numbers. 11. Let y be a positive real number. Prove that for every n e N there exists a I unique positive real number x such that x" = y. ☆ *12. Let D be a nonempty set and suppose that f: D → R and g: D → R. Define the function f +g:D→R by (f+g)(x)= f(x) +g(x). (a) If f(D) and g(D) are bounded above, then prove that (f + g)(D) is bounded above and sup[(ƒ +g)(D)] < sup f(D) + sup g(D). (b) Find an example to show that a strict inequality in part (a) may occur. (c) State and prove the analog of part (a) for infima. 13. Let x e R. Prove that x = sup…arrow_forward5. Does f(x) = In(x) satisfy the hypotheses of the Mean Value Theorem on [1,4]? If it satisfies the hypotheses, find all numbers c that satisfy the conclusion of the Mean Value Theorem.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

