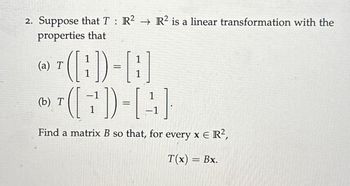
2. Suppose that T R² R2 is a linear transformation with the:properties that(a) T1(b) T(7)-[(4)Find a matrix B so that, for every x € R²,T(x) = Bx.

Answered: Image /qna-images/answer/ffad9913-44b9-484b-84a0-8601dafa0bff.jpg

Answered: 2. Suppose that T R² R² is a linear…

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

Consider a linear transformation T from R³ to R2 for which 0 3 6 T ([]) - · ~ ([1]) - · · (8) - C] = [{}], T = 8 2 T 0 = 5 Find the matrix A of T. A = =
Consider a linear transformation T from R³ to R² for which Find the matrix A of T. +46 A = T (B)-· *(B)-· *(8) - A T T
7. Let T: R³ R³ be defined by (a) (b) (E) x1 + x2 + x3 = X1 X2 X3 [X1X2 X3] Find the matrix of linear transformation for T. T Find all vectors 7 € R³ such that T(x) = x.
Let 9 2 A 0 7 and B = Find the matrix C of the linear transformation T(x) = B(A(x)). C =
Find the matrix of the linear transformation T from R2 - R² where T([1, 0]) = [1, –2] and T([2, 1]) = [2, 3] %3D (Note: matrix A = [T([1, 0]), T([0, 1])] 1 -2 (a) 1 (b) 1 (c) -2 (d) -2 3 7 3 Ob a C
-29 If T:R- R'is a linear transformation such that T 10 and 7 then the matrix that represents T is -35
5. Find the standard matrix of the given linear transformation: (a) P: R? - R? projects a vector onto the line y = -2x. For the vector u = find P(ü). (b) Rg: R3 - R rotates a vector clockwise about the z - axis through the angle 30°. For the 21 vector u = 2| find R9 (ũ). 6. For the following linear transformations find S • T and T • S. Indicate the domain and codomain of the new linear transformations. x+ y+z] x-y-zl 2x + 7. Find the inverse of the following linear transformation: [3x + 2y] [5x + 3yl %3D
Find the matrix M of the linear transformation T: R² → R² given by T ([21]) - [-5²₁ + (-7) ²1]. = -5x1-x₂ M:
Suppose T is a linear transformation on R² (i.e. T(x, y) = (ax + by, cx + dy) where a, b, c, d are real numbers and, interpreting T as a matrix, det(T) # 0. Let P be the parallelogram which is the image of the square D = = [0, 1] × [0, 1] (i.e. T(D) =P). Then the area of P is given by O lad - bcl ad bc abcd 1 |ad - bc| O labcd|
Let T : R³ → R³ be the linear transformation that does the following things, in this order, to an input vector x = [x y z]¹: (i) Interchanges the second and third coordinates of . (ii) Multiplies the first coordinate of the resulting vector by 2. (iii) Replaces the second coordinate of the resulting vector with a 0. (iv) Multiplies the resulting vector by the following matrix: 0 0 0 0 You don't have to show that T is linear. (a) The description of T given above is purely algebraic, in that it explicitly describes how to take 7 = [x y z] and write down T() in coordinates. Give a geometric description of what each of the four "steps" of applying T actually does to a vector. (Your Week 9 tutorials may help in describing what the last step does.) (b) Find the standard matrix AT of T. (c) Find a spanning set for null(AT), and describe what null(AT) is geometrically (i.e., describe it geomet- rically as a subset of R³) (d) Find a spanning set for im(AT), and describe what im(AȚ) is…
Suppose T: R³-R² is a linear transformation. Let U, V and W be the vectors given below, and suppose that T(U) and T(V) are as given. Find T(W). -3 3 2 3 ਪ = -3 -1 0 T(W) = 0 0 V = -3 W = | 3 - 1 T(I) = 0 T(V) = 0 -3
Consider a linear transformation To R₂ [x] →> R²³² whose matrix relative to the bases B = {X², X, I is MT (B, C) = 0 2 and C= {(0), (6), (8)} 2 1) Find a formula for T. That is, what is T(a+bx+cx²) ?

SEE MORE QUESTIONS

Recommended textbooks for you

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Mathematics For Machine Technology

Advanced Math

ISBN:

9781337798310

Author:

Peterson, John.

Publisher:

Cengage Learning,

Basic Technical Mathematics

Advanced Math

ISBN:

9780134437705

Author:

Washington

Publisher:

PEARSON

Topology

Advanced Math

ISBN:

9780134689517

Author:

Munkres, James R.

Publisher:

Pearson,

SEE MORE TEXTBOOKS

2. Suppose that T R² R² is a linear transformation with the : properties that (3)-B] (7)-[(4) D = 1 Find a matrix B so that, for every x E R², T(x) = Bx. (a) T (b) T