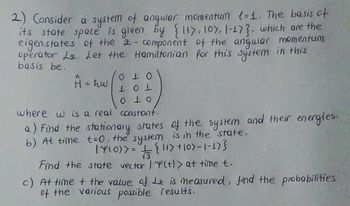
2) Consider a system of angular momentum 1=1. The basis of its state space is given by {117, 107, 1-17}, which are the eigen states of the component of the angular momentum operator L. Let the Hamiltonian for this system in this basis be. ^ Ĥ = ħw 010 101 010 where w is a real constant. a) Find the stationary states of the system and their energies. state

Suppose that you have the Lagrangian L = (;2 + ʻr²) + 20 for a 2D 20 system in plane polar coordinates (r, 0). Determine the Hamiltonian.

A massless spring with equilibrium length d and spring constant k connects two particles. The system is flat and horizontal, yet it may spin and vibrate (ccompress\stretch).1- Determine the system's Lagrangian.2- Determine the system's Hamiltonian.3- Calculate Hamilton's equations of motion. It should be noted that the generalized momenta can be omitted. -It is worth noting that as the mass spins, it begins to expand. Hint: make your coordinate system's origin the center of the unstretched spring.  also In generalized coordinates of (r_i) and (theta_i) , express your equations.

Theoretical Mechanics Topic: Lagrangian and Hamiltonian Dynamics >Generate the necessary equations to this system. > Use the equations of motion >Generate equations for (x,y), (Vx,Vy), V², T > L = T-U --- For study purposes. Thank you!