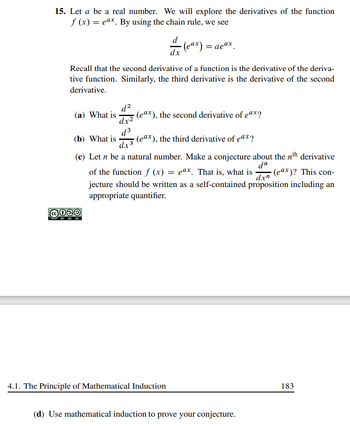
15. Let a be a real number. We will explore the derivatives of the function f(x) = eax. By using the chain rule, we see (a) What is Recall that the second derivative of a function is the derivative of the deriva- tive function. Similarly, the third derivative is the derivative of the second derivative. (b) What is d² dx² d3 C030 BY NO SA d dx = aeax (eax), the third derivative of eax? (c) Let n be a natural number. Make a conjecture about the nth derivative dn of the function f (x) = eax. That is, what is (eax)? This con- dxn jecture should be written as a self-contained proposition including an appropriate quantifier. (eax), the second derivative of eax? 4.1. The Principle of Mathematical Induction (d) Use mathematical induction to prove your conjecture. 183

Q: The Chain Rule for second derivativesa. Derive a formula for the second derivative,b. Use the…

A:

Q: 5) Chapter 3.3 Find the second derivative of f and discuss the concavity of its graph. a. f (x) =…

A:

Q: If F is a derivative function, obtain the derivative of the function u(x) = (F(x))² in terms of F…

A: given u(x)=4x2-12u(x)=F(x)2to find derivative of u(x)

Q: (c) Use the Composite Simpson's rule with N = 4 to approximate the integral in part (b) and compare…

A: The formula for composite Simpsons rule in our problem is h3f(x0)+4f(x1)+f(x3)+2f(x2)+f(x4)

Q: 11) find the derivative of the function.

A:

Q: In (4xy) + e?xy + sen (2y) = 3x* – 7xy³

A: Differentiate wrt x

Q: |(9-x)³ x² – 2 9. f(x) = In -

A:

Q: Find the derivative of the function 54² (4²+4)³ 3 g'(u) = g(u) =

A:

Q: Write True if the given derivative of the function is correct. If it is false, write the correct…

A: Dear student, Please verify and check that my answer matches with the answer given in your question.…

Q: (18) Find the derivative of the functio 2²+5 3 Y=2²-5 Nor me it to Vitou inst

A:

Q: Find the second derivative of the function. 8. f(x) = 4x3/2 9. g(x) = x-1 10. h(x) = sinx

A: NOTE: Refresh your page if you can't see any equations. . take derivative

Q: Write a derivative formula for the function fx)- 12.2(4.7 x2 1.5475x(4.7-2( 4.7*) f'(x) 12.2 = X

A: Given

Q: At what value of x does the function f(x)=x' -3r? -9x change from deceasing to increasing? 1 -1 Find…

A:

Q: Can you help me step by step with this problem?

A: Let's assume u = 3 + xsecxPlease note that the second term is made up of two functions of x and they…

Q: Find the derivative of the function. f(x) = (2x3)4(x² + x + 1)5 :- 3) ³ ( x² + x +: f'(x) = (2x-…

A:

Q: Question 19. Obtain the second derivative of the function f(x) = -5x? + 2x5 + 4. Enter your answer…

A: Topic:- derivatives

Q: 19.What is the derivative with respect to a of f(x) = 4-32 3z+2 4x-3

A:

Q: 2.2 Determine which function is the derivative of the other. Explain your answer. y = f(x) y = g(x)

A:

Q: vative of y with respect to x, the Chain Rule will be required and produce a factor that dx late.…

A: As you have mentioned