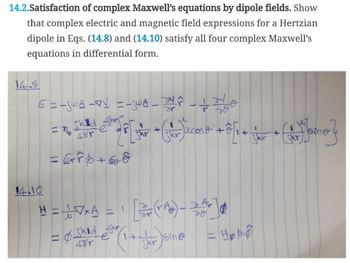
14.2.Satisfaction of complex Maxwell's equations by dipole fields. Show that complex electric and magnetic field expressions for a Hertzian dipole in Eqs. (14.8) and (14.10) satisfy all four complex Maxwell's equations in differential form.

4. Derive the maxwell equations for U, H, G and A Table 6.2.1: Maxwell Relations Function U H A G Differential dU = TdS-pdV dH = TdS + Vdp dA=-pdV - SdT dG = Vdp - SdT Natural Variables S, V S, P V, T P, T Maxwell Relation (or)--( др as V (37), = (35), as (OP), - (OV), = V (3r), - - (35), = T

Class: Electromagnetics Consider a fixed hollow spherical shell with radius R and surface charge +? (sigma). A particle with mass m and charge -Q that is initially at rest falls in from infinity. Assume that a tiny hole has been cut in the shell to let the charge through. Determine the speed of the particle when it reaches the center of the shell. Your answer should be a function of ? (sigma), Q, and m.