a) Find the total electric force exerted by the charges in the hexagon on the charge at the center
b) Determine the magnitude of the electric field in the center of the hexagon
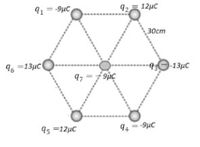
Given: The charge is .
The charge is .
The charge is .
The charge is .
The charge is .
The charge is .
The charge is .
The distance between any of the two charges is 30 cm.
To determine: (a) Total electric force exerted by all the charges on the charge at center.
Like charges repel each other and unlike charges attract each other.
The force between the two charges is given as
where F is the force between the two charges, are the two charges and r is the distance between the two charges.
The direction of force due to charge on is repulsive, it will along the charge . Similarly the direction of force due to charge on is repulsive and will be along . These two forces will cancel out each other.
Step by stepSolved in 4 steps with 1 images

- A charge q1 equal to 0.500 μC is at the origin, and a second charge q2 equal to 0.800 μC is on the x axis at 4.00 cm. Find the force (magnitude and direction) that 0.500-μC charge exerts on 0.800-μC charge. Enter a positive value if the force acts in the +x direction and a negative value if the force acts in the -x direction. (Express your answer to three significant figures.) Find the force (magnitude and direction) that 0.800-μC charge exerts on 0.500-μC charge. Enter a positive value if the force acts in the +x direction and a negative value if the force acts in the -x direction. (Express your answer to three significant figures.) How would your answers change if q2q2 were -0.800 μC? The magnitudes of the forces would rise, while the directions would be the same. The magnitudes of the forces would rise and the directions would change to opposite. The directions of the forces would change to opposite, while the magnitudes would be the same Answers would be the same.arrow_forwardA positively charged particle Q1 = +25 nC is held fixed at the origin. A second charge Q2 of mass m = 6.5 μg is floating a distance d = 35 cm above charge Q1. The net force on Q2 is equal to zero. You may assume this system is close to the surface of the Earth. a)Which of the following represents the free-body diagram of charge Q2? b)What is the sign of the charge on Q2? c)Write an equation for the magnitude of charge Q2 in terms of the given variables.arrow_forwardQuestion 1 A positively charged particle q= 12 µC is placed between two infinite charged sheets with surface charge density +a and-a as shown in the figure. If aj-3.54 µC/m², then the magnitude of the electric force (in N) on the particle is: 0.0 90 ON 48 0.00 0672arrow_forward
- 1-Dimensional forces Three fixed particles exist in a straight line, The first particle is of charge 5.0 10-6 C. The second particle is of charge -5.0 10-6 C and located 20.0 cm to the right of the first charge. The Third particle is of charge -7.5-10-6 C and located 15.0 cm to the left of the first charge. (a) What force do they exert on a fourth particle of charge -2.5 10-6 C that is 13cm to the right of the first charge? (Include magnitude and direction) (b) What is the force of the fourth particle is instead it had a charge of 3.12-10-C and is still located at 13cm to the right of the first charge? (Include magnitude and direction) (a) (b) jouarrow_forwarda K 6.0 cm 15.0 cm 1 a 10.0 cm 15.0 cm b 5.0 cm 42 Two point charges q1 and q2 of -300 nC and 300 nC, respectively, are separated by a distance of 0.15 m. Calculate the electric field caused by q1, the field caused by q2, and the total field. a) at point a Eq₁ = b) at point c Eq₁ = Eq₂=_ , Eq₂=. _ET=_ _E₁=arrow_forwardA long, thin wire along the x-axis has a linear charge density of 8.8e-6 C/m. a) Find the magnitude of the electric field at point p a distance of 0.8 m from the wire. b) Find the magnitude of the net force on a test charge of 8e-6 C at point p. c) Which direction will the test charge accelerate? parallel to wire perpendicular to and toward wire perpendicular to and away from wirearrow_forward