
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Concept explainers
Topic Video
Question
Part a from #12
Transcribed Image Text:Thomas W. Hungerford - Abstrac x
+
O File | C:/Users/angel/Downloads/Thomas%20W.%20Hungerford%20-%20Abstract%20Algebra_%20AN%20lntroduction-Cengage%20Learning%20(201..
...
Flash Player will no longer be supported after December 2020.
Turn off
Learn more
of 621
+
-- A' Read aloud
V Draw
F Highlight
O Erase
89
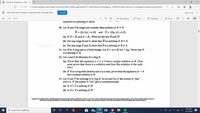
numbers is a subring of M(R).
10. Let R and S be rings and consider these subsets of R X S:
R= {(r, Os) |rER} and S= {(OR, s) | SES}.
%3D
(a) If R= Z3 and S = Zs. What are the sets R and S?
(b) For any rings Rand S, show that Ris a subring of RX S.
Goun at
(c) For any rings R and S, show that S is a subring of RX S.
11. Let R be a ring and ma fixed integer. Let S = {rɛR|mr =
is a subring of R.
OR}. Prove that S
12. Let a and b be elements of a ring R.
(a) Prove that the equation a + x = b has a unique solution in R. (You
must prove that there is a solution and that this solution is the only
one.)
(b) If R is a ring with identity and a is a unit, prove that the equation ax = b
has a unique solution in R.
13. Let Sand T be subrings of a ring R. In (a) and (b), if the answer is "yes,"
prove it. If the answer is “no," give a counterexample.
(a) Is Sn Ta subring of R?
(b) Is SU Ta subring of R?
Copyrigt 2012Ca ng AN Righ Ra nd May aot be apied ed ar dapticd is whele ar ta part D to dearonie cig, tird pary eate mey be ned fon te eBoak darehagerta). Bd N ba
ned ttoy apped a d dly dea be ovd ing apria Cagge Loaing a eright to ove dddonnl a ty timef D dghts cetti nt
9:06 AM
O Search for anything
EPIC
Ai
EPIC
O 4)
34
12/7/2020
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 2 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

