
Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Question
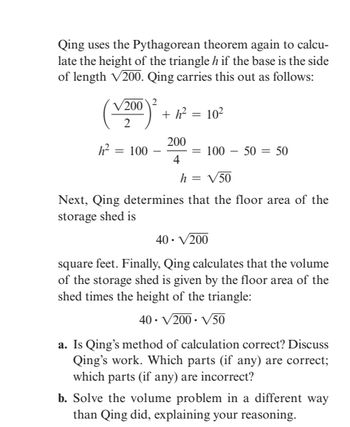
Transcribed Image Text:Qing uses the Pythagorean theorem again to calcu-
late the height of the triangle h if the base is the side
of length √200. Qing carries this out as follows:
200 2
2
h² = 100
+ h² = 10²
200
4
= 100 50 = 50
h = √50
Next, Qing determines that the floor area of the
storage shed is
40. √/200
square feet. Finally, Qing calculates that the volume
of the storage shed is given by the floor area of the
shed times the height of the triangle:
40. √/200-√/50
a. Is Qing's method of calculation correct? Discuss
Qing's work. Which parts (if any) are correct;
which parts (if any) are incorrect?
b. Solve the volume problem in a different way
than Qing did, explaining your reasoning.
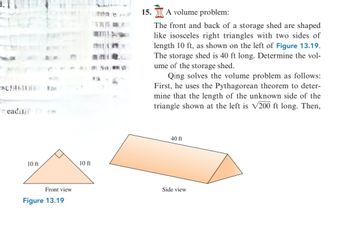
Transcribed Image Text:reading m
10 ft
Front view
Figure 13.19
10 ft
man e ju
1111 89 H
15. A volume problem:
The front and back of a storage shed are shaped
like isosceles right triangles with two sides of
length 10 ft, as shown on the left of Figure 13.19.
The storage shed is 40 ft long. Determine the vol-
ume of the storage shed.
Qing solves the volume problem as follows:
First, he uses the Pythagorean theorem to deter-
mine that the length of the unknown side of the
triangle shown at the left is √200 ft long. Then,
40 ft
Side view
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- 3 A can is in the shape of a right circular cylinder with an inner diameter of 7.5 centimeters and an inner height of 12.5 centimeters. The can is placed on its circular base, and 440 milliliters of juice is poured into the can. Given that 1 milliliter is equivalent to 1 cubic centimeter, what is the height of the juice in the can to the nearest tenth of a centimeter?arrow_forwardQuestion 10 II Pause Q Zoom Tools- /Question 10 A company makes an ice-cream treat in the shape of a hemisphere on top of a cone, as shown below. 2 cm 10 cm What is the volume of the ice-cream treat? 192 T cm T cm T cm T om 2021 lluminate Education Inc. Type here to searcharrow_forward1. A water tank is shaped fike a cylinder, It is 20 m tall and has a radius of 7 m. How many cubic meters of water can the tank hold? Round your answer to the nearest whole number. Asked: Given: Formula: Solution: Answer:arrow_forward
- Solve these problems. When necessary, round the answers to 2 decimal places unless otherwise specified Compute the height of a regular pyramid with a base area of 32.00 square feet and a volume of 152.0 cubic feet. A right circular cone 1.2 meters high contains 0.80 cubic meters of material. Find the cone base area. The base area of a wooden form in the shape of a regular pyramid is 28.0 square feet. The form contains 21.0 cubic feet of airspace. How high is the form? A container with a capacity of 6.0 gallons is in the shape of a right circular cone. The container is 1.5 feet high. Find the container base area. A tent in the shape of a regular pyramid is designed to contain 5.60 cubic meters of airspace. The base of the tent is square with each side 2.50 meters long. What is the height of the tent?arrow_forwardI have an area composite figures geometry problem.arrow_forwardcalculate the following problem and show your complete solutionarrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning