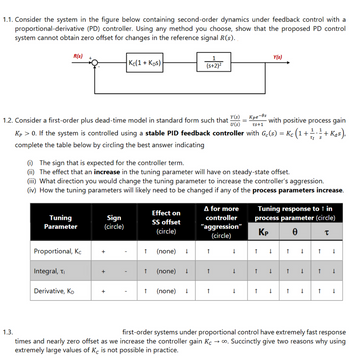
1.1. Consider the system in the figure below containing second-order dynamics under feedback control with a proportional-derivative (PD) controller. Using any method you choose, show that the proposed PD control system cannot obtain zero offset for changes in the reference signal R(s).. R(s) Kc(1+ KDS) 1 (s+2)² Y(s) 1.2. Consider a first-order plus dead-time model in standard form such that Y(s) Kpe-0s U(s) TS+1 with positive process gain 1.3. Kp > 0. If the system is controlled using a stable PID feedback controller with G₁(s) = Kc (1 + ±1. 1½ + Kas), complete the table below by circling the best answer indicating (i) The sign that is expected for the controller term. (ii) The effect that an increase in the tuning parameter will have on steady-state offset. TI S (iii) What direction you would change the tuning parameter to increase the controller's aggression. (iv) How the tuning parameters will likely need to be changed if any of the process parameters increase. Tuning response to ↑ in process parameter (circle) Tuning Parameter Sign (circle) Effect on SS offset A for more controller "aggression" (circle) (circle) KP 0 τ Proportional, Kc + ↑ (none) ↓ ↑ ↓ ↑ ↓ ↑ ↓ ↑ ↓ Integral, ti ↑ (none) ↓ ↑ ↓ ↑ ↓ ↑ ↓ ↑ ↓ Derivative, KD + ↑ (none) ↓ ↑ ↓ ↑ ↓ ↑ ↓ ↑ ↓ first-order systems under proportional control have extremely fast response times and nearly zero offset as we increase the controller gain Kc → ∞. Succinctly give two reasons why using extremely large values of Kε is not possible in practice.

(a) An audio amplifier is to be designed to develop a 50-V peak-to-peak sinusoidal signal at a frequency of 20 kHz. What is the slew-rate specification of the amplifier? (b) Repeat for a frequency of 20 Hz.

For the model defined in Question , is the closed loop system with the negative feedback control law?     a. unstable b.asymptotically stable c.not unstable d.not asymptotically stable

I need the answer as soon as possible

6. Consider the feedback system shown in Figure 5. The process transfer function is marginally stable, The controller is the proportional-derivative (PD) controller G,(s) = K, +Kps. Determine if it is possible to find values of K, and K, such that the closed-loop system is stable. If so, obtain values of the controller parameters such that the steady-state tracking error E(s) = R(s)-Y(s) to a unit step input R(s) =1/s is e, = lim e(t) <0.1 and the damping of the closed-loop system is 5=V2/2. Controller Process E,(s) 4 R(s) Kp+Kps Y(s) +4 erwe Figure 5

Pls solve in detail by hand.

Electrical Engineering Major. Course: EE350 ( System Dynamics and control ).

1. The DC-DC converter has several circuit configurations. a) (Draw a simple connection of IGBT switch, diode and inductor that can discriminate between Buck, Boost and Buck-Boost converter circuits respectively.) b) ('A Boost converter produces an output voltage, Vo that is more than or equal to the input voltage, Vi. Based on this statement develop the equation for the relationship between Vo Viand D. where D represents the duty ratio. You can use any suitable diagram to illustrate your answer.)

Feedback improves Ex. 220. Refer to Figure 220. disturbance rejection. Given: R=0, controller gain K=50, and a unit step disturbance, (i.e. D(s) = 1/s), determine the steady state output, Css, when: A) the loop is closed with H=1, and B) the loop is open with H=0. ans:2

QUESTION4 Consider the feedback system in the figure with G(s) Using the Ziegler-Nichols Oscillation Method. %3D (s+1)(s+3)(s+10) for an ideal PID controller C(s) - K, ++ Kps determine the gains K K, and K. Choose the closest set of gains. C(s) G(s) OK, - 18, K, = 12.81, K, = 6.32 O Kp = 4.80, K, = 2.65, Kp = 2.18 O Kp - 47.52, K, = 85.57, K, - 12.60 %3D O K, - 68.64, K, = 143.27, Kp = 8.22