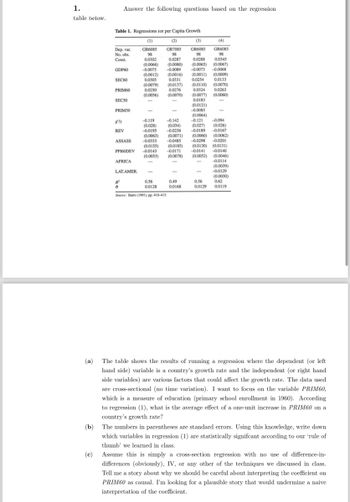
1. table below. Answer the following questions based on the regression Table 1. Regressions for per Capita Growth (1) (2) (3) (4) Dep. var. GR6085 GR7085 GR6085 GR6085 No. obs. 98 98 98 98 Const. 0.0302 0.0287 0.0288 0.0345 (0.0066) (0.0080) (0.0065) (0.0067) GDP60 -0.0075 -0.0089 -0.0073 -0.0068 (0.0012) (0.0016) (0.0011) (0.0009) SEC60 PRIM60 0.0305 (0.0079) 0.0250 (0.0056) 0.0331 0.0254 0.0133 (0.0137) (0.0110) (0.0070) 0.0276 0.0324 0.0263 (0.0070) (0.0077) (0.0060) SEC50 0.0183 (0.0121) PRIM50 -0.0085 (0.0064) gly -0.119 -0.142 -0.121 -0.094 (0.028) (0.034) (0.027) (0.026) REV -0.0195 -0.0236 -0.0189 -0.0167 (0.0063) (0.0071) (0.0060) (0.0062) ASSASS -0.0333 -0.0485 -0.0298 -0.0201 (0.0155) (0.0185) (0.0130) (0.0131) PP160DEV -0.0143 -0.0171 -0.0141 -0.0140 (0.0053) (0.0078) (0.0052) (0.0046) AFRICA -0.0114 (0.0039) LAT.AMER. -0.0129 (0.0030) 0.56 0.0128 0.49 0.0168 0.56 0.62 0.0129 0.0119 Source: Barro (1991), pp. 410-413. (a) The table shows the results of running a regression where the dependent (or left hand side) variable is a country's growth rate and the independent (or right hand side variables) are various factors that could affect the growth rate. The data used are cross-sectional (no time variation). I want to focus on the variable PRIM60, which is a measure of education (primary school enrollment in 1960). According to regression (1), what is the average effect of a one-unit increase in PRIM60 on a country's growth rate? (b) The numbers in parentheses are standard errors. Using this knowledge, write down which variables in regression (1) are statistically signifcant according to our 'rule of thumb' we learned in class. (c) Assume this is simply a cross-section regression with no use of difference-in- differences (obviously), IV, or any other of the techniques we discussed in class. Tell me a story about why we should be careful about interpreting the coefficient on PRIM60 as causal. I'm looking for a plausible story that would undermine a naive interpretation of the coefficient.

A: Revenue: The revenue is the income earned by a producer from the sales of goods and services.

A: Underground economy is the economic activities which are beyond the reach of tax regulators and…

A: Data refers to individual facts, figures, or bits of information that are often quantitative. A…

A: Discussing the "matter" of economics involves exploring its fundamental components and core…

A: Answer: Ideology: Ideology refers to the set of ideas, beliefs, manner of thinking, etc. of a person…

A: Infrastructure is organisational structure which is required for the societal operation.

A: The systematic examination and assessment of economic phenomena, such as the creation, exchange, and…

A: When distributors receive 55 million, the value captured of 330 million can be understood through…

A: People are perplexed when they go car shopping because they believe they are purchasing a vehicle…

A: A supervisor is a person who is responsible for supervising an activity or business. He is…

A: Unemployment is an expression alluding to people who are employable and effectively looking for a…

A: The court is the place where trials and legal cases take place.