
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
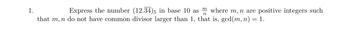
Transcribed Image Text:1.
n
Express the number (12.34)5 in base 10 as m where m, n are positive integers such
that m, n do not have common divisor larger than 1, that is, gcd(m, n) = 1.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps

Knowledge Booster
Similar questions
- Let 1Sasb be two positive integers. How can I prove or disprove that there exists a 1 sksb-1 such that ak=1 (mod b)arrow_forwardhow would you prove thisarrow_forward(5) (Extra credit, 5 points) Show that 102n-1 +1 is divisible by 11 (namely, 102n−1 +1 = 11 ·an, where a₁ = 1 and an+1 = 100an 9 for all natural numbers n = 1, 2, ...). [Hint: Show that 102n+1+1=100 (102n−1 + 1) - 99.]arrow_forward
- Prove that 52N-1 is divisible by 8 for all NEN 5²-1 - 8 2(1) 1) Show for N=1 5203-1-N 8 52h-Y8 3) Apply K+1: 52 (H+1)_1 = 2) Assume K: · L = N 1=3 yes, divisiblearrow_forwardUse the factorization A = PDP-1 to compute Ak, where k represents an arbitrary positive integer. 2-142 1³:1 = 01 AK = - 15 72 -4 19 49 30 -2 9 1 -4arrow_forwardLet x be a natural number with a binary representation where the difference between the number of 1 bits with an even index and the number of 1 bits with an odd index is a multiple of 3. Prove that x is a multiple of 3.arrow_forward
- Define the fibonacci numbers by f(0) = 0; f(1) = 1; and then recursively for natural numbers n ≥ 2 by f(n) = f(n-1) + f(n-2). a) Write out the first 7 fibonacci numbers. I b) Make a conjecture about which numbers n will give us that f(n) will be an even number. c) Prove an exact characterization of these numbers using strong induction. (i.e. show that if your characterization is true up to some point, then it would be true just after that)arrow_forwardExpand (x+4) using Pascal's triangle. 1. What is the correct row of numbers to use from Pascal's triangle? A) 1, 3, 3, 1 B) 1, 4, 6, 4, 1 C) 1, 3, 6, 3, 1 D) 1, 2, 1 2. Which of the following shows the correct expression to expand the binomial? A) 1x'(4)* +2x²(4)² +1x*(4}° B) 1x°(4)° +3x'(4)* +3x*(4}* +1x°(4}³ C) 1x°(4)° +3x²(4)* +3x*(4)° + ix°{4}³ D) 1x'(4)° +(3x}(4)' +(3x)*(4)* +1x°(4)³ 3. Write the expansion in simplified form. A) x³ +36x² +144x+64 B) 1+12x+48x² +64x³ C) x +36x? + 48x +64 D) x' +12x? +48x+64arrow_forward2- 3" – 1. 3an + 2, for all nonnegative integers n. Prove that an %3D Let ao 1, and let an+! %3Darrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

