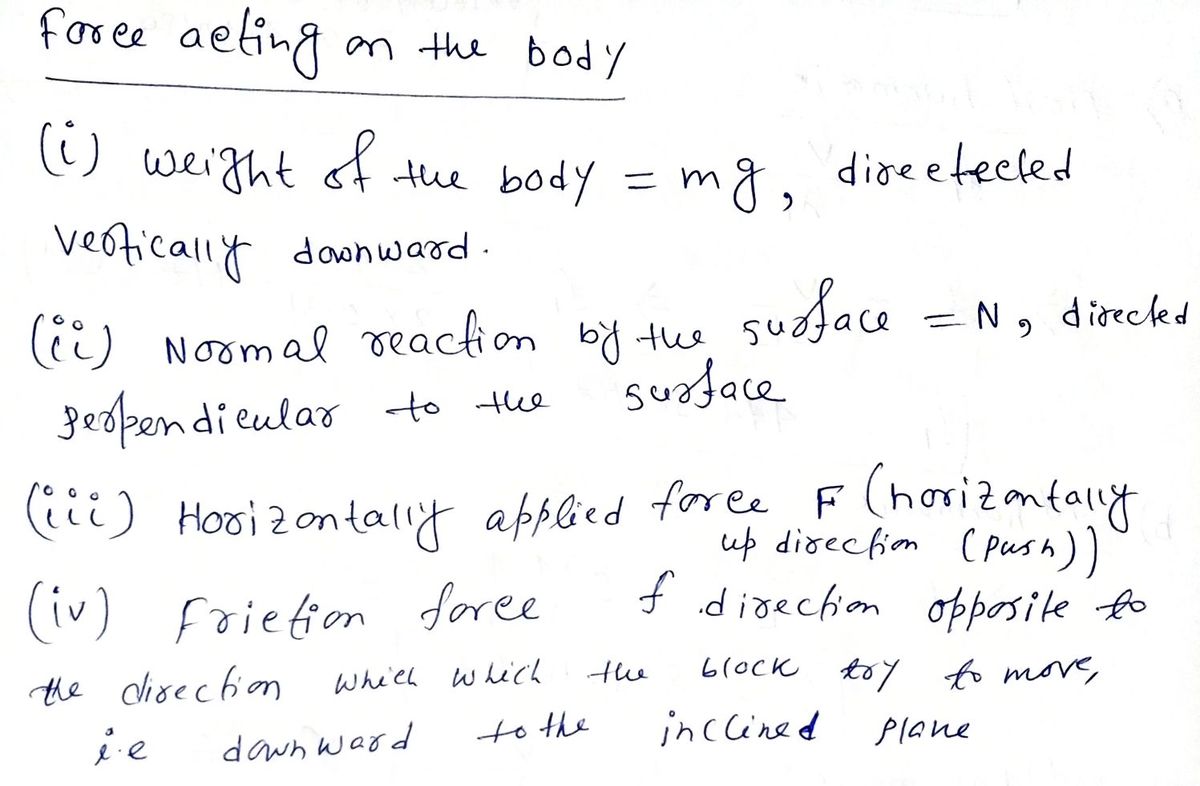
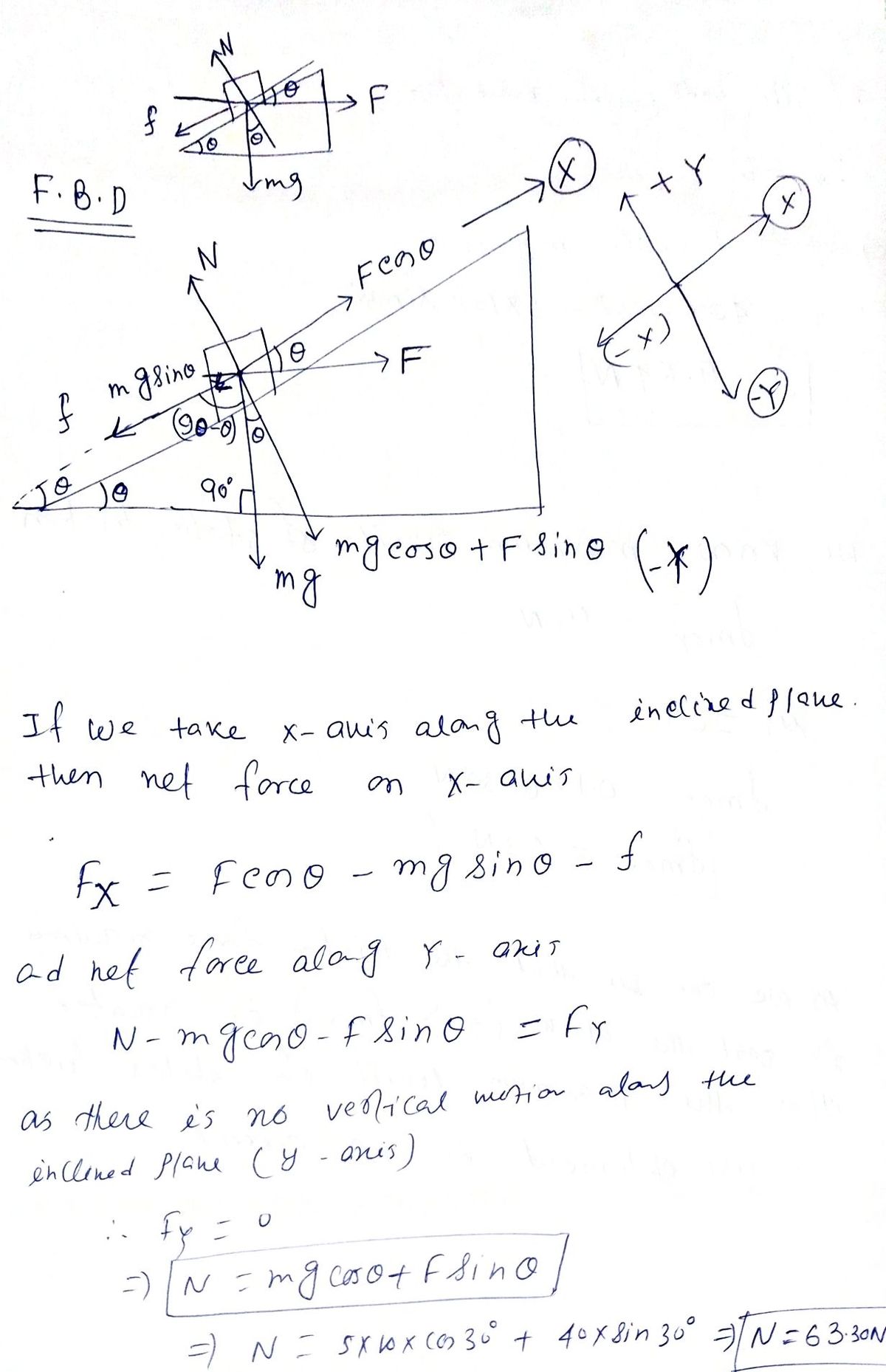
1. A 5 kg body resting on a 30° inclined plane is pushed by an applied force of 40N acting horizontally. The coefficient of friction is 0.1 a) Draw a free body diagram identifying all the forces acting on the body. F.B.D. Explanations
Q: Advanced Physics Question
A:
Q: With the installation of noise suppression materials, the noise level in an auditorium was reduced f...
A:
Q: A line of charge lying along the x-axis starts at x=+x0 and extends to positive infinity. It has a ...
A:
Q: An eagle has good eyes, enabling it to see small animals from afar. Assume the smallest animal an ea...
A: Given that, The aperture of the eye, D is 5 mm. The distance, d is 3 km. The wavelength of the light...
Q: A new design of Saturn 17 rocket with mass 1.37 x106 kg has a propulsion engine that can eject gases...
A: For the rocket motion, -udmdt=mdvdtIntegrating both side,v=ulnmtotmrocket
Q: A 21186Rn nucleus undergoes alpha decay according to the reaction 21186Rn → 20784Po + 42He. Determin...
A: (a) Write the expression to calculate the Q-value for the process. Q=M211Rn-M207Po-M4He C2 Here, M...
Q: A horizontal 1-m-square plate electric heater dissipates heat to the atmospheric air at 25oC. The po...
A: Given thatTsurface=85°CTatmosphere=25°CPower of electric heater,Q= 0.5 kwQ=500wattas we know Q=hA(Ts...
Q: The question number 23 & 25
A:
Q: 109. Monochromatic light passes through materials a, b, and c. Rank the materials according to index...
A: We have been given three different mediums a, b & c with corresponding refractive index μa, μb &...
Q: Stretching a spring farther before letting it bounce around will decrease the frequency of its oscil...
A:
Q: Suppose you were a linear polariser and a quater wave plate. How could you determine which was which...
A: Introduction: Linear Polarizers are Polarizers designed to linearly polarize incoming light. Passing...
Q: The gravitational acceleration on planet XR-17's surface is 16.0 m/s2 . What is the gravitational ...
A:
Q: (1)An energy-efficient lightbulb, taking in 23.0 W of power, can produce the same level of brightnes...
A:
Q: y (-5) cos(4x) What is the amplitude of the given function? What is the period of the given function...
A: Given function y = - 5 cos(4x) a)Therefore its amplitude is ymax=5unit b) we know that in one comple...
Q: Draw and write circuit and wave single phase half wave and full wave un-controlled rectifier. with a...
A: 1) Single Phase Half Wave Un-Controlled Rectifier The current and voltage at the output in this rect...
Q: A circuit consists of a 0.8 H inductor and a 3.5 ohm resistor. At t = 0 the current through the ind...
A:
Q: A dolphin uses echolocation underwater to "see" its surroundings. If the speed of sound in the ocean...
A: Assuming the sound is transmitted and recieved when dolphin is at rest. distance of the sea floor = ...
Q: A 2.91 kg particle has a velocity of (3.08 i - 3.95 j) m/s. (a) Find its x and y components of momen...
A: Given particle has mass M=2.91kg And its velocity is Therefore its momentum will be Here I've take...
Q: Considering the problem of a time independent one- dimensional particle in a box with a dimension fr...
A:
Q: A new design of Saturn 19 rocket with mass 1.17 x106 kg has a propulsion engine that can eject gases...
A: The variable mass of the rocket can be expressed through the following equation, Here, u is the spe...
Q: Suppose a person wishes to lift a large rock with a lever. If the rock has a mass of 250kg and is lo...
A:
Q: . A 0.40kg mass is attached to a spring with a spring constant of 160N/m so that the mass is allowed...
A: Given: Mass, m = 0.40 kg Spring constant, k= 160 N/m Mass is released from rest when the spring is c...
Q: The membrane that surrounds a certain type of living cell has a surface area of 5.45 10-9 m2 and a ...
A: A capacitor is a system of two conductors carrying equal and opposite charges placed at a very short...
Q: Please very soon
A:
Q: 110. The two mirrors shown meet at a right angle. The beam of light in the vertical plane P strikes ...
A:
Q: Could you please help?
A: Torque will be calculated about the pivot which knee joint in this case. Torque by a force is given ...
Q: Advanced Physics Question
A: (b) Consider a small element on the disk. The mass element be calculated as, σ=MA=MπR2M=σπR2Therefor...
Q: Given the equation vf = Vị + a*t , and multiple measurements of final velocity and time, which varia...
A: y=mx+c m is the slope c is the y intercept
Q: A bell in the middle of a carriage rings if its associated detector observes light pulses from the t...
A: Hello. Since your question has multiple sub-parts, we will solve the first three sub-parts for you. ...
Q: Describe the process of finding a capacitor’s current?
A: A capacitor is made up of two separate plate surface . When it is connected with a battery i.e. a po...
Q: 1.A 675-N Marine in basic training climbs a 10.5-m vertical rope at a constant speed in 9.25 s. What...
A:
Q: question E
A: Conservation of Energy For an isolated system, the initial total energy of the system is equal to th...
Q: The positron is the antiparticle of the electron, having exactly the same mass but opposite charge. ...
A: Given : Positron e+ and electron e- have the same rest mass. When a positron and an electron ...
Q: In preparing to shoot an arrow, an archer pulls a bow string back 0.40m by exerting a force of 230N....
A: Given information: The force exerted by the bow, F=230 N The compression in the bow, x=0.40 m
Q: I am given an equation (attatched image) and am told that G = newtons gravitational constant, m1 is ...
A: Given object 1 has mass m1 and object 2 has mass m2 which under go circular motion about first one i...
Q: \
A: Given an object is at a distance u=22.3 cm to the left of a lens having focal length f=+45.9 cm Thus...
Q: A rubber ball and a clay ball have equal mass and are dropped onto a digital scale. The rubber ball ...
A:
Q: A circuit consists of a 0.80 H inductor and a 3.5 ohm resistor. At t = 0 the current through the in...
A: The current through the inductor can be written as It=I0e-RtL ...
Q: The radio galaxy Cygnus A possesses a lobe of plasma that is detected by both radio and X-ray observ...
A: a) Collision frequency is, f=12πne2εomef=12π4×103 m-31.6×10-1928.854×10-12 C2/Nm29.1×10-31 kgf=567.4...
Q: Find the particle's horizontal position x(t) and velocity v(x) at any point in a fluid whose drag fo...
A: Part (a) Given: The drag force in the particle is Fdrag=kmv. The formula to calculate the net forc...
Q: 120 mm 60 mm 40 mm 80 mm x 60 mm For the plane area shown, determine the first moments with respect ...
A: We know Section area centroid (X-coordinate) Centroid (y-coordinate) Rectangle (Sides: a,b) ...
Q: The greatest value ever achieved for the speed of sound in air is about 1.0 x 10ʻm/s, and the highes...
A: Given: The speed in which the air travels =1.0×104 m/s The highest frequency produced=2.0×1010 Hz ...
Q: Suppose a person wishes to lift a large rock with a lever. If the rock has a mass of 250kg and is lo...
A:
Step by step
Solved in 3 steps with 3 images
