
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
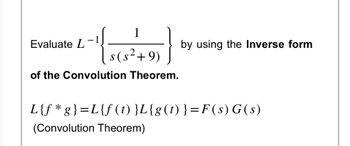
Transcribed Image Text:1
s(s² +9)
of the Convolution Theorem.
Evaluate L
by using the Inverse form
L{f*g}=L{f(t)}L{g(t)}=F(s) G(s)
(Convolution Theorem)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 2 images

Knowledge Booster
Similar questions
- What is this? Can you show with circles? For real function f A = f(f(A)) for en every why is A subset of the inverse? Can you show with for every real functim f: IR→IR, A = ² (F(A)) for every A CIR =) To show A is contained in f(f(A)), let a EA; we need to show that a Ef(f(A)). But this holds ift f(a) Ef (A), which holds since a EA and +(A) = {f(x)=xEA} The other inclusion i.e. f-1 (+(A)) S.A does not hold in general. So I have the following: Let fix→y be a function. Then of is one-to-one (injective) iff for every A CX, we have A = f(f(A)). pref= Assume first that of is injective, Let A CX. So we We already know that A ≤ f($(A)). -need to show that f-1 (F(A)) SA. only Let x Ef(f(A)). i.e. f(x) Ef(A) So 3 EA st. f(x) = f(a). Since of is one-to-one (injective), so f(x) = f(x) =)x=a SO REA = f(f(A)) ≤A Hence A = f(f(A)) Now, Conversely; Assume that A = f(f(A)) for all A EX. can you explain the proof (injective)arrow_forwardFind the rule of f of g of harrow_forwardpls show workarrow_forward
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

