
Elements Of Electromagnetics
7th Edition
ISBN: 9780190698614
Author: Sadiku, Matthew N. O.
Publisher: Oxford University Press
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
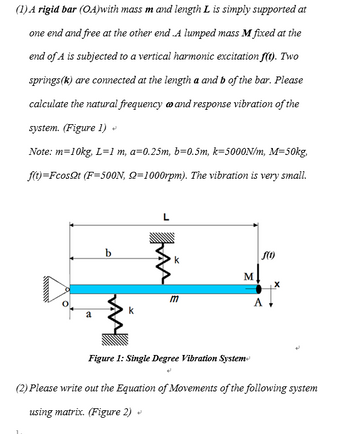
Transcribed Image Text:(1) A rigid bar (OA)with mass m and length L is simply supported at
one end and free at the other end .A lumped mass M fixed at the
end of A is subjected to a vertical harmonic excitation f(t). Two
springs (k) are connected at the length a and b of the bar. Please
calculate the natural frequency and response vibration of the
system. (Figure 1)
Note: m=10kg, L=1 m, a=0.25m, b=0.5m, k-5000N/m, M=50kg,
f(t)=Fcos.Qt (F=500N, Q=1000rpm). The vibration is very small.
a
b
k
L
k
+
m
M
Figure 1: Single Degree Vibration System+
f(1)
A
X
(2) Please write out the Equation of Movements of the following system
using matrix. (Figure 2)
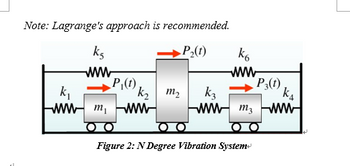
Transcribed Image Text:Note: Lagrange's approach is recommended.
P₂(t)
k₁
k5
www
P₁(t)
k₂ m₂
wwwm,ww
m₁
k3
k6
www
P3(t)
k₁
wwwm3wWw
Figure 2: N Degree Vibration System
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A 2 DOF mass-spring system shown below with n = 1, k = 8, and m initial conditions 2₁ (0) = 1, 2₂(0) = 0, ₁ (0) = 0, and ₂(0) = 1. (a) Find the natural frequencies and normalized modal matrix [X] for the system. T x₁ (1) (b) Using modal analysis and the Cartesian solution form, find the free vibration re- sponse of the system x₁(t) and x2(t). Ţ x2₂ (1) www. 00000 = m m₁ = 00000 k₁ = k 00000 k₂ = nk m₂ = m k3= k = 2 DOF mass-spring system. 2 with thearrow_forwardA cantilever is driven in flexural vibration by an electrical coil mounted at its free end and moving in the field of a magnet. The coil has been so designed that its mass is sufficiently small that it may be neglected but unfortunately this resulted in the moment of inertia of the coil being by no means negligible. For the beam, Young's modulus is E, the density is p its length is I and the second moment of inertia is I. The moment of inertia of the coil about an axis through its centre of gravity (which coincides with the end of the beam) perpendicular to the plane of vibration is J. Determine the frequency equation for this system.arrow_forwardFor the system below, the mass, m is attached to the end of a cantilever beam of length, l, elasticity, E, and moment of inertia, I. Derive the equation of motions and find the natural frequencies in two cases by lagrange energy method a) k1=k2=0 b) k1≠k2≠0arrow_forward
- t. Design the following application case The mechanical vibration system is shown in Figure (a). When a step input with 3N amplitude is subjected to this system, the movement rule of the mass m is shown in Figure (b). Evaluate the values of mass m, damping constant c and spring constant k. m (a) F-3N XX 1.0 x(1)(cm) 0 2 (b) M,-0.095 Ⓒarrow_forward(1) A rigid bar (OA)with mass m and length L is simply supported at one end and free at the other end .A lumped mass M fixed at the end of A is subjected to a vertical harmonic excitation f(t). Two springs(k) are connected at the length a and b of the bar. Please calculate the natural frequency w and response vibration of the system. (Figure 1) Note: m=10kg, L=1 m, a=0.25m, b=0.5m, k=5000N/m, M=50kg, f(t)=Fcos2t (F=500N, Q=1000rpm). The vibration is very small. a b k L MA m M Figure 1: Single Degree Vibration System f(t) A Xarrow_forwardQI Calculate the frequency of damped oscillation of the system shown in Figure () for the value m = 1.75 kg,c 350 N. s /m, k =7 x 107 N Am, a = 1.25 m and b = 2.5 m. If the mass pulled down 10' find the response of the system after cycle. I, mb² rigid linkarrow_forward
- Do not give answer in image and hand writingarrow_forwardFind the natural frequency (in Hertz) of the system illustrated in the following figure. Consider the length of the rigid bar as 2L and assume K1=4,659 N/m, K2=5,977 N/m, M=0.6 kg, L=0.5 m and g=9.81 m/s2.arrow_forward(b) A passenger car has a tyre spring rate of 18,900 N/m. The front suspension rate is 1,450 N/m and the rear is 1,050 N/m. Assume the front tyres are loaded to 10,000 N and rear tyres are at 7,900 N. (i) Solve the front suspension ride rate in N/m. (11) Evaluate the natural frequency at front suspension.arrow_forward
- Questionarrow_forwardConsider the damped mass-spring system for mass of 1.5 kg, spring constant 10 N/m, damping 3 kg/s and an oscillating force 4.7 cos(@t) Newtons. That is, 1.5x" + 3x' + 10x = 4.7 cos(@t). What positive angular frequency o leads to maximum practical resonance? W = help (numbers) What is the maximum displacement of the mass in the steady state solution when the we are at practical resonance: C(@) = helparrow_forwardThe 1 degree of freedom underdamped vibration system is subjected to external force as shown in the figure. (1) Finding a steady state response. (2) Induce the frequency at which the maximum displacement occurs in detail and (3) Find the maximum displacement at that time. (4) Plotting the frequency response function and explain its concept.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY

Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press

Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON

Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education

Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY

Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning

Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY