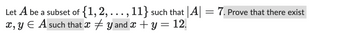
Let A be a subset of {1, 2,. 11} such that |A| = 7. Prove that there exist... 9x, y = A such that xy and x + y = 12.

Answered: Image /qna-images/answer/6f6a4b2c-37e2-4660-81f1-e86d355016b3.jpg

Answered: ...9 Let A be a subset of {1, 2, 11}…

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Similar questions

(b) Suppose that X,Y and Z are subsets of {1, 2, 3, . . . , 10} and |X| = |Y| = |Z| = 7.Deduce that X ∩ Y ∩ Z is non-empty. [Hint: Consider (X ∩ Y) ∪ Z.]
2.28 let xa be a translation of R Then (1) La is a continuous bijection from onto R (ii) The image of an open set under Da is an oper set. of (iii) let & be an open set. The component interval +a are exactly The images of the component intervals of the set & under translation a the goal of this section is to establish the following result.
Let S = {x | 6x – 1 E Q} and T be the - set of all odd integers. Prove that S and T have the same cardinality (no need to prove bijectivity of functions). Do not use the Schroder-Bernstein Theorem.
1. The following subsets A, B, and C of RX R are relations on R. Sketch the set. B = {(x, y): |x|+|y| ≤ 2} (i)
Let R be the relation defined on P({1,., 100}) by ARB if and only if |A n B| is even. Is R reflexive? Is R symmetric? Is R anti-symmetric? Is R transitive?
Assume R1 and R2 are reflective relations on a set A. Prove or disprove each of the following statements. (a) R1 U R2 is reflexive. (b) Rị N R2 is reflexive.
(a). Find a set S and a bijection between S and a proper subset of S. (b). What condition on S guarantees that S cannot be in bijection with a proper subset of itself?
Let R3 be the relation on Z+ such that xR3y if and only if 2x – 3y > 0. (Recall that Z+ = {1,2,3, 4, ...}, the positive integers.) 14) Prove or Disprove: R3 is anti-symmetric.
A. Let R be a relation on set A. A={x : x ∊ J and 0 ≤ x ≤ 12}, and R={(a,b) : a - b is multiple of 4 } . Represent this relation by a matrix. B. Using induction, prove that n2 + n is divisible by 2, where n ∊ N
Discrete Math
Let x Ry be defined as follows: Ry means that x > y, where x, y are integers. Select the true statements OR is none of these OR is reflexive R is transitive R is symmetric
Exercise 3.4.6. Let A be a non-empty family of sets and let B be a set. (1) Prove that (U X∈A X)−B = U X∈A(X −B). (2) Prove that (∩ X∈A X)−B = ∩ X∈A(X −B).

Recommended textbooks for you

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Advanced Engineering Mathematics

Advanced Math

ISBN:

9780470458365

Author:

Erwin Kreyszig

Publisher:

Wiley, John & Sons, Incorporated

Numerical Methods for Engineers

Advanced Math

ISBN:

9780073397924

Author:

Steven C. Chapra Dr., Raymond P. Canale

Publisher:

McGraw-Hill Education

Introductory Mathematics for Engineering Applicat…

Advanced Math

ISBN:

9781118141809

Author:

Nathan Klingbeil

Publisher:

WILEY

Mathematics For Machine Technology

Advanced Math

ISBN:

9781337798310

Author:

Peterson, John.

Publisher:

Cengage Learning,

Basic Technical Mathematics

Advanced Math

ISBN:

9780134437705

Author:

Washington

Publisher:

PEARSON

Topology

Advanced Math

ISBN:

9780134689517

Author:

Munkres, James R.

Publisher:

Pearson,

SEE MORE TEXTBOOKS

...9 Let A be a subset of {1, 2, 11} such that |A| = 7. Prove that there exist ï, y ¤ A such that ï ‡ y and x + y = 12.