Algebra & Trigonometry with Analytic Geometry
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN: 9781133382119
Author: Swokowski
Publisher: Cengage
Bartleby Related Questions Icon

Related questions

Q: Product rule combinatorics. Please correctly and handwritten.answers are also attached
A:
Q: Suppose V1, V2, V3 is an orthogonal set of vectors in R5. Let w be a vector in Span(V1, V2, V3) such…
A: Step 1:Step 2:Step 3:
Q: Consider the cyclic group {Z, o}, where o is + i.e. addition of integers. Show that 2303-2 is a…
A:
Q: Instructions to follow: * Give original work *Support your work with examples and graphs where…
A:
Q: 1. Prove whether or not the following are linear transformations. Make sure to provide a specific…
A: Step 1:A transformation T:V→W is linear if it satisfies the following properties for all functions…
Q: Instructions to follow: * Give original work *Support your work with examples and graphs where…
A:
Q: Calculate
A: We have a sports team selection committee with the following voting weights:Team Captain (P1): 5…
Q: Prove the following statements using direct, contrapositive, or proof by-contradiction methods. a.…
A: abcde
Q: Instructions to follow: * Give original work "Support your work with examples and graphs where…
A:
Q: please full solution thank youu
A: More explain
Q: Instructions to follow: * Give original work *Support your work with examples and graphs where…
A:
Q: What are dilated convolutions, and where are they typically used? Convolution Neural Network (CNN)…
A: Approach to solving the question: Detailed explanation: 1. What are Dilated Convolutions?In…
Q: Pls help on all asked questions. Pls show all work and steps. Pls circle the final answer.
A:
Q: Real estate tax $2,010.88 was due December 14, 2022. Lorna lost her job and could not pay her tax…
A: The given values are:Principal amount (P) = $2,010.88Interest rate (r) = 6 1/2% = 0.065 (in decimal…
Q: 6. Find the circulation and outward flux of the velocity field = sin yi + cos yj over the square cut…
A: Step 1: Step 2: Step 3:
Q: Instructions to follow: Give original work *Support your work with examples and graphs where…
A:
Q: Question 9: Spectral Theory of Weighted Shift Operators and Measure- Theoretic Dynamics Problem…
A: References with exact page numbers1. "Linear Operators: Part II: Spectral Theory" by Nelson Dunford…
Q: Instructions to follow: * Give original work Copy paste from chatgpt will get downvote *Support your…
A:
Q: A AT Reference book: KREYSZIG Introductory Functional Analysis with Applications Complement of ses A…
A: 1. Weak Topology Characterization  2. Banach-Alaoglu Theorem  3. Reflexivity Criterion
Q: Please prove Corollary 5.3.4
A:
Q: (1) Determine the reactions at pins A and C of the loaded two-bar frame. Ans: A = C = 80.4 lb Note:…
A: all the forces are in lband moment are in lb=ftplease upvote
Q: QUESTION 14 For the equation y" +6y' +5y = 7sin(x). write the form the you assume for the particular…
A:
Q: Statement: Prove the Bounded Gaps Conjecture, which states that there are infinitely many pairs of…
A: Step 1: Step 2: Step 3: Step 4: Step 5:
Q: Don't use chat gpt It Chatgpt means downvote
A:
Q: Prove the following statements with either induction, strong induction or proof by smallest…
A:
Q: Don't use chat gpt It Chatgpt means downvote
A: To prove Lemma: We need to show that the given statements for any subset A of a universal set U: 1.…
Q: Let the vertices of a rhombus be labeled by numerals 1, 2,3, 4. Write the elements of S that…
A: Step 1: Understand the Symmetries of the RhombusA rhombus has specific symmetries that keep its…
Q: Pls help on all asked questions. Pls show all work and steps. Pls circle the final answer.
A:
Q: Instruction: Do not use AI. : Do not just give outline, Give complete solution with visualizations.…
A:
Q: Instructions: 1. Give geometric interpretation and graphs where required. 2. Give your original…
A: Solution to Problem 5: Hyperbolic Surfaces and Graph Embeddings 1. Hyperbolic Surface EmbeddingThis…
Q: Instruction: Do not use AI. : Do not just give outline, Give complete solution with visualizations.…
A:
Q: Instruction: Do not use AI. : Do not just give outline, Give complete solution with visualizations.…
A:
Q: Instructions to follow: * Give original work "Support your work with examples and graphs where…
A:
Q: How many ways are there to arrange 10 identical apples and 5 different oranges in a row so that no…
A: Solve this question step by step with full calculations.
Q: 1 Use Euler’s method with step size h = 0.1 to approximate the solution ofthe IVP:y′ = y − t^2 + 1,…
A: Step 1: Step 2: Step 3: Step 4:
Q: full handritten solution please
A: Solve this question step by step with full calculations.
Q: Reported solution Export Ste Answer question 10 from chapter 5 Please follow this book to answer the…
A:
Q: Instructions to follow: * Give original work *Support your work with examples and graphs where…
A:
Q: Use the inner product (§, 9) = for f(x)9(x) dx in the vector space P₂ of polynomials of degree at…
A:
Q: Please help on all asked questions. Please show all work and steps. Also, please circle the final…
A:
Q: Instructions to follow: * Give original work "Support your work with examples and graphs where…
A:
Q: 39. 1. A set of 10 computers, 3 of which are defective, is sampled for inspection. Three computers…
A: Detailed explanation: Problem 1: Sampling ComputersWe are given a set of 10 computers, 3 of which…
Q: Kernel of Linear Transformation Consider the linear mapping F : Rª → Rª F(x, y, z, t) = (x −y+z+t,…
A: Given:The linear transformation  F:R4→R4 is defined by:F(x,y,z,t)=(x−y+z+t,2x−2y+3z+4t,3x−3y+4z+5t)…
Q: DISCRETE MATH
A: Step 1: Step 2: Step 3: Step 4:
Q: By considering u*u* N * N, prove that 00 Σ01(d) 01 (1) = Σ doo(d) oo (7) · d\n d\n
A: To prove the equation given in the image:∑d∣nσ1(d)σ1(n/d) = ∑d∣ndσ0(d)σ0(n/d), we'll use properties…
Q: Suppose a spring with spring constant 2 N/m is horizontal and has one end attached to a wall and the…
A: Introduction:We know the frequency of SHM is given as:f=2π1​mk​​ormk​=(2πf)2orm=(2πf)2k​…
Q: Instructions to follow: * Give original work "Support your work with examples and graphs where…
A: PART 1Semigroups of Operators on a Banach SpaceIn functional analysis, semigroups of operators are…
Q: Let op: G₁ → G₂ be an isomorphism. Prove that (Z(G1)) = Z(G2) i.e. centers of two isomorphic…
A: We prove that φ(Z(G1)) = Z(G2).  Here, G1 and G2 are groups, and φ: G1 → G2 is an isomorphism.…
Q: 2 -4 -24 -4 -1 0 4 4 A matrix A=-6 -6 1 28 22 reduces to 0 142 -1 0 04 4 0004 Find a basis for Nul…
A: Step 1: Step 2: Step 3: Step 4:
Q: 12. In each case, find all values of x for which the given matrix does not have an inverse. Explain…
A: Ans 12 a:Ans 12 b:
Question

No Chatgpt please 

Need correct solutions 

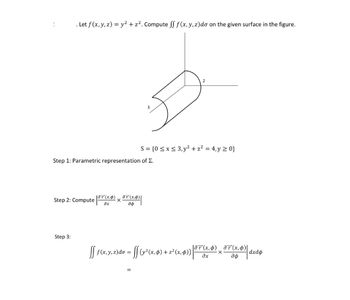
. Let f (x, y, z) = y² + z². Compute ff f(x, y, z) do on the given surface in the figure. 3 S = {0≤x≤3, y² + z² = 4, y ≥ 0} Step 1: Parametric representation of Σ. Step 2: Compute (x дт(х,ф) × (x) дф Step 3: ſſ ƒ (x, y, z)do = ſſ (y² (x, $) + z² (x,¢)) |ǝF‍(x,¢)¸ðF(x, 0)| dxdo дх дф
expand button
Transcribed Image Text:. Let f (x, y, z) = y² + z². Compute ff f(x, y, z) do on the given surface in the figure. 3 S = {0≤x≤3, y² + z² = 4, y ≥ 0} Step 1: Parametric representation of Σ. Step 2: Compute (x дт(х,ф) × (x) дф Step 3: ſſ ƒ (x, y, z)do = ſſ (y² (x, $) + z² (x,¢)) |ǝF‍(x,¢)¸ðF(x, 0)| dxdo дх дф
expand button
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
bartleby

Step by stepSolved in 2 steps

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Similar questions
Recommended textbooks for you
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Text book image
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Text book image
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Text book image
Elementary Linear Algebra (MindTap Course List)
Algebra
ISBN:9781305658004
Author:Ron Larson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS