What Are the Legs of a Triangle?
Answer – The legs of a triangle are its sides. Some triangles like the right triangle and isosceles triangle have two sides called legs with the third side having a different name.
Explanation:
The legs of a triangle are another way of referring to its sides. In the case of a right triangle though, the legs refer to the shorter sides that converge to form the right angle. The side opposite the right angle, which is the longest side, is called the hypotenuse.
Below is a right triangle, with its legs and hypotenuse marked:
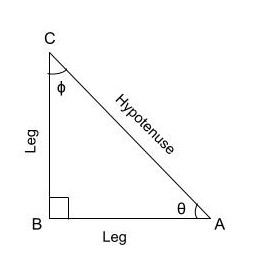
The legs of a right triangle can also be further differentiated as the opposite leg and the adjacent leg. Depending on which angle of the right triangle is being considered, the sides referenced as the opposite and adjacent legs will vary.
When considering the angle θ in the above figure, the side opposite it (BC) is the opposite leg and the side adjacent to it (AB) is the adjacent leg. On the other hand, when the angle being considered is ɸ, the opposite leg is AB while the adjacent leg is BC.
If the triangle being considered is an isosceles triangle, the sides of equal length are its legs while the side opposite the vertex angle is called the base.
Popular Questions
SHOW MORE TEXTBOOK SOLUTIONS+
- Find the missing dimensions of the following figures. Round to the nearest hundredth if necessary.
- If a triangle that has an area of 110 square feet has a base that is two feet less than twice the height, what is the length of its base and height?
- In the following exercises, solve using any method. Round your answers to the nearest tenth, if needed.. 202. The base of a triangle is six more th...
- In the following exercises, solve using any method. Round your answers to the nearest tenth, if needed.. 201. A triangle with area 45 square inches...
- Find the area of each figure. All hexagons are regular. Round to the nearest whole number unless otherwise directed in parentheses.
- In the following exercises, solve using triangle properties. 222. What is the height of a triangle with area 893 square inches and base 38 inches?
- In the following exercises, solve the given problem. 190. The sum of the measures of the angles of a triangle is 180. The sum of the measures of th...
- In the following exercises, solve. Approximate to the nearest tenth, if necessary. 405. Sergio needs to attach a wire to hold the antenna to the ro...
- Find the area of a triangle given a = 4.38 ft, b = 3.79 ft, and c = 5.22 ft.
- A triangular tent door has area 15 square feet. The height is five feet. What is the base?
- What is the area of the triangle shown in the following figure?
- Use Properties of Triangles In the following exercises, solve using the properties of triangles. One side of a triangle is three times the smallest...
-
Solve each triangle. See Examples 2 and 3.
35. a = 3.0 ft, b = 5.0 ft, c = 6.0 ft
- In the following exercises, solve using a geometry formula. 197. What is the base of a triangle with area 207 square inches and height 18 inches?
- In the following exercises, solve using triangle properties. 394. What is the height of a triangle with area 67.5 square meters and base 9 meters?
- For the following exercises, use Herons formula to find the area of the triangle. Round to the nearest hundredth. 29. a=12m,b=13m,c=14m .
- Solve each triangle. See Examples 2 and 3. a = 28 ft, b = 47 ft, c = 58 ft
- Use vectors to prove that the line joining the midpoints of two sides of a triangle is parallel to the third side and half its length.
- In the following exercises, solve using triangle properties. 392. The playground at a shopping mall is a triangle with perimeter 48 feet. The lengt...
- TRY IT ::9.46 Use the Pythagorean Theorem to find the length of the leg.
- 3. If 3 and 4 are the legs of a right triangle, the hypotenuse is _____ .
- Is there a triangle that has one side of length 4 inches, one side of length 2 inches, and one side of length 1 inch? Explain.
- Use the Properties of Triangles In the following exercises, solve using the properties of triangles. 178. What is the height of a triangle with an ...
- In the following exercises, solve. 358. The length of one leg of a right triangle is three more than the other leg. If the hypotenuse is 15, find t...
- Find the base and height of a triangle whose base is four inches more than six times height and hasan area of 456 square inches.
- In the following exercises, solve using any method. Round your answers to the nearest tenth, if needed.. 203. The area of a triangular flower bed i...
- In the following exercises, solve using any method. Round your answers to the nearest tenth, if needed.. 204. A triangular banner for the basketbal...
- The lengths of the legs of a right triangle are consecutive even integers. The numerical value of the area is three times that of the longer leg. F...
- The area of a triangular painting is 126 square inches. The base is 18 inches. What is the height?
- In the following exercises, solve the given problem. 191. The sum of the measures of the angles of a triangle is 180. The sum of the measures of th...
- The area of a triangle is 80x5y3. The height of the triangle is x4y. What is the length of the base of the triangle?
- In the following exercises, solve using triangle properties. 221. What is the base of a triangle with area 207 square inches and height 18 inches?
- What type of triangle has two equal sides?
- Find the unknown area, base, or height for each of the triangles 7 through 18. Where necessary, round the answers to 1 decimal place.
- Use Properties of Triangles In the following exercises, solve using the properties of triangles. The perimeter of a triangle is 59 feet. One side o...
- Find the area A of a triangle with height 4 inches and base 2 inches.
- In the following exercises, solve using a geometry formula. 198. What is the height of a triangle with area 893 square inches and base 38 inches?
- The height of a triangle is 4x inches and the base is (5x+1) inches. The area of the triangle is 500 square inches. What are the dimensions of the ...
- Use Herons formula to find the area of a triangle with sides of lengths a = 29.7 ft, b = 42.3 ft, and c = 38.4 ft.
- In Exercises 1-14, use the formulas developed in this section to find the area of each figure.
- Translate to an algebraic expression. lf the height of a triangle is doubled, is its area also doubled? Why or why not?
- TRY IT ::9.45 Use the Pythagorean Theorem to find the length of the leg.
- Use Properties of Triangles In the following exercises, solve using the properties of triangles. A tile in the shape of an isosceles triangle has a...
- Geometry A right triangle has a hypotenuse of length 8 inches. If one angle is 35 , find the length of each leg.
- For the following exercises, find the measurement of angle A.
- Solve each problem. Round to the nearest tenth, if necessary. Use 3.14 for pi. A triangle has base 7m and area 17.5m2. What is the height?
