Consider the following dynamic IS-LM model. C(t) = 20+ 0.8% a(t-1) Ya(t) = Y(t) - Tx(t) Tx(t) 5 +0.25Y(t) (iv) = I(t) = 20 – 2r(t) G = 50 (i) What is the equilibrium level of Y and r? (ii) Show that dynamic IS and LM equations are the recursive equations for Y(+1) and r(t+1). That is, this policy change? E (t) = C(t) + 1(t) + G AY(t + 1) = 0.05 [E(t) - Y(t)] Ma(t) = 10+ 0.25Y(t) 0.5r(t) M,(t) = 55 Ar(t+1)=0.8 [Ma(t)- M, (t)] Y(t + 1) = 86a +(1- a)Y(t) + 0.6aY(t-1)-2ar(t) r(t + 1) = -45ß +0.25BY(t) + (1 - 0.5ß) r(t) where a = 0.05 is the speed of good market adjustment and ß = 0.8 is the speed of money market adjustment. [Hint: Substitute all the relationships in each of the adjustment equations in turn. The algebra can be somewhat tedious but not intellectually difficult.] Suppose the Reserve Bank reduces the money supply (Ms) to 53.6 in period 2, given Y and r are at their equilibrium values in periods 0 and 1. (iii) calculate the new equilibrium output and interest rate. [Hint: The LM curve shifts left so you need to re-calculate the dynamic LM curve] plot the trajectory of the economy resulting from

Answered: Consider the following dynamic IS-LM model. C(t) = 20+ 0.8% a(t-1) Ya(t) = Y(t) - Tx(t) Tx(t) 5 +0.25Y(t) (iv) = I(t) = 20 – 2r(t) G = 50 (i) What is the…

ENGR.ECONOMIC ANALYSIS

14th Edition

ISBN: 9780190931919

Author: NEWNAN

Publisher: Oxford University Press

See similar textbooks

Related questions

Q: YEAR PRODUCT 1988 1989 1990 1991 $6.00 $8.00 price: $2.00 quantity: price: $1.00 product $4.00 2,000…

A: The Consumer Price Index (CPI) is a measure of the average change over time in the prices paid by…

Q: If the population increases by 2.5% per year, what percentage does it change in 5 years?

A: Given,Population increases by per year.

Q: 1. Compute the linear cost function, relating total overhead costs to physician contact hours, using…

A: “Since you have posted multiple questions, we will provide the solution only to the first question…

Q: Can you show a diagram with numbers (detailed)?

A: Any type of intervention by the government interferes with the free-market mechanism and generates a…

Q: Carson used $800 000 of their savings to open a coffee shop. The savings account paid 2% interest.…

A: We know that the economic profit is the difference between the total sales revenue of output and the…

Q: References Mailings Review View Help In a one-shot game, if you advertise and your rival advertises,…

A: a) From the given information ,we get the payoffs of the game as shown below: Your rival Your…

Q: what will happen if two assets are earning the same exoected return, but one is risker than the…

A: You are presented with two assets in your investment portfolio.Both of these assets are expected to…

Q: Tony decided to visit his parent in San Diego during the long weekend. He has two options. Either he…

A: Opportunity cost is the value of the following best alternative that must be given up in order to…

Q: QUESTION 1 Given the Budget of $1000 P of X = 10 10 Q of X 0 10 20 30 40 50 60 TU of X (10s) 0 100…

A: Budget constraint represents all the possible combinations of the goods or services that a consumer…

Q: Just to make sure this answer is 100% accurate and is the correct answer to the question, this is am…

A: The Solow Growth Model, an exogenous model of economic growth, examines changes in an economy's…

Q: Question 7 (Hint: fill in the graph.) D: 5000-1.5Q S: 200+ 2.5Q In the world market for scooters,…

A: A country becomes an exporter when world price is greater than domestic equilibrium price.

Q: Question 6 85 Price 8 75- 7 6.5 6 55. Social Cast

A: Government intervention refers to the actions made by a government to influence or impact the…

Q: Use the following table to find percentage changes in the cost of living (COL) from 2020 to 2021:…

A: The cost of incorporating housing, food and transportation for the year between 2020 to 2022 is…

Q: SERNAME: student ASSWORD: Broward1

A: Introduction The percentage of the population living in poverty. The difference between the poverty…

Q: A B 1 Car Sales Report Month 2 3 Jan. - April 4 May-Aug. 5 Sept. - Dec. 6 TOTAL 7 MINIMUM C 214 355…

A: The data gives the sale figures for cars from 2016 to 2018. Each year is subdivided into three…

Q: 20+100+200000

A: 100+20+200000120+200000200120

Q: SPEED (Feet per second) 20 18 18 12 10 N 4 5 TIME (Seconds) The area of the shaded quadrilateral is…

A: The question asked us to find the area of the shaded region.

Q: The following information has been gathered on the costs and effectiveness of the two treatments, A…

A: Cost-benefit analysis is a decision-making tool that evaluates the potential costs and benefits of a…

Q: In the following list of action profiles, check all the ones that are NE.

A: Nash equilibrium is a concept in game theory that represents a stable state in a strategic…

Q: What are some causes of rising food prices and the challenges faced by rural farmers in Mt. Royal,…

A: Growing food costs have important ramifications since they affect the accessibility and…

Q: I need typing clear urjent no chatgpt use i will give 5 upvotes I need 3 answers

A: Thank you,Please rate my response.

Q: 2. A person considers the following four criteria for buying a car. The pairwise comparison matrix…

A: The weights are supposed to represent the relative significance of the different products and…

Q: PLS HELP ASAP ON BOTH

A: Participating preferred shares are a type of preferred stock that comes with the benefit of allowing…

Q: What is the MC of the 2nd unit? Select an answer and submit. For keyboajd navigation, use the…

A: MC = Change in TC/change in Q

Q: b. Task Machine 1 2 3 4 5 C. Processing time = Research CEAS

A: (a)Create the Cost MatrixThe given cost matrix (processing times) is: Machine 1Machine 2Machine…

Q: The total revenue generated is $400 and the output is 12 units Find the value of average revenue

A: The information being given is:- Total revenue = $400 Output = 12 units We have to calculate the…

Q: Suppose the imposition of a $2-unit tariff on X leads to a fall in the international price of X from…

A: An imposition of a unit tariff refers to the levying of a fixed amount of tax or duty on the import…

Question

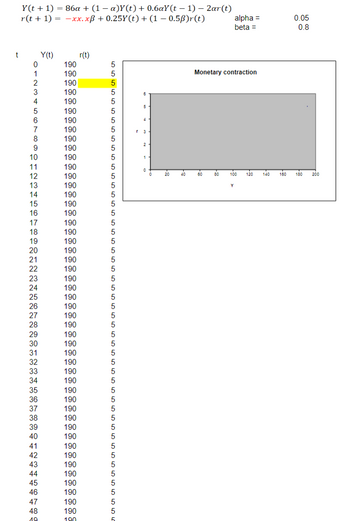
just need graph for (iv) please

t
Y(t + 1) = 86a + (1 - a)Y(t) + 0.6aY(t− 1) — 2ar(t)
r(t + 1) = -xx.xß +0.25Y(t) + (1 - 0.5p)r(t)
0
1
2
3
4
5
6
7
8
9
10
12345678 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 4142434456F48 49
11
47
Y(t)
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
190
r(t)
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
5
6
r3
1
0
20
40
Monetary contraction
60
80
alpha=
beta =
100
Y
120
140
160
0.05
0.8
180
200

Consider the following dynamic IS-LM model.
C(t) = 20 +0.8Y(t-1)
(i) What is the equilibrium level of Y and r?
(ii) Show that dynamic IS and LM equations are the recursive equations for Y(t+1) and
r(t+1). That is,
(iv)
Ya(t) = Y(t) - Tx(t)
Tx(t) = 5 +0.25Y(t)
I(t) = 20 2r(t)
G = 50
E (t) = C(t) + 1(t) + G
AY(t+1) = 0.05 [E(t) - Y(t)]
Ma(t) = 10+ 0.25Y(t)
0.5r(t)
M,(t) = 55
Ar(t+1)= 0.8[Ma(t) - Ms(t)]
Y(t+1) = 86a + (1 − a)Y(t) + 0.6aY(t-1)-2ar(t)
r(t + 1) = -45ß +0.25ßY(t) + (1 - 0.5B)r(t)
where a = 0.05 is the speed of good market adjustment and ß= 0.8 is the speed of
money market adjustment. [Hint: Substitute all the relationships in each of the
adjustment equations in turn. The algebra can be somewhat tedious but not
intellectually difficult.]
Suppose the Reserve Bank reduces the money supply (Ms) to 53.6 in period 2, given Y and r
are at their equilibrium values in periods 0 and 1.
(iii)
calculate the new equilibrium
output and interest rate. [Hint: The LM curve shifts left so you need to re-calculate the
dynamic LM curve]
this policy change?
plot the trajectory of the economy resulting from

Expert Solution

Step 1: Define IS LM model

The IS-LM model is a macroeconomic model that shows the relationship between interest rates and output in the economy. The model is divided into two parts: the IS curve and the LM curve.

The IS curve shows the combinations of interest rates and output that result in equilibrium in the goods and services market. The IS curve is downward-sloping because lower interest rates lead to higher investment and higher output.

The LM curve shows the combinations of interest rates and output that result in equilibrium in the money market. The LM curve is upward-sloping because higher interest rates lead to higher demand for money and lower output.

Step by stepSolved in 3 steps with 4 images

See solution

Check out a sample Q&A here

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, economics and related others by exploring similar questions and additional content below.