Question
It says (in blue highlighted) that I will be of constant motion if I and H poisson commute.
Please show that this is true in detail.
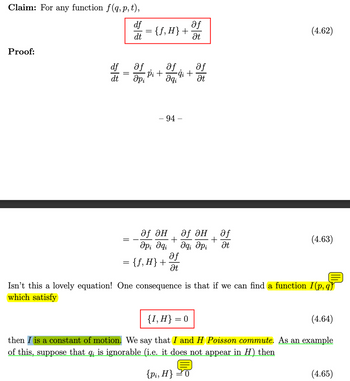
Transcribed Image Text:Claim: For any function f(q, p,t),
Proof:
df
af
{f, H} +
(4.62)
dt
Ət
df
dt
=
af
дрі
af
af
·Pi +
-ġi +
Əqi
Ət
- 94 -
дf дн
дf ән
=
+
მp; მq; да дрі
af
= {ƒ,H} +
Ət
+
55
af
(4.63)
Ət
Isn't this a lovely equation! One consequence is that if we can find a function I (p,q)
which satisfy
{I, H} = 0
(4.64)
then I is a constant of motion. We say that I and H Poisson commute. As an example
of this, suppose that q; is ignorable (i.e. it does not appear in H) then
{pi, H}
(4.65)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- This problem is designed to give you practice using the Dirac delta function. Eval- uate the following integrals. Show your reasoning.arrow_forwardConsider the special shape pictured in the diagram below. It is a cylinder, centered on the origin with its axis oriented along z, and it has been partially hollowed to leave two cone-shaped cavities at the top and bottom of the cylinder. The radius of the object is a, its height is 2a, and the solid part of the object (the shaded region that is visible in the rightmost panel of the illustration above, which shows a drawing of the cross-section of the object) has a uniform volume charge density of po. Assume that the object is spinning counter clockwise about its cylinder axis at an angular frequency of w. Which of the following operations is part of the calculation of the magnitude of the current density that is associated with the motion of the rotating object as a function of r (select all that apply)?arrow_forwardA particle of electric charge q and mass m is initially at rest in the presence of a uniform electric field E and a uniform magnetic field B, perpendicular to E (directions are not specified in this problem). Describe the subsequent motion of the particle. Namely, if we use a Cartesian laboratory frame of reference whose origin is the initial position of the particle, you must find the particle trajectory (x(t), y(t), z(t)).arrow_forward
- Brick with mass m that resting at the top of the inclined plane that has a height of 4.43 m and has an angle of theta = 15.2 degrees with respect to the horizontal. After begin released, is observed to be moving at v = 0.79 m/s a distance d after inclined plane. With coefficient of kinetic friction between brick and plane u_p = 0.1 and coefficient on horizontal surface is u_r = 0.2. Based in the image attached. What would the distance (meters) would be?arrow_forwardSketch how water curls down a sink, say, in clock-wise rotation. Draw the resulting vector of the curl-operator applied on this water flow.arrow_forwardFor each of the following vector fields F , decide whether it is conservative or not by computing curl F . Type in a potential function f (that is, ∇f=F). F(x,y)=(−3siny)i+(10y−3xcosy)jarrow_forward
arrow_back_ios
arrow_forward_ios